php-perl哈希算法实现(times33哈希算法)_PHP教程
APR_DECLARE_NONSTD(unsigned int) apr_hashfunc_default(const char *char_key,
apr_ssize_t *klen)
{
unsigned int hash = 0;
const unsigned char *key = (const unsigned char *)char_key;
const unsigned char *p;
apr_ssize_t i;
/*
* This is the popular `times 33' hash algorithm which is used by
* perl and also appears in Berkeley DB. This is one of the best
* known hash functions for strings because it is both computed
* very fast and distributes very well.
*
* The originator may be Dan Bernstein but the code in Berkeley DB
* cites Chris Torek as the source. The best citation I have found
* is "Chris Torek, Hash function for text in C, Usenet message
* in comp.lang.c , October, 1990." in Rich
* Salz's USENIX 1992 paper about INN which can be found at
* .
*
* The magic of number 33, i.e. why it works better than many other
* constants, prime or not, has never been adequately explained by
* anyone. So I try an explanation: if one experimentally tests all
* multipliers between 1 and 256 (as I did while writing a low-level
* data structure library some time ago) one detects that even
* numbers are not useable at all. The remaining 128 odd numbers
* (except for the number 1) work more or less all equally well.
* They all distribute in an acceptable way and this way fill a hash
* table with an average percent of approx. 86%.
*
* If one compares the chi^2 values of the variants (see
* Bob Jenkins ``Hashing Frequently Asked Questions'' at
* http://burtleburtle.net/bob/hash/hashfaq.html for a description
* of chi^2), the number 33 not even has the best value. But the
* number 33 and a few other equally good numbers like 17, 31, 63,
* 127 and 129 have nevertheless a great advantage to the remaining
* numbers in the large set of possible multipliers: their multiply
* operation can be replaced by a faster operation based on just one
* shift plus either a single addition or subtraction operation. And
* because a hash function has to both distribute good _and_ has to
* be very fast to compute, those few numbers should be preferred.
*
* -- Ralf S. Engelschall
*/
if (*klen == APR_HASH_KEY_STRING) {
for (p = key; *p; p++) {
hash = hash * 33 + *p;
}
*klen = p - key;
}
else {
for (p = key, i = *klen; i; i--, p++) {
hash = hash * 33 + *p;
}
}
return hash;
}
对函数注释部分的翻译: 这是很出名的times33哈希算法,此算法被perl语言采用并在Berkeley DB中出现.它是已知的最好的哈希算法之一,在处理以字符串为键值的哈希时,有着极快的计算效率和很好哈希分布.最早提出这个算法的是Dan Bernstein,但是源代码确实由Clris Torek在Berkeley DB出实作的.我找到的最确切的引文中这样说”Chris Torek,C语言文本哈希函数,Usenet消息 in comp.lang.c ,1990年十月.”在Rich Salz于1992年在USENIX报上发表的讨论INN的文章中提到.这篇文章可以在上找到. 33这个奇妙的数字,为什么它能够比其他数值效果更好呢?无论重要与否,却从来没有人能够充分说明其中的原因.因此在这里,我来试着解释一下.如果某人试着测试1到256之间的每个数字(就像我前段时间写的一个底层数据结构库那样),他会发现,没有哪一个数字的表现是特别突出的.其中的128个奇数(1除外)的表现都差不多,都能够达到一个能接受的哈希分布,平均分布率大概是86%. 如果比较这128个奇数中的方差值(gibbon:统计术语,表示随机变量与它的数学期望之间的平均偏离程度)的话(见Bob Jenkins的http://burtleburtle.net/bob/hash/hashfaq.html,中对平方差的描述),数字33并不是表现最好的一个.(gibbon:这里按照我的理解,照常理,应该是方差越小稳定,但是由于这里不清楚作者方差的计算公式,以及在哈希离散表,是不是离散度越大越好,所以不得而知这里的表现好是指方差值大还是指方差值小),但是数字33以及其他一些同样好的数字比如 17,31,63,127和129对于其他剩下的数字,在面对大量的哈希运算时,仍然有一个大大的优势,就是这些数字能够将乘法用位运算配合加减法来替换,这样的运算速度会提高.毕竟一个好的哈希算法要求既有好的分布,也要有高的计算速度,能同时达到这两点的数字很少.

Heiße KI -Werkzeuge

Undresser.AI Undress
KI-gestützte App zum Erstellen realistischer Aktfotos

AI Clothes Remover
Online-KI-Tool zum Entfernen von Kleidung aus Fotos.

Undress AI Tool
Ausziehbilder kostenlos

Clothoff.io
KI-Kleiderentferner

AI Hentai Generator
Erstellen Sie kostenlos Ai Hentai.

Heißer Artikel

Heiße Werkzeuge

Notepad++7.3.1
Einfach zu bedienender und kostenloser Code-Editor

SublimeText3 chinesische Version
Chinesische Version, sehr einfach zu bedienen

Senden Sie Studio 13.0.1
Leistungsstarke integrierte PHP-Entwicklungsumgebung

Dreamweaver CS6
Visuelle Webentwicklungstools

SublimeText3 Mac-Version
Codebearbeitungssoftware auf Gottesniveau (SublimeText3)

Heiße Themen
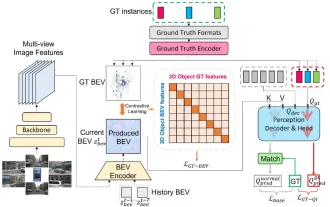 CLIP-BEVFormer: Überwacht explizit die BEVFormer-Struktur, um die Leistung der Long-Tail-Erkennung zu verbessern
Mar 26, 2024 pm 12:41 PM
CLIP-BEVFormer: Überwacht explizit die BEVFormer-Struktur, um die Leistung der Long-Tail-Erkennung zu verbessern
Mar 26, 2024 pm 12:41 PM
Oben geschrieben und das persönliche Verständnis des Autors: Derzeit spielt das Wahrnehmungsmodul im gesamten autonomen Fahrsystem eine entscheidende Rolle Das Steuermodul im autonomen Fahrsystem trifft zeitnahe und korrekte Urteile und Verhaltensentscheidungen. Derzeit sind Autos mit autonomen Fahrfunktionen in der Regel mit einer Vielzahl von Dateninformationssensoren ausgestattet, darunter Rundumsichtkamerasensoren, Lidar-Sensoren und Millimeterwellenradarsensoren, um Informationen in verschiedenen Modalitäten zu sammeln und so genaue Wahrnehmungsaufgaben zu erfüllen. Der auf reinem Sehen basierende BEV-Wahrnehmungsalgorithmus wird von der Industrie aufgrund seiner geringen Hardwarekosten und einfachen Bereitstellung bevorzugt, und seine Ausgabeergebnisse können problemlos auf verschiedene nachgelagerte Aufgaben angewendet werden.
 Wie implementiert man die doppelte WeChat-Anmeldung auf Huawei-Mobiltelefonen?
Mar 24, 2024 am 11:27 AM
Wie implementiert man die doppelte WeChat-Anmeldung auf Huawei-Mobiltelefonen?
Mar 24, 2024 am 11:27 AM
Wie implementiert man die doppelte WeChat-Anmeldung auf Huawei-Mobiltelefonen? Mit dem Aufkommen der sozialen Medien ist WeChat zu einem unverzichtbaren Kommunikationsmittel im täglichen Leben der Menschen geworden. Viele Menschen können jedoch auf ein Problem stoßen: Sie können sich gleichzeitig auf demselben Mobiltelefon bei mehreren WeChat-Konten anmelden. Für Huawei-Mobiltelefonbenutzer ist es nicht schwierig, eine doppelte WeChat-Anmeldung zu erreichen. In diesem Artikel wird erläutert, wie eine doppelte WeChat-Anmeldung auf Huawei-Mobiltelefonen erreicht wird. Erstens bietet das EMUI-System, das mit Huawei-Mobiltelefonen geliefert wird, eine sehr praktische Funktion – das doppelte Öffnen von Anwendungen. Durch die doppelte Öffnungsfunktion der Anwendung können Benutzer gleichzeitig
 Implementierung von Algorithmen für maschinelles Lernen in C++: Häufige Herausforderungen und Lösungen
Jun 03, 2024 pm 01:25 PM
Implementierung von Algorithmen für maschinelles Lernen in C++: Häufige Herausforderungen und Lösungen
Jun 03, 2024 pm 01:25 PM
Zu den häufigsten Herausforderungen, mit denen Algorithmen für maschinelles Lernen in C++ konfrontiert sind, gehören Speicherverwaltung, Multithreading, Leistungsoptimierung und Wartbarkeit. Zu den Lösungen gehören die Verwendung intelligenter Zeiger, moderner Threading-Bibliotheken, SIMD-Anweisungen und Bibliotheken von Drittanbietern sowie die Einhaltung von Codierungsstilrichtlinien und die Verwendung von Automatisierungstools. Praktische Fälle zeigen, wie man die Eigen-Bibliothek nutzt, um lineare Regressionsalgorithmen zu implementieren, den Speicher effektiv zu verwalten und leistungsstarke Matrixoperationen zu nutzen.
 So implementieren Sie die WeChat-Klonfunktion auf Huawei-Mobiltelefonen
Mar 24, 2024 pm 06:03 PM
So implementieren Sie die WeChat-Klonfunktion auf Huawei-Mobiltelefonen
Mar 24, 2024 pm 06:03 PM
So implementieren Sie die WeChat-Klonfunktion auf Huawei-Mobiltelefonen Mit der Popularität sozialer Software und der zunehmenden Bedeutung von Datenschutz und Sicherheit rückt die WeChat-Klonfunktion allmählich in den Mittelpunkt der Aufmerksamkeit der Menschen. Die WeChat-Klonfunktion kann Benutzern helfen, sich gleichzeitig bei mehreren WeChat-Konten auf demselben Mobiltelefon anzumelden, was die Verwaltung und Nutzung erleichtert. Es ist nicht schwierig, die WeChat-Klonfunktion auf Huawei-Mobiltelefonen zu implementieren. Sie müssen lediglich die folgenden Schritte ausführen. Schritt 1: Stellen Sie sicher, dass die Version Ihres Mobiltelefonsystems und die WeChat-Version den Anforderungen entsprechen. Stellen Sie zunächst sicher, dass die Version Ihres Huawei-Mobiltelefonsystems sowie die WeChat-App auf die neueste Version aktualisiert wurden.
 Entdecken Sie die zugrunde liegenden Prinzipien und die Algorithmusauswahl der C++-Sortierfunktion
Apr 02, 2024 pm 05:36 PM
Entdecken Sie die zugrunde liegenden Prinzipien und die Algorithmusauswahl der C++-Sortierfunktion
Apr 02, 2024 pm 05:36 PM
Die unterste Ebene der C++-Sortierfunktion verwendet die Zusammenführungssortierung, ihre Komplexität beträgt O(nlogn) und bietet verschiedene Auswahlmöglichkeiten für Sortieralgorithmen, einschließlich schneller Sortierung, Heap-Sortierung und stabiler Sortierung.
 PHP-Programmierhandbuch: Methoden zur Implementierung der Fibonacci-Folge
Mar 20, 2024 pm 04:54 PM
PHP-Programmierhandbuch: Methoden zur Implementierung der Fibonacci-Folge
Mar 20, 2024 pm 04:54 PM
Die Programmiersprache PHP ist ein leistungsstarkes Werkzeug für die Webentwicklung, das eine Vielzahl unterschiedlicher Programmierlogiken und Algorithmen unterstützen kann. Unter diesen ist die Implementierung der Fibonacci-Folge ein häufiges und klassisches Programmierproblem. In diesem Artikel stellen wir vor, wie Sie die Fibonacci-Folge mit der Programmiersprache PHP implementieren, und fügen spezifische Codebeispiele bei. Die Fibonacci-Folge ist eine mathematische Folge, die wie folgt definiert ist: Das erste und das zweite Element der Folge sind 1, und ab dem dritten Element ist der Wert jedes Elements gleich der Summe der beiden vorherigen Elemente. Die ersten paar Elemente der Sequenz
 Verbesserter Erkennungsalgorithmus: zur Zielerkennung in hochauflösenden optischen Fernerkundungsbildern
Jun 06, 2024 pm 12:33 PM
Verbesserter Erkennungsalgorithmus: zur Zielerkennung in hochauflösenden optischen Fernerkundungsbildern
Jun 06, 2024 pm 12:33 PM
01Ausblicksübersicht Derzeit ist es schwierig, ein angemessenes Gleichgewicht zwischen Detektionseffizienz und Detektionsergebnissen zu erreichen. Wir haben einen verbesserten YOLOv5-Algorithmus zur Zielerkennung in hochauflösenden optischen Fernerkundungsbildern entwickelt, der mehrschichtige Merkmalspyramiden, Multierkennungskopfstrategien und hybride Aufmerksamkeitsmodule verwendet, um die Wirkung des Zielerkennungsnetzwerks in optischen Fernerkundungsbildern zu verbessern. Laut SIMD-Datensatz ist der mAP des neuen Algorithmus 2,2 % besser als YOLOv5 und 8,48 % besser als YOLOX, wodurch ein besseres Gleichgewicht zwischen Erkennungsergebnissen und Geschwindigkeit erreicht wird. 02 Hintergrund und Motivation Mit der rasanten Entwicklung der Fernerkundungstechnologie wurden hochauflösende optische Fernerkundungsbilder verwendet, um viele Objekte auf der Erdoberfläche zu beschreiben, darunter Flugzeuge, Autos, Gebäude usw. Objekterkennung bei der Interpretation von Fernerkundungsbildern
 Kann künstliche Intelligenz Kriminalität vorhersagen? Entdecken Sie die Möglichkeiten von CrimeGPT
Mar 22, 2024 pm 10:10 PM
Kann künstliche Intelligenz Kriminalität vorhersagen? Entdecken Sie die Möglichkeiten von CrimeGPT
Mar 22, 2024 pm 10:10 PM
Die Konvergenz von künstlicher Intelligenz (KI) und Strafverfolgung eröffnet neue Möglichkeiten zur Kriminalprävention und -aufdeckung. Die Vorhersagefähigkeiten künstlicher Intelligenz werden häufig in Systemen wie CrimeGPT (Crime Prediction Technology) genutzt, um kriminelle Aktivitäten vorherzusagen. Dieser Artikel untersucht das Potenzial künstlicher Intelligenz bei der Kriminalitätsvorhersage, ihre aktuellen Anwendungen, die Herausforderungen, denen sie gegenübersteht, und die möglichen ethischen Auswirkungen der Technologie. Künstliche Intelligenz und Kriminalitätsvorhersage: Die Grundlagen CrimeGPT verwendet Algorithmen des maschinellen Lernens, um große Datensätze zu analysieren und Muster zu identifizieren, die vorhersagen können, wo und wann Straftaten wahrscheinlich passieren. Zu diesen Datensätzen gehören historische Kriminalstatistiken, demografische Informationen, Wirtschaftsindikatoren, Wettermuster und mehr. Durch die Identifizierung von Trends, die menschliche Analysten möglicherweise übersehen, kann künstliche Intelligenz Strafverfolgungsbehörden stärken






