Ermitteln Sie mithilfe der C++-Programmierung die Anzahl der Stopps

X 点和 Y 点之间有 n 个中间火车站。计算可以安排火车在 s 个车站停靠的不同方式的数量，使得没有两个车站彼此相邻。因此，在本文中，我们将解释各种可能的方法来找出停靠站的数量。看看这个问题，我们可以发现我们需要找到可以让火车在 s 个站点停靠的组合。

解决问题的方法

让我们举个例子：有八个中间站，我们需要找到让火车在三个中间站停下的方法。

n = 8, s = 3

我们还有 (n - s) 个车站，即火车无法停靠的五个车站，

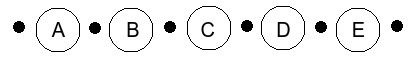
我们有五个车站 A、B、C、D、E，火车不能停靠。现在我们有六个点来安排三个停靠站，使得没有两个站是连续的。因此，方法的数量是 -

6<sub>c<sub>3</sub></sub>= [fact(6) - fact(3)] / fact(3) = 6 * 5 * 4 / 3 * 2 * 1 = 20

有 20 种方法可以从 X 点和 Y 点安排三个停靠站。所以这里是示例 -

Input : n = 15 s = 4
Output : 495
Input : n = 8 s = 3
Output : 20

Example

的中文翻译为：

示例

#include<bits/stdc++.h>
using namespace std;
int main(){
    int n = 8, s = 3;
    int flag1 = 1, flag2 = 1, temp = s, ans;
    // selecting &#39;s&#39; positions out of &#39;n-s+1&#39;
    int x = n - s + 1;
    while (x != (n - 2 * s + 1)) {
       flag1 = flag1 * x;
       x--;
    }
    while (temp != 1) {
       flag2 = flag2 * temp;
       temp--;
    }
    ans = flag1 / flag2;
    if ((n - s + 1) >= s)
       cout << "Number of ways : " << ans;
    else
       cout << "not possible to find";
    return 0;
}

输出

Number of ways : 20

上述代码的解释

为了理解这段C++代码，我们可以将解决方案分为几个步骤。

• 取数字n 中的车站数量和 s 中的停止车站作为输入。

• 用 1 初始化 flag1 和 flag 2 变量，并将 s 的值存储在 temp 中变量。

• 计算flag1，它是分子[fact(n) -fact(r)]。

• 计算flag2，它是是分母[fact(r)]

• 打印结果。

结论

在此在这篇文章中，我们解决了一个问题，找出火车可以在中间站停靠的方式数量，使得没有两个站是连续的。我们还学习了解决这个问题的C++程序以及解决这个问题的完整方法。我们可以用其他语言编写相同的程序，例如C、java、python等语言。

Das obige ist der detaillierte Inhalt vonErmitteln Sie mithilfe der C++-Programmierung die Anzahl der Stopps. Für weitere Informationen folgen Sie bitte anderen verwandten Artikeln auf der PHP chinesischen Website!