How Far You Can Optimize a Program to Compute the Fibonacci Sequence?

How Far Can You Optimize a Program to Compute the Fibonacci Sequence?

Introduction

When I was learning Python, our teacher gave us a homework -- calculate the Nth number of Fibonacci Sequence.

I think it's very easy, so I write this code:

def fib(n):
    if n == 0:
        return 0
    elif n == 1:
        return 1
    else:
        return fib(n - 1) + fib(n - 2)

Later, I know this kind of solution cost too much time.

Optimize a Program

I change the solution to iteration.

def fib(n):
    ls = [1,1]
    for i in range(2,n):
        ls.append(ls[i-1]+ls[i-2])

    return ls[n-1]

I use matplotlib draw the time it cost:

import time
import matplotlib.pyplot as plt


def bench_mark(func, *args):
    # calculate the time
    start = time.perf_counter()
    result = func(*args)
    end = time.perf_counter()

    return end - start, result  # return the time and the result

def fib(n):
    ls = [1,1]
    for i in range(2,n):
        ls.append(ls[i-1]+ls[i-2])

    return ls[n-1]

mark_list = []

for i in range(1,10000):
    mark = bench_mark(fib,i)
    mark_list.append(mark[0])
    print(f"size : {i} , time : {mark[0]}")

plt.plot(range(1, 10000), mark_list)
plt.xlabel('Input Size')
plt.ylabel('Execution Time (seconds)')
plt.title('Test fot fibonacci')
plt.grid(True)
plt.show()

Result Here:

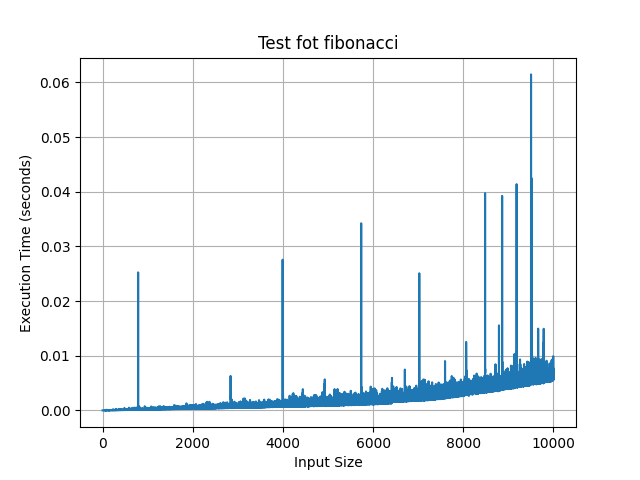
The time it cost is very short.

But I write fib(300000), cost 5.719049899998936 seconds. It's too long.

When I grow up, I learn CACHE, so I change the solution to use dict to store the result.

from functools import lru_cache
@lru_cache(maxsize=None)
def fib(n):
    if n < 2:
        return 1
    else:
        return fib(n - 1) + fib(n - 2)

Or we can write the CACHE by ourself.

def fib(n, cache={}):
    if n in cache:
        return cache[n]
    elif n < 2:
        return 1
    else:
        ls = [1, 1]
        for i in range(2, n):
            next_value = ls[-1] + ls[-2]
            ls.append(next_value)
            cache[i] = next_value
        cache[n-1] = ls[-1]
        return ls[-1]

The above is the detailed content of How Far You Can Optimize a Program to Compute the Fibonacci Sequence?. For more information, please follow other related articles on the PHP Chinese website!