Recursion on the basis of PHP data structure
This article mainly introduces the recursion on the basis of PHP data structure, which has certain reference value. Now I share it with you. Friends in need can refer to it
What is recursion?
As mentioned before, recursion is a solution that breaks down large problems into small ones. Generally speaking, recursion is called a call to the function itself. It may sound strange to say this, but in fact in recursion the function does have to call itself.
A chestnut
For example, in mathematics, we all know the concept of "factorial". For example, the factorial of 5 is 5*4*3*2*1.
5! = 5 * 4!
4! = 4 * 3!
3! = 3 * 2!
2! = 2 * 1!
1! = 1 * 0!
0! = 1
We can summarize the rule for finding the factorial of n, that is, n! = n * (n -1) !
This reflects recursion. You can find from this that we transformed the factorial of 5 into another small problem step by step.
Characteristics of recursive algorithms
Every recursive call must be based on a small sub-problem. For example, the factorial of 5 is the factorial of 5 times 4.
Recursion must have a Base case. For example, the base case of factorial is 0. When the condition is 0, the recursion stops.
Avoid loop calls during recursion, otherwise the computer will display a stack overflow error in the end.
function factorial(int $n): int
{
if ($n = 0) {
return 1;
}
return $n * factorial($n - 1);
}Looking at the above code, we can see that we have a basic condition for the solution to the factorial problem, which is that when n is 0, we return 1. If this condition is not met, we return n multiplied by factorial(n), which meets the first and third items of the recursion property. We avoid looping calls because we break each recursive call into a smaller sub-problem of the larger problem. The above algorithm idea can be expressed as:
Recursion Vs iteration
We can also use the iterative method to implement the above recursive code
function factorial(int $n): int
{
$result = 1;
for ($i = $n; $i > 0; $i--) {
$result*= $n;
}
return $result;
}If a problem can be very It's easy to use iteration to solve, why do we use recursion?
Recursion is used to deal with more complex problems. Not all problems can be solved simply using iteration. Recursion uses function calls to manage the call stack, so recursion uses more time and memory than iteration. Furthermore, in iteration, we will have a result at each step, but in recursion we have to wait until the base case execution ends before we have any result. Looking at the above example, we find that in the recursive algorithm we do not have any variables or declarations to save the results, while in the iterative algorithm we use $result to save the returned results each time.
Fibonacci Sequence
In mathematics, the Fibonacci Sequence is a special integer sequence. Each number in the sequence is generated by the sum of two other numbers. . The rules are as follows:
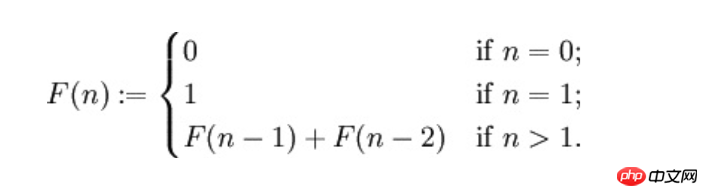
function fibonacci($n)
{
if ($n == 0) {
return 0;
}
if ($n == 1) {
return 1;
}
return fibonacci($n - 1) + fibonacci($ - 2);
}Greatest Common Factor
Another common problem using recursive algorithms is to find the greatest common factor of two numbers.
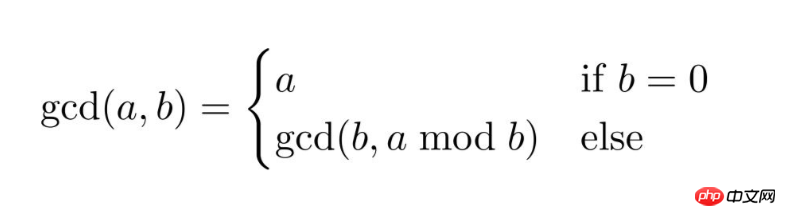
function gcd(int $a, int $b)
{
if ($b == 0) {
return $a;
}
return gcd($b, $a % $b);
}Recursion type
Linear recursion
In every recursive call , the function only calls itself once, which is called linear recursion.
Biary recursion
In binary recursion, each recursive call to the function calls itself twice. The algorithm for solving the Fibonacci sequence is binary recursion. In addition, binary search, divide and conquer algorithm, merge sort, etc. also use binary recursion.
Tail recursion
When a recursion returns without waiting for operations, it is called tail recursion. In the Fibonacci algorithm, the return value needs to be multiplied by the return value of the previous recursion, so it is not tail recursive, and the algorithm for solving the greatest common factor is tail recursive. Tail recursion is a form of linear recursion.
Mutual recursion
For example, in each recursive call, A() calls B(), and B() calls A(). Such recursion is called mutual recursion.
Nested recursion
When a recursive function calls itself recursively as a parameter, it is called nested recursion. A common example is the Ackerman function, see the expression below.
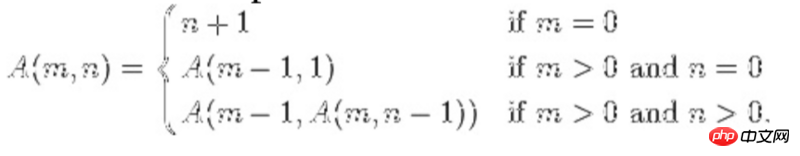
#Looking at the last line, you can see that the second parameter is the recursive function itself.
Next section
The next article will use recursion to solve some problems encountered in actual development, such as building N-level classifications, building nested comments, traversing directory files, etc. .
The above is the entire content of this article. I hope it will be helpful to everyone's study. For more related content, please pay attention to the PHP Chinese website!
Related recommendations:
How to obtain the real IP address of the client in PHP
How to use Elasticsearch in PHP
The above is the detailed content of Recursion on the basis of PHP data structure. For more information, please follow other related articles on the PHP Chinese website!

Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

Video Face Swap
Swap faces in any video effortlessly with our completely free AI face swap tool!

Hot Article

Hot Tools

Notepad++7.3.1
Easy-to-use and free code editor

SublimeText3 Chinese version
Chinese version, very easy to use

Zend Studio 13.0.1
Powerful PHP integrated development environment

Dreamweaver CS6
Visual web development tools

SublimeText3 Mac version
God-level code editing software (SublimeText3)

Hot Topics
 1386
1386
 52
52
 Recursive implementation of C++ functions: Is there a limit to recursion depth?
Apr 23, 2024 am 09:30 AM
Recursive implementation of C++ functions: Is there a limit to recursion depth?
Apr 23, 2024 am 09:30 AM
The recursion depth of C++ functions is limited, and exceeding this limit will result in a stack overflow error. The limit value varies between systems and compilers, but is usually between 1,000 and 10,000. Solutions include: 1. Tail recursion optimization; 2. Tail call; 3. Iterative implementation.
 Do C++ lambda expressions support recursion?
Apr 17, 2024 pm 09:06 PM
Do C++ lambda expressions support recursion?
Apr 17, 2024 pm 09:06 PM
Yes, C++ Lambda expressions can support recursion by using std::function: Use std::function to capture a reference to a Lambda expression. With a captured reference, a Lambda expression can call itself recursively.
 Recursive implementation of C++ functions: Comparative analysis of recursive and non-recursive algorithms?
Apr 22, 2024 pm 03:18 PM
Recursive implementation of C++ functions: Comparative analysis of recursive and non-recursive algorithms?
Apr 22, 2024 pm 03:18 PM
The recursive algorithm solves structured problems through function self-calling. The advantage is that it is simple and easy to understand, but the disadvantage is that it is less efficient and may cause stack overflow. The non-recursive algorithm avoids recursion by explicitly managing the stack data structure. The advantage is that it is more efficient and avoids the stack. Overflow, the disadvantage is that the code may be more complex. The choice of recursive or non-recursive depends on the problem and the specific constraints of the implementation.
 Count the number of occurrences of a substring recursively in Java
Sep 17, 2023 pm 07:49 PM
Count the number of occurrences of a substring recursively in Java
Sep 17, 2023 pm 07:49 PM
Given two strings str_1 and str_2. The goal is to count the number of occurrences of substring str2 in string str1 using a recursive procedure. A recursive function is a function that calls itself within its definition. If str1 is "Iknowthatyouknowthatiknow" and str2 is "know" the number of occurrences is -3. Let us understand through examples. For example, input str1="TPisTPareTPamTP", str2="TP"; output Countofoccurrencesofasubstringrecursi
 Recursive program to find minimum and maximum elements of array in C++
Aug 31, 2023 pm 07:37 PM
Recursive program to find minimum and maximum elements of array in C++
Aug 31, 2023 pm 07:37 PM
We take the integer array Arr[] as input. The goal is to find the largest and smallest elements in an array using a recursive method. Since we are using recursion, we will iterate through the entire array until we reach length = 1 and then return A[0], which forms the base case. Otherwise, the current element is compared to the current minimum or maximum value and its value is updated recursively for subsequent elements. Let’s look at various input and output scenarios for this −Input −Arr={12,67,99,76,32}; Output −Maximum value in the array: 99 Explanation &mi
 Detailed explanation of C++ function recursion: application of recursion in string processing
Apr 30, 2024 am 10:30 AM
Detailed explanation of C++ function recursion: application of recursion in string processing
Apr 30, 2024 am 10:30 AM
A recursive function is a technique that calls itself repeatedly to solve a problem in string processing. It requires a termination condition to prevent infinite recursion. Recursion is widely used in operations such as string reversal and palindrome checking.
 C++ Recursion Advanced: Understanding Tail Recursion Optimization and Its Application
Apr 30, 2024 am 10:45 AM
C++ Recursion Advanced: Understanding Tail Recursion Optimization and Its Application
Apr 30, 2024 am 10:45 AM
Tail recursion optimization (TRO) improves the efficiency of certain recursive calls. It converts tail-recursive calls into jump instructions and saves the context state in registers instead of on the stack, thereby eliminating extra calls and return operations to the stack and improving algorithm efficiency. Using TRO, we can optimize tail recursive functions (such as factorial calculations). By replacing the tail recursive call with a goto statement, the compiler will convert the goto jump into TRO and optimize the execution of the recursive algorithm.
 A beginner's guide to C++ recursion: Building foundations and developing intuition
May 01, 2024 pm 05:36 PM
A beginner's guide to C++ recursion: Building foundations and developing intuition
May 01, 2024 pm 05:36 PM
Recursion is a powerful technique that allows a function to call itself to solve a problem. In C++, a recursive function consists of two key elements: the base case (which determines when the recursion stops) and the recursive call (which breaks the problem into smaller sub-problems ). By understanding the basics and practicing practical examples such as factorial calculations, Fibonacci sequences, and binary tree traversals, you can build your recursive intuition and use it in your code with confidence.





