Understand the Vue Diff algorithm in one article-Vue.js-php.cn

This article brings you relevant knowledge about vue, which mainly introduces related issues about the diff algorithm. The function of the diff algorithm is to find the difference between the two sets of vdom nodes. And reuse DOM nodes as much as possible so that the update operation can be completed with minimal performance consumption. Let's take a look at it together. I hope it will be helpful to everyone.

Understand the Vue Diff algorithm in one article

【Related recommendations: javascript video tutorial, vue.js tutorial】

Why Need diff algorithm?

For a container (such as our commonly used #app), its content generally has three situations:

String type, which is text .
Child node array, that is, it contains one or more child nodes
null, that is, there is no child node

In vue, the dom element will be processed as vdom. Our HTML Attributes and event binding will be processed on vdom, and finally rendered into the real dom.

Virtual Dom: JavaScript object used to describe real dom nodes.

The reason for using vdom is that if each operation is performed directly on the real dom, it will cause a lot of overhead. When using vdom, the performance consumption can be reduced from the level of real dom operations to the JavaScript level, which is relatively better. A simple vdom is as follows:

const vdom = {
    type:"div",
    props:{
        class: "class",
        onClick: () => { console.log("click") }
    },
    children: [] // 简单理解这就是上述三种内容
}

Copy after login

For the update of the vue node, the vdom is used for comparison.

The diff algorithm is the second case used for container content. When the content in the container before updating is a set of child nodes, and the content after updating is still a set of nodes. If the diff algorithm is not used, the simplest operation is to uninstall all the previous DOM and then mount all the current new nodes.

But directly operating dom objects is very performance-intensive, so the role of the diff algorithm is to find the difference between the two sets of vdom nodes and reuse the dom nodes as much as possible to minimize performance consumption. Complete the update operation.

Next, let’s talk about three diff algorithms, starting from simple to complex step by step.

Simple Diff algorithm

Why do you need a key?

Next, we will explain why a key is needed through two situations?

If there are two sets of old and new node arrays as follows:

const oldChildren = [
    {type: &#39;p&#39;},
    {type: &#39;span&#39;},
    {type: &#39;div&#39;},
]
const newChildren = [
    {type: &#39;div&#39;},
    {type: &#39;p&#39;},
    {type: &#39;span&#39;},
    {type: &#39;footer&#39;},
]

Copy after login

If we are performing a normal comparison, the steps should be as follows:

Find the relatively shorter group Perform a circular comparison

The first p tag does not match the div tag. You need to uninstall the p tag first and then mount the div tag.
The first spam tag does not match the p tag. You need to uninstall the span tag first and then mount the p tag.
The first div tag does not match the span tag. You need to uninstall the div tag first and then mount the span tag.
The last extra label footer exists in the new node array, just mount it.

Then we found that 7 DOM operations were performed, but the first three names can be reused, but the positions have changed. If we reuse nodes, we need to determine whether the two nodes are equal, but the current existing conditions cannot be met.

So we need to introduce key, which is equivalent to the ID number of the virtual node. As long as the type and key of the two virtual nodes are the same, we consider them equal and can reuse DOM.

At this time, we can find the reused elements and move the dom, which is relatively better than constantly mounting and unloading the nodes.

However, the reuse of dom does not mean that there is no need to update:

const oldVNode = {type: &#39;p&#39;, children: &#39;old&#39;, key: 1}
const newVNode = {type: &#39;p&#39;, children: &#39;new&#39;, key: 2}

Copy after login

The above nodes have the same type and key, we can reuse them, and just update the child nodes at this time.

Simple diff algorithm steps

First use an example to illustrate the entire process, and then describe the method

const oldChildren = [
    {type: &#39;p&#39;, children: &#39;p&#39;, key: 1},
    {type: &#39;span&#39;, children: &#39;span&#39;, key: 2},
    {type: &#39;div&#39;, children: &#39;div&#39;, key: 3},
    {type: &#39;section&#39;, children: &#39;section&#39;, key : 4},
]
const newChildren = [
    {type: &#39;div&#39;, children: &#39;new div&#39;, key: 3},
    {type: &#39;p&#39;, children: &#39;p&#39;, key: 1},
    {type: &#39;span&#39;, children: &#39;span&#39;, key: 2},
    {type: &#39;footer&#39;, children: &#39;footer&#39;, key: 5},
]

Copy after login

For the sake of description Simple, use different tags here. The whole process is as follows:

Understand the Vue Diff algorithm in one article

##Traverse from the new node array
The first one is the div tag, currently The subscript of is 0, and the previous subscript was 2. The relative position has not changed, no movement is required, only the node content needs to be updated.
The second one is the p tag, the current subscript is 1, and the previous subscript is 0. In terms of relative position, p has changed relative to the div tag and needs to be moved. The moved position is after the div tag.
第三个是span标签，当前的下标是2，之前的下标是1。就相对位置而言，p相对于div标签有变化，需要进行移动。移动的位置就是在p标签之后。
第四个标签是footer，遍历旧节点数组发现并无匹配的元素。代表当前的元素是新节点，将其插入，插入的位置是span标签之后。
最后一步，遍历旧节点数组，并去新节点数组中查找是否有对应的节点，没有则卸载当前的元素。

如何找到需要移动的元素？

上述声明了一个lastIdx变量，其初始值为0。作用是保存在新节点数组中，对于已经遍历了的新节点在旧节点数组的最大的下标。那么对于后续的新节点来说，只要它在旧节点数组中的下标的值小于当前的lastIdx，代表当前的节点相对位置发生了改变，则需要移动，

举个例子：div在旧节点数组中的位置为2，大于当前的lastIdx，更新其值为2。对于span标签，它的旧节点数组位置为1，其值更小。又因为当前在新节点数组中处于div标签之后，就是相对位置发生了变化，便需要移动。

当然，lastIdx需要动态维护。

总结

简单diff算法便是拿新节点数组中的节点去旧节点数组中查找，通过key来判断是否可以复用。并记录当前的lastIdx，以此来判断节点间的相对位置是否发生变化，如果变化，需要进行移动。

双端diff算法

简单diff算法并不是最优秀的，它是通过双重循环来遍历找到相同key的节点。举个例子：

const oldChildren = [
    {type: &#39;p&#39;, children: &#39;p&#39;, key: 1},
    {type: &#39;span&#39;, children: &#39;span&#39;, key: 2},
    {type: &#39;div&#39;, children: &#39;div&#39;, key: 3},
]
const newChildren = [
    {type: &#39;div&#39;, children: &#39;new div&#39;, key: 3},
    {type: &#39;p&#39;, children: &#39;p&#39;, key: 1},
    {type: &#39;span&#39;, children: &#39;span&#39;, key: 2},
]

Copy after login

其实不难发现，我们只需要将div标签节点移动即可，即进行一次移动。不需要重复移动前两个标签也就是p、span标签。而简单diff算法的比较策略即是从头至尾的循环比较策略，具有一定的缺陷。

顾名思义，双端diff算法是一种同时对新旧两组子节点的两个端点进行比较的算法

Understand the Vue Diff algorithm in one article

那么双端diff算法开始的步骤如下：

比较 oldStartIdx节点与 newStartIdx 节点，相同则复用并更新，否则
比较 oldEndIdx节点与 newEndIdx 节点，相同则复用并更新，否则
比较 oldStartIdx节点与 newEndIdx 节点，相同则复用并更新，否则
比较 oldEndIdx节点与 newStartIdx 节点，相同则复用并更新，否则

简单概括：

旧头 === 新头？复用，不需移动
旧尾 === 新尾？复用，不需移动
旧头 === 新尾？复用，需要移动
旧尾 === 新头？复用，需要移动

对于上述例子而言，比较步骤如下：

Understand the Vue Diff algorithm in one article

上述的情况是一种非常理想的情况，我们可以根据现有的diff算法完全的处理两组节点，因为每一轮的双端比较都会命中其中一种情况使得其可以完成处理。

但往往会有其他的情况，比如下面这个例子：

const oldChildren = [
    {type: &#39;p&#39;, children: &#39;p&#39;, key: 1},
    {type: &#39;span&#39;, children: &#39;span&#39;, key: 2},
    {type: &#39;div&#39;, children: &#39;div&#39;, key: 3},
    {type: &#39;ul&#39;, children: &#39;ul&#39;, key: 4},
]
const newChildren = [
    {type: &#39;div&#39;, children: &#39;new div&#39;, key: 3},
    {type: &#39;p&#39;, children: &#39;p&#39;, key: 1},
    {type: &#39;ul&#39;, children: &#39;ul&#39;, key: 4},
    {type: &#39;span&#39;, children: &#39;span&#39;, key: 2},
]

Copy after login

此时我们会发现，上述的四个步骤都会无法命中任意一步。所以需要额外的步骤进行处理。即是：在四步比较失败后，找到新头节点在旧节点中的位置，并进行移动即可。动图示意如下：

Understand the Vue Diff algorithm in one article

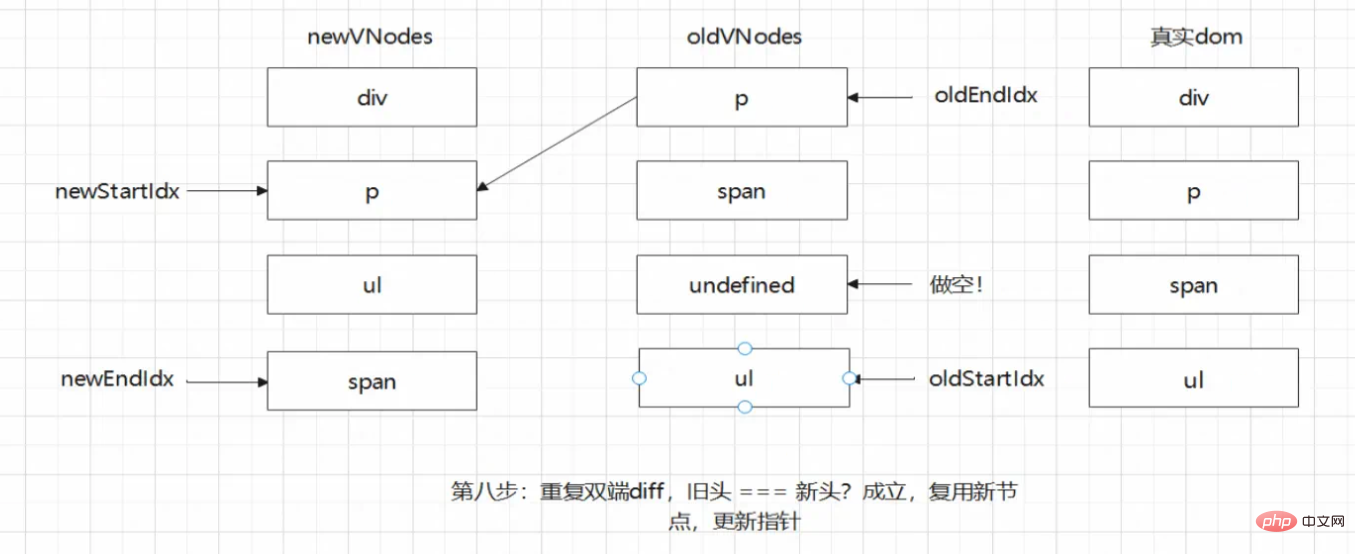
当然还有删除、增加等均不满足上述例子的操作，但操作核心一致，这里便不再赘述。

总结

双端diff算法的优势在于对于一些比较特殊的情况能更快的对节点进行处理，也更贴合实际开发。而双端的含义便在于通过两组子节点的头尾分别进行比较并更新。

快速diff算法

首先，快速diff算法包含了预处理步骤。它借鉴了纯文本diff的思路，这时它为何快的原因之一。

比如：

const text1 = &#39;我是快速diff算法&#39;
const text2 =  &#39;我是双端diff算法&#39;

Copy after login

那么就会先从头比较并去除可用元素，其次会重后比较相同元素并复用，那么结果就会如下：

const text1 = &#39;快速&#39;
const text2 = &#39;双端&#39;

Copy after login

此时再进行一些其他的比较和处理便会简单很多。

其次，快速diff算法还使用了一种算法来尽可能的复用dom节点，这个便是最长递增子序列算法。为什么要用呢？先举个例子：

// oldVNodes
const vnodes1 = [
    {type:&#39;p&#39;, children: &#39;p1&#39;, key: 1},
    {type:&#39;div&#39;, children: &#39;div&#39;, key: 2},
    {type:&#39;span&#39;, children: &#39;span&#39;, key: 3},
    {type:&#39;input&#39;, children: &#39;input&#39;, key: 4},
    {type:&#39;a&#39;, children: &#39;a&#39;, key: 6}
    {type:&#39;p&#39;, children: &#39;p2&#39;, key: 5},
]
// newVNodes 
const vnodes2 = [
    {type:&#39;p&#39;, children: &#39;p1&#39;, key: 1},
    {type:&#39;span&#39;, children: &#39;span&#39;, key: 3},
    {type:&#39;div&#39;, children: &#39;div&#39;, key: 2},
    {type:&#39;input&#39;, children: &#39;input&#39;, key: 4},
    {type:&#39;p&#39;, children: &#39;p2&#39;, key: 5},
]

Copy after login

经过预处理步骤之后得到的节点如下：

// oldVNodes
const vnodes1 = [
    {type:&#39;div&#39;, children: &#39;div&#39;, key: 2},
    {type:&#39;span&#39;, children: &#39;span&#39;, key: 3},
    {type:&#39;input&#39;, children: &#39;input&#39;, key: 4},
    {type:&#39;a&#39;, children: &#39;a&#39;, key: 6},
]
// newVNodes 
const vnodes2 = [
    {type:&#39;span&#39;, children: &#39;span&#39;, key: 3},
    {type:&#39;div&#39;, children: &#39;div&#39;, key: 2},
    {type:&#39;input&#39;, children: &#39;input&#39;, key: 4},
]

Copy after login

此时我们需要获得newVNodes节点相对应oldVNodes节点中的下标位置，我们可以采用一个source数组，先循环遍历一次newVNodes，得到他们的key，再循环遍历一次oldVNodes，获取对应的下标关系，如下：

const source = new Array(restArr.length).fill(-1)
// 处理后
source = [1, 2, 0, -1]

Copy after login

注意！这里的下标并不是完全正确！因为这是预处理后的下标，并不是刚开始的对应的下标值。此处仅是方便讲解。其次，source数组的长度是剩余的newVNodes的长度，若在处理完之后它的值仍然是-1则说明当前的key对应的节点在旧节点数组中没有，即是新增的节点。

此时我们便可以通过source求得最长的递增子序列的值为 [1, 2] 。对于index为1，2的两个节点来说，他们的相对位置在原oldVNodes中是没有变化的，那么便不需要移动他们，只需要移动其余的元素。这样便能达到最大复用dom的效果。

步骤

以上述例子来说：

首先进行预处理

Understand the Vue Diff algorithm in one article

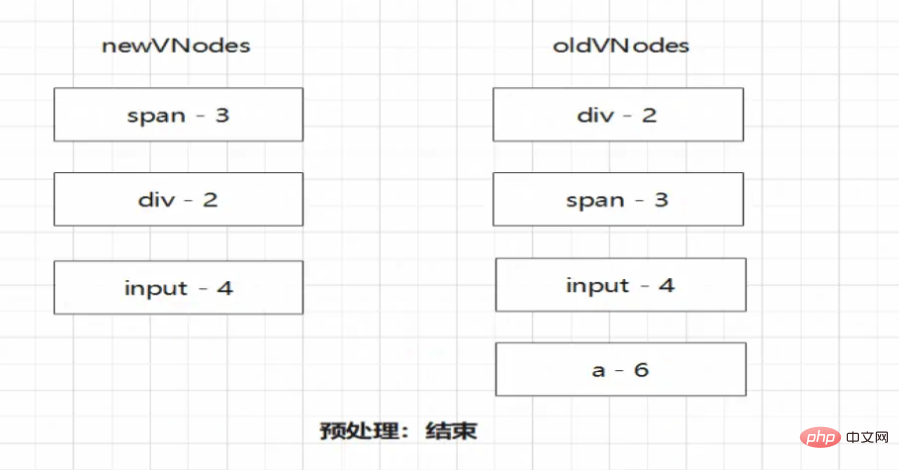
注意！预处理过的节点虽然复用，但仍然需要进行更新。

进行source填充

Understand the Vue Diff algorithm in one article

当然这里递增子序列 [1, 2] 和 [0, 1]都是可以的。

进行节点移动

用索引i指向新节点数组中的最后一个元素

用索引s指向最长递增子序列中的最后一个元素

然后循环进行以下步骤比较：

source[i] === -1？等于代表新节点，挂载即可。随后移动i

i === 递增数组[s]? 等于代表当前的节点存在在递增子序列中，是复用的节点，当前的节点无需移动。

上述均不成立代表需要移动节点。

Understand the Vue Diff algorithm in one article

节点更新，结束。

总结

核心思路便是进行了类似文本预处理的步骤去除头尾重复的节点。其次便是采用了最长递增子序列来复用相对位置没有发生变化的节点，这些节点是不需要移动的，便能最快的复用和更新。

最后

其实不论是哪一种diff算法，它都需要遵循同样的处理规则：

判断哪些节点需要移动，以及如何移动。

找出那些需要被添加的或者被移除的节点。

【相关推荐：javascript视频教程、vue.js教程】

The above is the detailed content of Understand the Vue Diff algorithm in one article. For more information, please follow other related articles on the PHP Chinese website!