
First of all, let’s add a supplementary explanation of what a binary search tree is:
In a binary search tree, for each For a node, the values in its left subtree are smaller than it, and the values in its right subtree are larger than it. So the in-order traversal of a binary search tree is an ordered set of data.

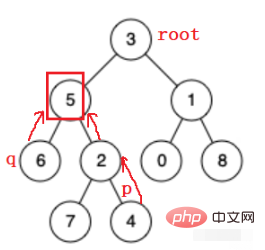
#For the above tree, assume that the nearest common ancestor of p q is required.
Then it has the following situations:
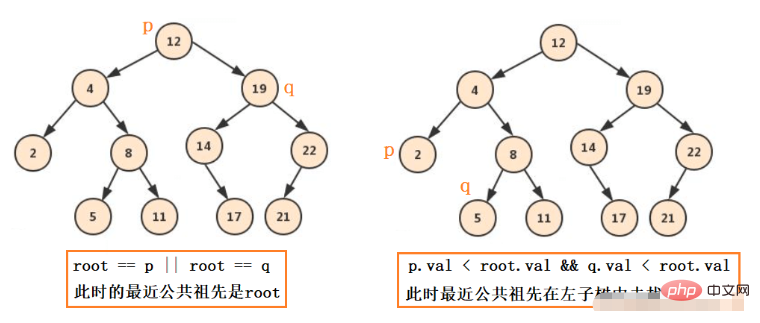
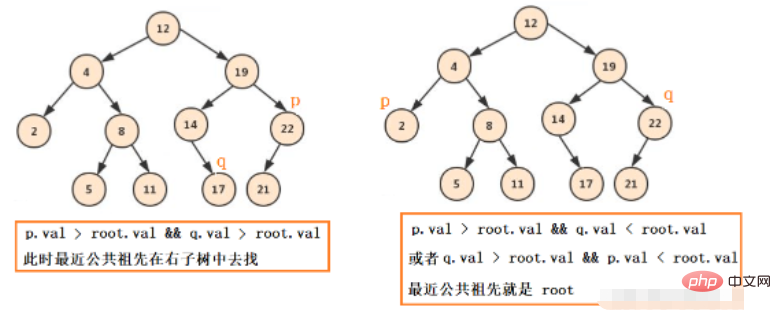
For ordinary binary trees, there are nothing more than these situations. : pq are all on the left, pq are all on the right, pq is one on the left and one on the right, and one of pq is the root node.
So recursively go to the left subtree and right subtree to find the common ancestor of the p q node. If it is found, the node will be returned. If it is not found, it will return empty.
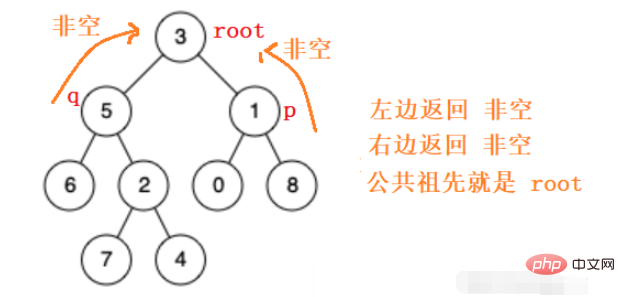
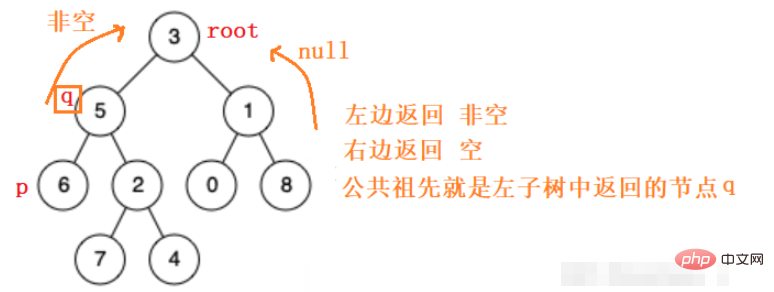

public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
if(root == null) return null;
// p 为当前树的根节点
if(p == root) return p;
// q 为当前树的根节点
if(q == root) return q;
// 去左子树中找
TreeNode left = lowestCommonAncestor(root.left,p,q);
// 去右子树中找
TreeNode right = lowestCommonAncestor(root.right,p,q);
// 左边右边都找到了
if(left != null && right != null) {
return root;
}
// 左边找到了,右边没找到
if(left != null) {
return left;
}
if(right != null) {
return right;
}
return null;
}
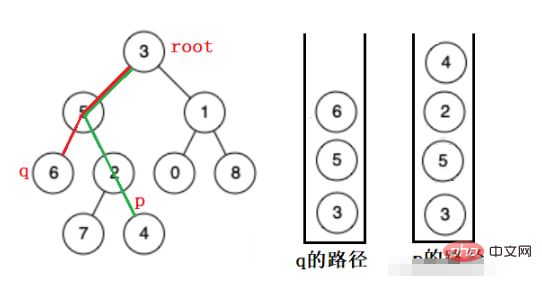
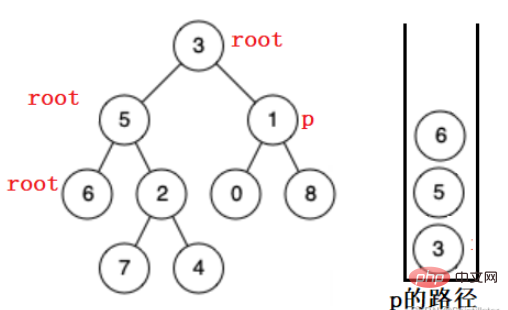
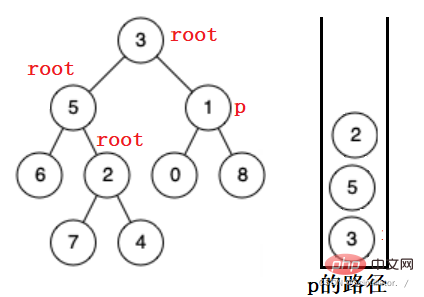
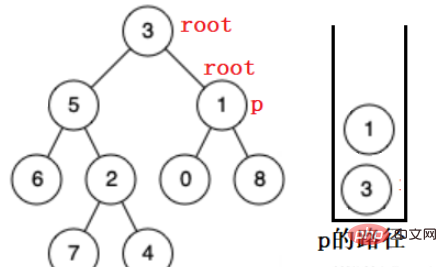
// 用于找节点的路径
public boolean getPath(TreeNode root, TreeNode node, Stack<TreeNode> stack) {
if(root == null || node == null) {
return false;
}
// 将当前节点放入栈中
stack.push(root);
if(root.val == node.val) {
return true;// 找到了
}
// 当前节点没找到,去左子树找
boolean flag = getPath(root.left,node,stack);
// 左子树中找到了,直接返回
if(flag) {
return true;
}
// 左子树没找到,去右子树找
flag = getPath(root.right,node,stack);
// 右子树中找到了,直接返回
if(flag) {
return true;
}
// 左右子树都没找到,弹出节点
stack.pop();
return false;
}
public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
if(root == null) {
return null;
}
Stack<TreeNode> stackp = new Stack<>();
Stack<TreeNode> stackq = new Stack<>();
// 分别得到 p q 的路径
getPath(root,p,stackp);
getPath(root,q,stackq);
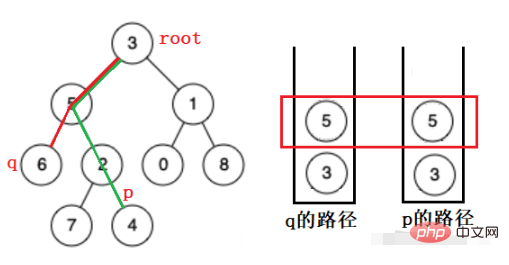
int sizep = stackp.size();
int sizeq = stackq.size();
if(sizep > sizeq) {
int size = sizep - sizeq;
// 弹出元素直至两栈中元素个数相等
while(size > 0) {
stackp.pop();
size--;
}
}else {
int size = sizeq - sizep;
// 弹出元素直至两栈中元素个数相等
while(size > 0) {
stackq.pop();
size--;
}
}
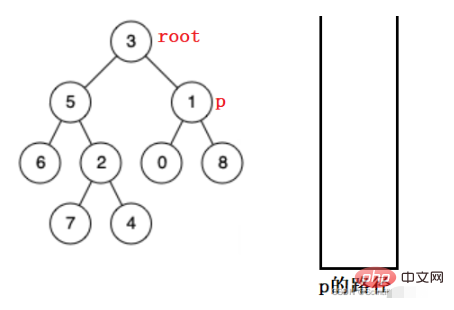
// 一起弹出,直到找到第一个相同的元素
while(!stackp.isEmpty() && !stackq.isEmpty()) {
if(stackp.peek() == stackq.peek()) {
// 找到了,就返回该节点
return stackq.pop();
}else {
stackp.pop();
stackq.pop();
}
}
// 没找到,返回 null
return null;
}The above is the detailed content of How to find the nearest common ancestor of a binary tree in Java. For more information, please follow other related articles on the PHP Chinese website!




