Count the number of N-digit numbers that do not have a given prefix

The problem here is to determine the total number of characters '0' to '9' contained in a string of length N, providing an integer N and a string prefix array pre[] , such that none of these strings contains the provided prefix. The purpose of this article is to implement a program that finds the number of N-digit numbers that does not have a given prefix.
In the C programming language, a set of distinct strings is called an array because an array is a linear combination of a set of data fragments of similar types.
As we already know, the string is a character-by-character, one-dimensional array that ends with an empty or a null character.
Example Example 1
Let us assume that the input N = 2,
The given prefix, pre = {“1”}
Output obtained: 90
explain
Here, except {"01","10","11", "12", "13", "14", "15", "16", "17", "18", "19 ", "21", "31", "41", "51", "61", "71", "81", "91"} are valid.
Example Example 2
Let us take the input value N = 3 as an example.
The given prefix, pre = {“56”}
Output obtained: 990
explain
Here, except {"560", "561", "562", "563", "564", "565", "566", "567", "568", "569"} All 3-digit strings are valid.
ExampleExample 3
Let’s look at an input N = 1,
The given prefix, pre = {“6”}
Output obtained: 9
explain
Except {"6"}, all 1-digit strings here are valid.
Problem Statement
Implement a program to find the number of N-digit numbers that does not have a given prefix.
method
To find the number of N digits without a given prefix, we use the following method.
Solve this problem and find the way to N number of digits that does not have the given prefix
Considering that there are 10 character options at each position in the string, there are (10N) potential strings in total. Instead of counting the total number of strings you want, subtract the total number of strings you don't want. Merging prefixes with the same initial characters into a longer prefix before iteration may result in the removal of some duplicates.
algorithm
Finding algorithm for counting N digits that does not have the following given prefix
First Step − Start
Step 2 - Define a function to count the total number of strings of length N that do not contain the given prefix
Step 3 - Calculate the total number of existing strings
Step 4 - Create an array and counters a and aCount and insert these prefixes into it
Step 5 − Create a new prefix string array
Step 6 - Iterate for each starting character
Step 7 - Iterate over the array to calculate the minimum size of the prefix
Step 8 - Now put all these minimal prefixes into a new prefix array
Step 9 - Iterate over new prefixes
Step 10 - Deduct unnecessary strings
Step 11 − Print the obtained results
Step 12 − Stop
Example: C program
This is a C program implementation of the above algorithm to find the number of N digits that does not have a given prefix.
#include <stdio.h>
#include <math.h>
#include <string.h>
#define MAX_LENGTH 10
// Function to calculate total strings of length N without the given prefixes
int totalStrings(int N, char pre[][MAX_LENGTH], int pre_Count){
// Calculate total strings present
int total = (int)(pow(10, N) + 0.5);
// Make an array and counter a and aCount respectively and insert these prefixes with same character in the array
char a[10][MAX_LENGTH];
int aCount[10] = {0};
for (int i = 0; i < pre_Count; i++) {
int index = pre[i][0] - '0';
strcpy(a[index] + aCount[index] * MAX_LENGTH, pre[i]);
aCount[index]++;
}
// Make a new array of prefixes strings
char new_pre[pre_Count][MAX_LENGTH];
int new_pre_count = 0;
// Iterating for each of the starting //character
for (int x = 0; x < 10; x++){
int m = N;
// Iterate over the array to calculate minimum size prefix
for (int j = 0; j < aCount[x]; j++){
int p_length = strlen(a[x] + j * MAX_LENGTH);
m = (m < p_length) ? m : p_length;
}
// now take all these minimum prefixes in the new array of prefixes
for (int j = 0; j < aCount[x]; j++){
int p_length = strlen(a[x] + j * MAX_LENGTH);
if (p_length <= m){
strcpy(new_pre[new_pre_count], a[x] + j * MAX_LENGTH);
new_pre_count++;
}
}
}
// Iterating through the new prefixes
for (int i = 0; i < new_pre_count; i++){
// Subtract the unwanted strings
total -= (int)(pow(10, N - strlen(new_pre[i])) + 0.5);
}
return total;
}
// The main function
int main(){
int N = 5;
char pre[][MAX_LENGTH] = {"1", "0", "2"};
int pre_Count = sizeof(pre) / sizeof(pre[0]);
printf("%d\n", totalStrings(N, pre, pre_Count));
return 0;
}
Output
70000
in conclusion
Similarly, we can find the number of N digits that does not have the given prefix.
In this post, the challenge of getting a program to find an N-digit count that does not have a given prefix is addressed.
C programming code is provided here along with the algorithm to find the count of N-digit numbers that do not have a given prefix.
The above is the detailed content of Count the number of N-digit numbers that do not have a given prefix. For more information, please follow other related articles on the PHP Chinese website!

Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

AI Hentai Generator
Generate AI Hentai for free.

Hot Article

Hot Tools

Notepad++7.3.1
Easy-to-use and free code editor

SublimeText3 Chinese version
Chinese version, very easy to use

Zend Studio 13.0.1
Powerful PHP integrated development environment

Dreamweaver CS6
Visual web development tools

SublimeText3 Mac version
God-level code editing software (SublimeText3)

Hot Topics
 CUDA's universal matrix multiplication: from entry to proficiency!
Mar 25, 2024 pm 12:30 PM
CUDA's universal matrix multiplication: from entry to proficiency!
Mar 25, 2024 pm 12:30 PM
General Matrix Multiplication (GEMM) is a vital part of many applications and algorithms, and is also one of the important indicators for evaluating computer hardware performance. In-depth research and optimization of the implementation of GEMM can help us better understand high-performance computing and the relationship between software and hardware systems. In computer science, effective optimization of GEMM can increase computing speed and save resources, which is crucial to improving the overall performance of a computer system. An in-depth understanding of the working principle and optimization method of GEMM will help us better utilize the potential of modern computing hardware and provide more efficient solutions for various complex computing tasks. By optimizing the performance of GEMM
 How to calculate addition, subtraction, multiplication and division in word document
Mar 19, 2024 pm 08:13 PM
How to calculate addition, subtraction, multiplication and division in word document
Mar 19, 2024 pm 08:13 PM
WORD is a powerful word processor. We can use word to edit various texts. In Excel tables, we have mastered the calculation methods of addition, subtraction and multipliers. So if we need to calculate the addition of numerical values in Word tables, How to subtract the multiplier? Can I only use a calculator to calculate it? The answer is of course no, WORD can also do it. Today I will teach you how to use formulas to calculate basic operations such as addition, subtraction, multiplication and division in tables in Word documents. Let's learn together. So, today let me demonstrate in detail how to calculate addition, subtraction, multiplication and division in a WORD document? Step 1: Open a WORD, click [Table] under [Insert] on the toolbar, and insert a table in the drop-down menu.
 How to count the number of elements in a list using Python's count() function
Nov 18, 2023 pm 02:53 PM
How to count the number of elements in a list using Python's count() function
Nov 18, 2023 pm 02:53 PM
How to use Python's count() function to calculate the number of an element in a list requires specific code examples. As a powerful and easy-to-learn programming language, Python provides many built-in functions to handle different data structures. One of them is the count() function, which can be used to count the number of elements in a list. In this article, we will explain how to use the count() function in detail and provide specific code examples. The count() function is a built-in function of Python, used to calculate a certain
 How to quickly add a prefix in Excel? How to add prefixes to Excel tables in batches
Mar 14, 2024 am 09:16 AM
How to quickly add a prefix in Excel? How to add prefixes to Excel tables in batches
Mar 14, 2024 am 09:16 AM
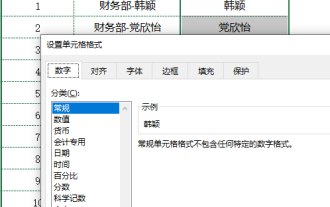
When editing Excel, you may need to add the same prefix to a column of data. If you add them one by one, it is a waste of time. Is there any way to add prefixes to Excel in batches? Of course there are, and here are some commonly used methods of adding prefixes. How to quickly add a prefix in Excel? 1. Cell formatting method 1. Select the cell range and press Ctrl1 at the same time to set the cell format. (Or right-click the mouse and select Format Cells) 2. Click [Customize], enter "Finance Department-@" in the [Type] option, and finally click [OK] to complete! 2. Plug-in method 1. Download and install the Excel plug-in Square Grid.
 Count the number of occurrences of a substring recursively in Java
Sep 17, 2023 pm 07:49 PM
Count the number of occurrences of a substring recursively in Java
Sep 17, 2023 pm 07:49 PM
Given two strings str_1 and str_2. The goal is to count the number of occurrences of substring str2 in string str1 using a recursive procedure. A recursive function is a function that calls itself within its definition. If str1 is "Iknowthatyouknowthatiknow" and str2 is "know" the number of occurrences is -3. Let us understand through examples. For example, input str1="TPisTPareTPamTP", str2="TP"; output Countofoccurrencesofasubstringrecursi
 How to use the Math.Pow function in C# to calculate the power of a specified number
Nov 18, 2023 am 11:32 AM
How to use the Math.Pow function in C# to calculate the power of a specified number
Nov 18, 2023 am 11:32 AM
In C#, there is a Math class library, which contains many mathematical functions. These include the function Math.Pow, which calculates powers, which can help us calculate the power of a specified number. The usage of the Math.Pow function is very simple, you only need to specify the base and exponent. The syntax is as follows: Math.Pow(base,exponent); where base represents the base and exponent represents the exponent. This function returns a double type result, that is, the power calculation result. Let's
 What is the magnet link prefix?
Feb 21, 2024 pm 08:45 PM
What is the magnet link prefix?
Feb 21, 2024 pm 08:45 PM
What is the magnet link prefix? Magnet links are a method for sharing files on the Internet. It has become the preferred way for many people to share and download resources. It allows users to easily get the files they need through a unified link. However, for those who are new to magnet links, some of the terms and concepts may be confusing. One of the common questions is, what is the magnet link prefix? Before answering this question, let us first understand the basic structure of magnet links. Magnet links consist of two parts: prefix and unique
 Java program to calculate the area of a triangle using determinants
Aug 31, 2023 am 10:17 AM
Java program to calculate the area of a triangle using determinants
Aug 31, 2023 am 10:17 AM
Introduction The Java program for calculating the area of a triangle using determinants is a concise and efficient program that can calculate the area of a triangle given the coordinates of three vertices. This program is useful for anyone learning or working with geometry, as it demonstrates how to use basic arithmetic and algebraic calculations in Java, as well as how to use the Scanner class to read user input. The program prompts the user for the coordinates of three points of the triangle, which are then read in and used to calculate the determinant of the coordinate matrix. Use the absolute value of the determinant to ensure the area is always positive, then use a formula to calculate the area of the triangle and display it to the user. The program can be easily modified to accept input in different formats or to perform additional calculations, making it a versatile tool for geometric calculations. ranks of determinants






