Reverse doubly linked list grouping by given size using C++

In this problem, we get a pointer to the head of the linked list and an integer k. In a group of size k, we need to reverse the linked list. For example -
Input : 1 <-> 2 <-> 3 <-> 4 <-> 5 (doubly linked list), k = 3 Output : 3 <-> 2 <-> 1 <-> 5 <-> 4
Methods to find solution
In this problem we will formulate a recursive algorithm to solve this problem. In this method we will use recursion and solve the problem using recursion.
Example
#include <iostream>
using namespace std;
struct Node {
int data;
Node *next, *prev;
};
// push function to push a node into the list
Node* push(Node* head, int data) {
Node* new_node = new Node();
new_node->data = data;
new_node->next = NULL;
Node* TMP = head;
if (head == NULL) {
new_node->prev = NULL;
head = new_node;
return head;
}
while (TMP->next != NULL) { // going to the last node
TMP = TMP->next;
}
TMP->next = new_node;
new_node->prev = TMP;
return head; // return pointer to head
}
// function to print given list
void printDLL(Node* head) {
while (head != NULL) {
cout << head->data << " ";
head = head->next;
}
cout << endl;
}
Node* revK(Node* head, int k) {
if (!head)
return NULL;
head->prev = NULL;
Node *TMP, *CURRENT = head, *newHead;
int count = 0;
while (CURRENT != NULL && count < k) { // while our count is less than k we simply reverse the nodes.
newHead = CURRENT;
TMP = CURRENT->prev;
CURRENT->prev = CURRENT->next;
CURRENT->next = TMP;
CURRENT = CURRENT->prev;
count++;
}
if (count >= k) {
head->next = revK(CURRENT, k); // now when if the count is greater or equal
//to k we connect first head to next head
}
return newHead;
}
int main() {
Node* head;
for (int i = 1; i <= 5; i++) {
head = push(head, i);
}
cout << "Original List : ";
printDLL(head);
cout << "\nModified List : ";
int k = 3;
head = revK(head, k);
printDLL(head);
}
Output
Original List : 1 2 3 4 5 Modified List : 3 2 1 5 4
Explanation of the above code
In this method, we loop through the list and iterate until the count is less than k. We make a recursive call giving the value to head -> next( Here we are just reversing the list while traversing, but when k is reached we need to make head point to the kth element of another list, for example if our list is 1 2 3 4 5, our k is 3, we reversed the middle element to be 3 2 1, but now we need 1 to point to 4 because that element will also be reversed, so that's why we use the recursive call and make additional if statements.).
Conclusion
In this article, we solved the problem using recursion. We also learned a C program for this problem and our complete approach to solving it. We can write the same program in other languages such as C, java, python and other languages. We hope this article was helpful to you.
The above is the detailed content of Reverse doubly linked list grouping by given size using C++. For more information, please follow other related articles on the PHP Chinese website!

Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

AI Hentai Generator
Generate AI Hentai for free.

Hot Article

Hot Tools

Notepad++7.3.1
Easy-to-use and free code editor

SublimeText3 Chinese version
Chinese version, very easy to use

Zend Studio 13.0.1
Powerful PHP integrated development environment

Dreamweaver CS6
Visual web development tools

SublimeText3 Mac version
God-level code editing software (SublimeText3)

Hot Topics
 1377
1377
 52
52
 Use C++ to write code to find the Nth non-square number
Aug 30, 2023 pm 10:41 PM
Use C++ to write code to find the Nth non-square number
Aug 30, 2023 pm 10:41 PM
We all know numbers that are not the square of any number, such as 2, 3, 5, 7, 8, etc. There are N non-square numbers, and it is impossible to know every number. So, in this article, we will explain everything about squareless or non-square numbers and ways to find the Nth non-square number in C++. Nth non-square number If a number is the square of an integer, then the number is called a perfect square. Some examples of perfect square numbers are -1issquareof14issquareof29issquareof316issquareof425issquareof5 If a number is not the square of any integer, then the number is called non-square. For example, the first 15 non-square numbers are -2,3,5,6,
 Inversion algorithm for right rotation of array written in C++
Sep 08, 2023 pm 08:17 PM
Inversion algorithm for right rotation of array written in C++
Sep 08, 2023 pm 08:17 PM
In this article, we will learn about the reversal algorithm to rotate a given array to the right by k elements, for example −Input:arr[]={4,6,2,6,43,7,3,7},k= 4Output:{43,7,3,7,4,6,2,6}Explanation:Rotatingeachelementofarrayby4-elementtotherightgives{43,7,3,7,4,6,2,6}.Input:arr[]={8 ,5,8,2,1,4,9,3},k=3Output:{4,9,3,8,5,8,2,1} Find the solution
 In C programming, find the area of a circle
Aug 25, 2023 pm 10:57 PM
In C programming, find the area of a circle
Aug 25, 2023 pm 10:57 PM
A circle is a closed figure. All points on a circle are equidistant from a point inside the circle. The center point is called the center of the circle. The distance from a point to the center of a circle is called the radius. Area is a quantitative representation of the span of dimensions of a closed figure. The area of a circle is the area enclosed within the dimensions of the circle. The formula to calculate the area of a circle, Area=π*r*r To calculate the area, we give the radius of the circle as input, we will use the formula to calculate the area, algorithm STEP1: Takeradiusasinputfromtheuserusingstdinput.STEP2: Calculatetheareaofcircleusing, area=(
 Find the number of unique pairs in an array using C++
Sep 07, 2023 am 11:53 AM
Find the number of unique pairs in an array using C++
Sep 07, 2023 am 11:53 AM
We need proper knowledge to create several unique pairs in array syntax of C++. While finding the number of unique pairs, we count all the unique pairs in the given array i.e. all possible pairs can be formed where each pair should be unique. For example -Input:array[]={5,5,9}Output:4Explanation:Thenumberoffalluniquepairsare(5,5),(5,9),(9,5)and(9,9).Input:array[]= {5,4,3,2,2}Output:16 Ways to Find Solution There are two ways to solve this problem, they are −
 Write a code using C++ to find the number of subarrays with the same minimum and maximum values
Aug 25, 2023 pm 11:33 PM
Write a code using C++ to find the number of subarrays with the same minimum and maximum values
Aug 25, 2023 pm 11:33 PM
In this article, we will use C++ to solve the problem of finding the number of subarrays whose maximum and minimum values are the same. The following is an example of the problem −Input:array={2,3,6,6,2,4,4,4}Output:12Explanation:{2},{3},{6},{6},{2 },{4},{4},{4},{6,6},{4,4},{4,4}and{4,4,4}arethesubarrayswhichcanbeformedwithmaximumandminimumelementsame.Input:array={3,3, 1,5,
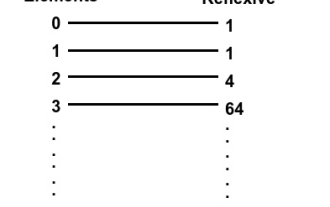 Written in C++, find the number of reflexive relations on a set
Aug 26, 2023 pm 08:17 PM
Written in C++, find the number of reflexive relations on a set
Aug 26, 2023 pm 08:17 PM
In this article we will explain ways to find reflexive relations on a set. In this problem, we are given a number n, and a set of n natural numbers, and we must determine the number of reflexive relations. Reflexive relation - A relation R is said to be a reflexive relation on the set A if for every 'a' in the set A, (a, a) belongs to the relation R. For example -Input:x=1Output:1Explanation:set={1},reflexiverelationsonA*A:{{1}}Input:x=2Output:4Explanation:set={1,2},reflexiverelationsonA*
 Reverse doubly linked list grouping by given size using C++
Sep 04, 2023 am 09:49 AM
Reverse doubly linked list grouping by given size using C++
Sep 04, 2023 am 09:49 AM
In this problem, we are given a pointer to the head of the linked list and an integer k. In a group of size k, we need to reverse the linked list. For example -Input:1<->2<->3<->4<->5(doublylinkedlist),k=3Output:3<->2<->1<->5<->4 looking for solutions Method In this problem, we will formulate a recursive algorithm to solve this problem. In this method we will use recursion and solve the problem using recursion. Example#include<iostream&
 Reversal algorithm for array rotation written in C++
Aug 28, 2023 pm 11:13 PM
Reversal algorithm for array rotation written in C++
Aug 28, 2023 pm 11:13 PM
In the given problem, we have an array and we need to rotate the array by d elements using inversion algorithm like −Input:arr[]=[1,2,3,4,5,6,7], d=2Output:arr[]=[3,4,5,6,7,1,2]Explanation:Asyoucanseewehavetorotatethisarraybyd=2butourmaintaskistoachievethisbyusingareversaltechnique. We performed some inversion technique calculations on the rotation of the array and concluded: First, we reverse




