 Software Tutorial
Software Tutorial
 Computer Software
Computer Software
 A graphic method for constructing the midpoint trajectory between a point on a circle and any point on the geometric sketchpad
A graphic method for constructing the midpoint trajectory between a point on a circle and any point on the geometric sketchpad
A graphic method for constructing the midpoint trajectory between a point on a circle and any point on the geometric sketchpad
Graphic method for constructing the midpoint trajectory between a point on a circle and any point on the geometric sketchpad In mathematics teaching, we often encounter the problem of constructing the midpoint trajectory of a point on a circle and any point on the geometric sketchpad. This question seems simple, but in fact it contains a profound connection between geometry and algebra. It is a classic question in geometry. This article will explore the solution to this problem from both theoretical and practical aspects, hoping to provide some ideas and methods for teachers and students. In the following content, we will first introduce the background and related knowledge of this problem from a theoretical level, and then combine it with specific examples to demonstrate how to use the geometric sketchpad to construct such a trajectory.
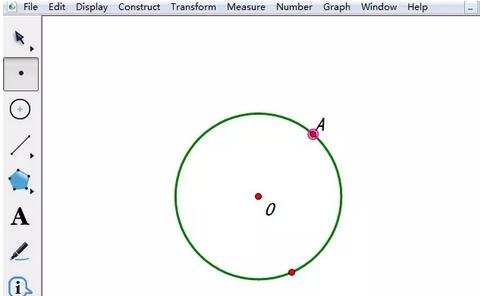
Construct a circle O and pick a point A on the circle. Open the geometric sketchpad, select the [Circle Tool] on the left sidebar and draw a circle O in the working area. Use the [Point Tool] to pick any point A on the circle.
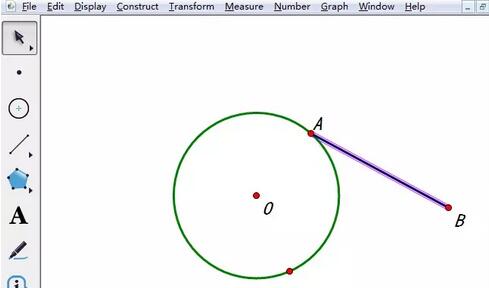
Pick any point B and construct line segment AB. Use the [Point Tool] to draw a point B arbitrarily in the blank area, then select points A and B in sequence, click the [Construct] menu on the upper menu bar, and select the [Line Segment] command from its drop-down option, thus constructing the line segment AB.
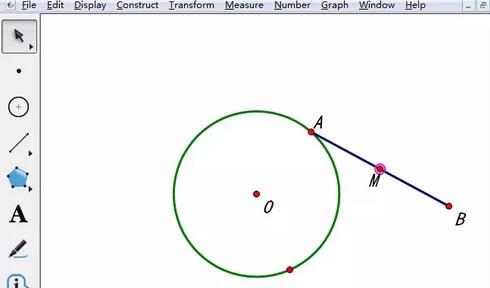
Construct the midpoint M of line segment AB. Use the move tool to select line segment AB, click the [Construction] menu on the upper menu bar, and select the [Midpoint] command from its drop-down option, thus obtaining the midpoint M of the line segment.
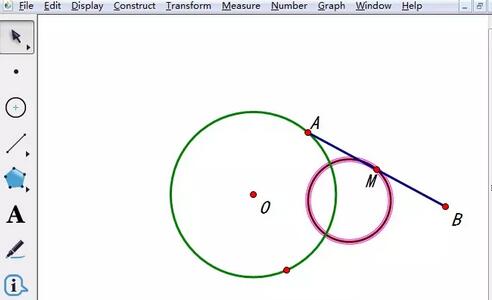
Construct the trajectory. Use the move tool to select point A and point M in sequence, click the [Construction] menu in the upper menu bar, and select the [Trajectory] command from the drop-down option. This way you will get the motion trajectory of midpoint M,
The above is the detailed content of A graphic method for constructing the midpoint trajectory between a point on a circle and any point on the geometric sketchpad. For more information, please follow other related articles on the PHP Chinese website!

Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

AI Hentai Generator
Generate AI Hentai for free.

Hot Article

Hot Tools

Notepad++7.3.1
Easy-to-use and free code editor

SublimeText3 Chinese version
Chinese version, very easy to use

Zend Studio 13.0.1
Powerful PHP integrated development environment

Dreamweaver CS6
Visual web development tools

SublimeText3 Mac version
God-level code editing software (SublimeText3)

Hot Topics
 how to unlink rockstar account from steam
Mar 11, 2025 pm 07:39 PM
how to unlink rockstar account from steam
Mar 11, 2025 pm 07:39 PM
This article explains how to unlink a Rockstar Games Social Club account from Steam. The process involves using the Rockstar Games Launcher to manage linked accounts, removing the Steam connection without impacting game progress or future Steam purc
 why is steam downloading so slow
Mar 11, 2025 pm 07:36 PM
why is steam downloading so slow
Mar 11, 2025 pm 07:36 PM
Slow Steam downloads stem from various factors: network congestion (home or ISP), Steam/game server issues, limited bandwidth, high latency, and computer hardware limitations. Troubleshooting involves checking internet speed, optimizing Steam settin
![[PROVEN] Steam Error e87 Fix: Get Gaming Again in Minutes!](https://img.php.cn/upload/article/202503/18/2025031817560457401.jpg?x-oss-process=image/resize,m_fill,h_207,w_330) [PROVEN] Steam Error e87 Fix: Get Gaming Again in Minutes!
Mar 18, 2025 pm 05:56 PM
[PROVEN] Steam Error e87 Fix: Get Gaming Again in Minutes!
Mar 18, 2025 pm 05:56 PM
Article discusses causes of Steam Error e87, including network issues, security software, server problems, outdated clients, and corrupted files. Offers prevention and solution strategies.[159 characters]
 Steam Error e87: What It Is & How to Fix It
Mar 18, 2025 pm 05:51 PM
Steam Error e87: What It Is & How to Fix It
Mar 18, 2025 pm 05:51 PM
Steam Error e87 occurs during Steam client updates or launches due to connection issues. Fix it by restarting devices, checking server status, changing DNS, disabling security software, clearing cache, or reinstalling Steam.
 Steam Error e87: Why It Happens & 5 Ways to Fix It
Mar 18, 2025 pm 05:55 PM
Steam Error e87: Why It Happens & 5 Ways to Fix It
Mar 18, 2025 pm 05:55 PM
Steam Error e87 disrupts gaming on Steam due to connectivity issues. The article discusses causes like unstable internet and server overload, and offers fixes like restarting Steam and checking for updates.
 How to Fix Steam Error Code e87: The ULTIMATE Guide
Mar 18, 2025 pm 05:51 PM
How to Fix Steam Error Code e87: The ULTIMATE Guide
Mar 18, 2025 pm 05:51 PM
Article discusses fixing Steam Error Code e87, caused by network issues, corrupt files, or client problems. Provides troubleshooting steps and prevention tips.
 Easy Fix: Steam Error e87 Explained & Solved
Mar 18, 2025 pm 05:53 PM
Easy Fix: Steam Error e87 Explained & Solved
Mar 18, 2025 pm 05:53 PM
Steam Error e87, caused by connectivity issues, can be fixed without reinstalling by restarting, checking internet, and clearing cache. Adjusting Steam settings helps prevent future occurrences.
 how to add page numbers in google docs
Mar 14, 2025 pm 02:57 PM
how to add page numbers in google docs
Mar 14, 2025 pm 02:57 PM
The article details how to add, customize, start from a specific page, and remove page numbers in Google Docs using step-by-step instructions.





