The operation content of drawing ellipse parametric equations on the geometric sketchpad

Geometric Sketchpad is a powerful geometric drawing software that can not only draw various plane graphics, but also some common spatial graphics, and can also draw some dynamically changing graphics. Today, PHP editor Xinyi will share with you a technique for drawing ellipses using the geometric sketchpad. An ellipse is a type of conic section, and its center is not necessarily at the origin. Therefore, when we draw an ellipse, we need to know the coordinates of its center, the length of its semi-major axis and its semi-minor axis. Next, PHP editor Xinyi will introduce in detail how to use the geometric sketchpad to draw an ellipse.

1. Open the geometric sketchpad, execute the [Data]-[New Function] command, and create two new functions: f(x)=6cos(x), g(x)=3sin(x), on the sketchpad Two function expressions appear on.

2. Execute the [Draw]-[Draw Parametric Curve] command to pop up the curve drawing dialog box. In (x, y) mode, click the function f(x)= in sequence. 6cos(x), g(x)=3sin(x), enter the appropriate value in the [Definition Domain].

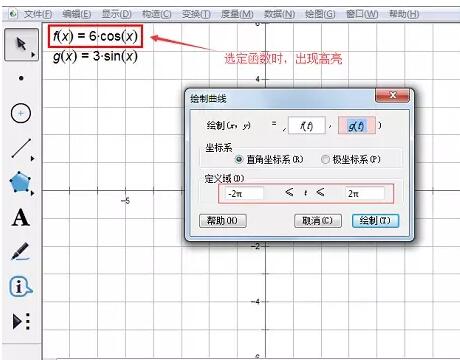
Note: The function can only be selected when it is highlighted. If it is not highlighted, just click the input box with the mouse, or click [Draw Parameter Curve] Before, select f(x)=6cos(x), g(x)=3sin(x), and then select (x, y) mode.

3. Drag the mouse on the graphic on the drawing board to get the parametric equation image as shown in the figure.

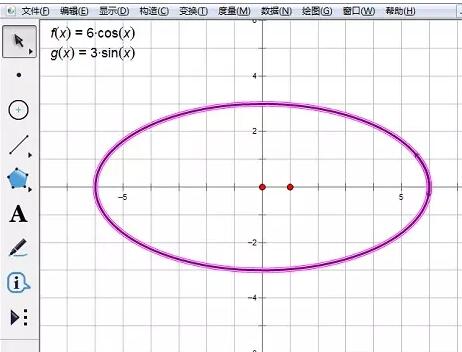
The above is the detailed content of The operation content of drawing ellipse parametric equations on the geometric sketchpad. For more information, please follow other related articles on the PHP Chinese website!