PHP+JS+rsa数据加密传输实现代码_PHP教程
JS端代码:
//文件base64.js:
var b64map="ABCDEFGHIJKLMNOPQRSTUVWXYZabcdefghijklmnopqrstuvwxyz0123456789+/";
var b64pad="=";
function hex2b64(h) {
var i;
var c;
var ret = "";
for(i = 0; i+3 c = parseInt(h.substring(i,i+3),16);
ret += b64map.charAt(c >> 6) + b64map.charAt(c & 63);
}
if(i+1 == h.length) {
c = parseInt(h.substring(i,i+1),16);
ret += b64map.charAt(c }
else if(i+2 == h.length) {
c = parseInt(h.substring(i,i+2),16);
ret += b64map.charAt(c >> 2) + b64map.charAt((c & 3) }
while((ret.length & 3) > 0) ret += b64pad;
return ret;
}
// convert a base64 string to hex
function b64tohex(s) {
var ret = ""
var i;
var k = 0; // b64 state, 0-3
var slop;
for(i = 0; i if(s.charAt(i) == b64pad) break;
v = b64map.indexOf(s.charAt(i));
if(v if(k == 0) {
ret += int2char(v >> 2);
slop = v & 3;
k = 1;
}
else if(k == 1) {
ret += int2char((slop > 4));
slop = v & 0xf;
k = 2;
}
else if(k == 2) {
ret += int2char(slop);
ret += int2char(v >> 2);
slop = v & 3;
k = 3;
}
else {
ret += int2char((slop > 4));
ret += int2char(v & 0xf);
k = 0;
}
}
if(k == 1)
ret += int2char(slop return ret;
}
// convert a base64 string to a byte/number array
function b64toBA(s) {
//piggyback on b64tohex for now, optimize later
var h = b64tohex(s);
var i;
var a = new Array();
for(i = 0; 2*i a[i] = parseInt(h.substring(2*i,2*i+2),16);
}
return a;
}
#文件jsbn.js
// Copyright (c) 2005 Tom Wu
// All Rights Reserved.
// See "LICENSE" for details.
// Basic JavaScript BN library - subset useful for RSA encryption.
// Bits per digit
var dbits;
// JavaScript engine analysis
var canary = 0xdeadbeefcafe;
var j_lm = ((canary&0xffffff)==0xefcafe);
// (public) Constructor
function BigInteger(a,b,c) {
if(a != null)
if("number" == typeof a) this.fromNumber(a,b,c);
else if(b == null && "string" != typeof a) this.fromString(a,256);
else this.fromString(a,b);
}
// return new, unset BigInteger
function nbi() { return new BigInteger(null); }
// am: Compute w_j += (x*this_i), propagate carries,
// c is initial carry, returns final carry.
// c // We need to select the fastest one that works in this environment.
// am1: use a single mult and divide to get the high bits,
// max digit bits should be 26 because
// max internal value = 2*dvalue^2-2*dvalue (function am1(i,x,w,j,c,n) {
while(--n >= 0) {
var v = x*this[i++]+w[j]+c;
c = Math.floor(v/0x4000000);
w[j++] = v&0x3ffffff;
}
return c;
}
// am2 avoids a big mult-and-extract completely.
// Max digit bits should be // on values up to 2*hdvalue^2-hdvalue-1 (function am2(i,x,w,j,c,n) {
var xl = x&0x7fff, xh = x>>15;
while(--n >= 0) {
var l = this[i]&0x7fff;
var h = this[i++]>>15;
var m = xh*l+h*xl;
l = xl*l+((m&0x7fff)c = (l>>>30)+(m>>>15)+xh*h+(c>>>30);
w[j++] = l&0x3fffffff;
}
return c;
}
// Alternately, set max digit bits to 28 since some
// browsers slow down when dealing with 32-bit numbers.
function am3(i,x,w,j,c,n) {
var xl = x&0x3fff, xh = x>>14;
while(--n >= 0) {
var l = this[i]&0x3fff;
var h = this[i++]>>14;
var m = xh*l+h*xl;
l = xl*l+((m&0x3fff)c = (l>>28)+(m>>14)+xh*h;
w[j++] = l&0xfffffff;
}
return c;
}
if(j_lm && (navigator.appName == "Microsoft Internet Explorer")) {
BigInteger.prototype.am = am2;
dbits = 30;
}
else if(j_lm && (navigator.appName != "Netscape")) {
BigInteger.prototype.am = am1;
dbits = 26;
}
else { // Mozilla/Netscape seems to prefer am3
BigInteger.prototype.am = am3;
dbits = 28;
}
BigInteger.prototype.DB = dbits;
BigInteger.prototype.DM = ((1BigInteger.prototype.DV = (1var BI_FP = 52;
BigInteger.prototype.FV = Math.pow(2,BI_FP);
BigInteger.prototype.F1 = BI_FP-dbits;
BigInteger.prototype.F2 = 2*dbits-BI_FP;
// Digit conversions
var BI_RM = "0123456789abcdefghijklmnopqrstuvwxyz";
var BI_RC = new Array();
var rr,vv;
rr = "0".charCodeAt(0);
for(vv = 0; vv rr = "a".charCodeAt(0);
for(vv = 10; vv rr = "A".charCodeAt(0);
for(vv = 10; vv function int2char(n) { return BI_RM.charAt(n); }
function intAt(s,i) {
var c = BI_RC[s.charCodeAt(i)];
return (c==null)?-1:c;
}
// (protected) copy this to r
function bnpCopyTo(r) {
for(var i = this.t-1; i >= 0; --i) r[i] = this[i];
r.t = this.t;
r.s = this.s;
}
// (protected) set from integer value x, -DV function bnpFromInt(x) {
this.t = 1;
this.s = (xif(x > 0) this[0] = x;
else if(x else this.t = 0;
}
// return bigint initialized to value
function nbv(i) { var r = nbi(); r.fromInt(i); return r; }
// (protected) set from string and radix
function bnpFromString(s,b) {
var k;
if(b == 16) k = 4;
else if(b == 8) k = 3;
else if(b == 256) k = 8; // byte array
else if(b == 2) k = 1;
else if(b == 32) k = 5;
else if(b == 4) k = 2;
else { this.fromRadix(s,b); return; }
this.t = 0;
this.s = 0;
var i = s.length, mi = false, sh = 0;
while(--i >= 0) {
var x = (k==8)?s[i]&0xff:intAt(s,i);
if(x if(s.charAt(i) == "-") mi = true;
continue;
}
mi = false;
if(sh == 0)
this[this.t++] = x;
else if(sh+k > this.DB) {
this[this.t-1] |= (x&((1this[this.t++] = (x>>(this.DB-sh));
}
else
this[this.t-1] |= xsh += k;
if(sh >= this.DB) sh -= this.DB;
}
if(k == 8 && (s[0]&0x80) != 0) {
this.s = -1;
if(sh > 0) this[this.t-1] |= ((1}
this.clamp();
if(mi) BigInteger.ZERO.subTo(this,this);
}
// (protected) clamp off excess high words
function bnpClamp() {
var c = this.s&this.DM;
while(this.t > 0 && this[this.t-1] == c) --this.t;
}
// (public) return string representation in given radix
function bnToString(b) {
if(this.s var k;
if(b == 16) k = 4;
else if(b == 8) k = 3;
else if(b == 2) k = 1;
else if(b == 32) k = 5;
else if(b == 4) k = 2;
else return this.toRadix(b);
var km = (1var p = this.DB-(i*this.DB)%k;
if(i-- > 0) {
if(p >p) > 0) { m = true; r = int2char(d); }
while(i >= 0) {
if(p d = (this[i]&((1d |= this[--i]>>(p+=this.DB-k);
}
else {
d = (this[i]>>(p-=k))&km;
if(p }
if(d > 0) m = true;
if(m) r += int2char(d);
}
}
return m?r:"0";
}
// (public) -this
function bnNegate() { var r = nbi(); BigInteger.ZERO.subTo(this,r); return r; }
// (public) |this|
function bnAbs() { return (this.s// (public) return + if this > a, - if this function bnCompareTo(a) {
var r = this.s-a.s;
if(r != 0) return r;
var i = this.t;
r = i-a.t;
if(r != 0) return r;
while(--i >= 0) if((r=this[i]-a[i]) != 0) return r;
return 0;
}
// returns bit length of the integer x
function nbits(x) {
var r = 1, t;
if((t=x>>>16) != 0) { x = t; r += 16; }
if((t=x>>8) != 0) { x = t; r += 8; }
if((t=x>>4) != 0) { x = t; r += 4; }
if((t=x>>2) != 0) { x = t; r += 2; }
if((t=x>>1) != 0) { x = t; r += 1; }
return r;
}
// (public) return the number of bits in "this"
function bnBitLength() {
if(this.t return this.DB*(this.t-1)+nbits(this[this.t-1]^(this.s&this.DM));
}
// (protected) r = this function bnpDLShiftTo(n,r) {
var i;
for(i = this.t-1; i >= 0; --i) r[i+n] = this[i];
for(i = n-1; i >= 0; --i) r[i] = 0;
r.t = this.t+n;
r.s = this.s;
}
// (protected) r = this >> n*DB
function bnpDRShiftTo(n,r) {
for(var i = n; i r.t = Math.max(this.t-n,0);
r.s = this.s;
}
// (protected) r = this function bnpLShiftTo(n,r) {
var bs = n%this.DB;
var cbs = this.DB-bs;
var bm = (1var ds = Math.floor(n/this.DB), c = (this.sfor(i = this.t-1; i >= 0; --i) {
r[i+ds+1] = (this[i]>>cbs)|c;
c = (this[i]&bm)}
for(i = ds-1; i >= 0; --i) r[i] = 0;
r[ds] = c;
r.t = this.t+ds+1;
r.s = this.s;
r.clamp();
}
// (protected) r = this >> n
function bnpRShiftTo(n,r) {
r.s = this.s;
var ds = Math.floor(n/this.DB);
if(ds >= this.t) { r.t = 0; return; }
var bs = n%this.DB;
var cbs = this.DB-bs;
var bm = (1r[0] = this[ds]>>bs;
for(var i = ds+1; i r[i-ds-1] |= (this[i]&bm)r[i-ds] = this[i]>>bs;
}
if(bs > 0) r[this.t-ds-1] |= (this.s&bm)r.t = this.t-ds;
r.clamp();
}
// (protected) r = this - a
function bnpSubTo(a,r) {
var i = 0, c = 0, m = Math.min(a.t,this.t);
while(i c += this[i]-a[i];
r[i++] = c&this.DM;
c >>= this.DB;
}
if(a.t c -= a.s;
while(i c += this[i];
r[i++] = c&this.DM;
c >>= this.DB;
}
c += this.s;
}
else {
c += this.s;
while(i c -= a[i];
r[i++] = c&this.DM;
c >>= this.DB;
}
c -= a.s;
}
r.s = (cif(c else if(c > 0) r[i++] = c;
r.t = i;
r.clamp();
}
// (protected) r = this * a, r != this,a (HAC 14.12)
// "this" should be the larger one if appropriate.
function bnpMultiplyTo(a,r) {
var x = this.abs(), y = a.abs();
var i = x.t;
r.t = i+y.t;
while(--i >= 0) r[i] = 0;
for(i = 0; i r.s = 0;
r.clamp();
if(this.s != a.s) BigInteger.ZERO.subTo(r,r);
}
// (protected) r = this^2, r != this (HAC 14.16)
function bnpSquareTo(r) {
var x = this.abs();
var i = r.t = 2*x.t;
while(--i >= 0) r[i] = 0;
for(i = 0; i var c = x.am(i,x[i],r,2*i,0,1);
if((r[i+x.t]+=x.am(i+1,2*x[i],r,2*i+1,c,x.t-i-1)) >= x.DV) {
r[i+x.t] -= x.DV;
r[i+x.t+1] = 1;
}
}
if(r.t > 0) r[r.t-1] += x.am(i,x[i],r,2*i,0,1);
r.s = 0;
r.clamp();
}
// (protected) divide this by m, quotient and remainder to q, r (HAC 14.20)
// r != q, this != m. q or r may be null.
function bnpDivRemTo(m,q,r) {
var pm = m.abs();
if(pm.t var pt = this.abs();
if(pt.t if(q != null) q.fromInt(0);
if(r != null) this.copyTo(r);
return;
}
if(r == null) r = nbi();
var y = nbi(), ts = this.s, ms = m.s;
var nsh = this.DB-nbits(pm[pm.t-1]); // normalize modulus
if(nsh > 0) { pm.lShiftTo(nsh,y); pt.lShiftTo(nsh,r); }
else { pm.copyTo(y); pt.copyTo(r); }
var ys = y.t;
var y0 = y[ys-1];
if(y0 == 0) return;
var yt = y0*(11)?y[ys-2]>>this.F2:0);
var d1 = this.FV/yt, d2 = (1var i = r.t, j = i-ys, t = (q==null)?nbi():q;
y.dlShiftTo(j,t);
if(r.compareTo(t) >= 0) {
r[r.t++] = 1;
r.subTo(t,r);
}
BigInteger.ONE.dlShiftTo(ys,t);
t.subTo(y,y); // "negative" y so we can replace sub with am later
while(y.t while(--j >= 0) {
// Estimate quotient digit
var qd = (r[--i]==y0)?this.DM:Math.floor(r[i]*d1+(r[i-1]+e)*d2);
if((r[i]+=y.am(0,qd,r,j,0,ys)) y.dlShiftTo(j,t);
r.subTo(t,r);
while(r[i] }
}
if(q != null) {
r.drShiftTo(ys,q);
if(ts != ms) BigInteger.ZERO.subTo(q,q);
}
r.t = ys;
r.clamp();
if(nsh > 0) r.rShiftTo(nsh,r); // Denormalize remainder
if(ts }
// (public) this mod a
function bnMod(a) {
var r = nbi();
this.abs().divRemTo(a,null,r);
if(this.s 0) a.subTo(r,r);
return r;
}
// Modular reduction using "classic" algorithm
function Classic(m) { this.m = m; }
function cConvert(x) {
if(x.s = 0) return x.mod(this.m);
else return x;
}
function cRevert(x) { return x; }
function cReduce(x) { x.divRemTo(this.m,null,x); }
function cMulTo(x,y,r) { x.multiplyTo(y,r); this.reduce(r); }
function cSqrTo(x,r) { x.squareTo(r); this.reduce(r); }
Classic.prototype.convert = cConvert;
Classic.prototype.revert = cRevert;
Classic.prototype.reduce = cReduce;
Classic.prototype.mulTo = cMulTo;
Classic.prototype.sqrTo = cSqrTo;
// (protected) return "-1/this % 2^DB"; useful for Mont. reduction
// justification:
// xy == 1 (mod m)
// xy = 1+km
// xy(2-xy) = (1+km)(1-km)
// x[y(2-xy)] = 1-k^2m^2
// x[y(2-xy)] == 1 (mod m^2)
// if y is 1/x mod m, then y(2-xy) is 1/x mod m^2
// should reduce x and y(2-xy) by m^2 at each step to keep size bounded.
// JS multiply "overflows" differently from C/C++, so care is needed here.
function bnpInvDigit() {
if(this.t var x = this[0];
if((x&1) == 0) return 0;
var y = x&3; // y == 1/x mod 2^2
y = (y*(2-(x&0xf)*y))&0xf; // y == 1/x mod 2^4
y = (y*(2-(x&0xff)*y))&0xff; // y == 1/x mod 2^8
y = (y*(2-(((x&0xffff)*y)&0xffff)))&0xffff; // y == 1/x mod 2^16
// last step - calculate inverse mod DV directly;
// assumes 16 y = (y*(2-x*y%this.DV))%this.DV; // y == 1/x mod 2^dbits
// we really want the negative inverse, and -DV return (y>0)?this.DV-y:-y;
}
// Montgomery reduction
function Montgomery(m) {
this.m = m;
this.mp = m.invDigit();
this.mpl = this.mp&0x7fff;
this.mph = this.mp>>15;
this.um = (1this.mt2 = 2*m.t;
}
// xR mod m
function montConvert(x) {
var r = nbi();
x.abs().dlShiftTo(this.m.t,r);
r.divRemTo(this.m,null,r);
if(x.s 0) this.m.subTo(r,r);
return r;
}
// x/R mod m
function montRevert(x) {
var r = nbi();
x.copyTo(r);
this.reduce(r);
return r;
}
// x = x/R mod m (HAC 14.32)
function montReduce(x) {
while(x.t x[x.t++] = 0;
for(var i = 0; i // faster way of calculating u0 = x[i]*mp mod DV
var j = x[i]&0x7fff;
var u0 = (j*this.mpl+(((j*this.mph+(x[i]>>15)*this.mpl)&this.um)// use am to combine the multiply-shift-add into one call
j = i+this.m.t;
x[j] += this.m.am(0,u0,x,i,0,this.m.t);
// propagate carry
while(x[j] >= x.DV) { x[j] -= x.DV; x[++j]++; }
}
x.clamp();
x.drShiftTo(this.m.t,x);
if(x.compareTo(this.m) >= 0) x.subTo(this.m,x);
}
// r = "x^2/R mod m"; x != r
function montSqrTo(x,r) { x.squareTo(r); this.reduce(r); }
// r = "xy/R mod m"; x,y != r
function montMulTo(x,y,r) { x.multiplyTo(y,r); this.reduce(r); }
Montgomery.prototype.convert = montConvert;
Montgomery.prototype.revert = montRevert;
Montgomery.prototype.reduce = montReduce;
Montgomery.prototype.mulTo = montMulTo;
Montgomery.prototype.sqrTo = montSqrTo;
// (protected) true iff this is even
function bnpIsEven() { return ((this.t>0)?(this[0]&1):this.s) == 0; }
// (protected) this^e, e function bnpExp(e,z) {
if(e > 0xffffffff || e var r = nbi(), r2 = nbi(), g = z.convert(this), i = nbits(e)-1;
g.copyTo(r);
while(--i >= 0) {
z.sqrTo(r,r2);
if((e&(1 0) z.mulTo(r2,g,r);
else { var t = r; r = r2; r2 = t; }
}
return z.revert(r);
}
// (public) this^e % m, 0 function bnModPowInt(e,m) {
var z;
if(e return this.exp(e,z);
}
// protected
BigInteger.prototype.copyTo = bnpCopyTo;
BigInteger.prototype.fromInt = bnpFromInt;
BigInteger.prototype.fromString = bnpFromString;
BigInteger.prototype.clamp = bnpClamp;
BigInteger.prototype.dlShiftTo = bnpDLShiftTo;
BigInteger.prototype.drShiftTo = bnpDRShiftTo;
BigInteger.prototype.lShiftTo = bnpLShiftTo;
BigInteger.prototype.rShiftTo = bnpRShiftTo;
BigInteger.prototype.subTo = bnpSubTo;
BigInteger.prototype.multiplyTo = bnpMultiplyTo;
BigInteger.prototype.squareTo = bnpSquareTo;
BigInteger.prototype.divRemTo = bnpDivRemTo;
BigInteger.prototype.invDigit = bnpInvDigit;
BigInteger.prototype.isEven = bnpIsEven;
BigInteger.prototype.exp = bnpExp;
// public
BigInteger.prototype.toString = bnToString;
BigInteger.prototype.negate = bnNegate;
BigInteger.prototype.abs = bnAbs;
BigInteger.prototype.compareTo = bnCompareTo;
BigInteger.prototype.bitLength = bnBitLength;
BigInteger.prototype.mod = bnMod;
BigInteger.prototype.modPowInt = bnModPowInt;
// "constants"
BigInteger.ZERO = nbv(0);
BigInteger.ONE = nbv(1);
#文件prng4.js
// prng4.js - uses Arcfour as a PRNG
function Arcfour() {
this.i = 0;
this.j = 0;
this.S = new Array();
}
// Initialize arcfour context from key, an array of ints, each from [0..255]
function ARC4init(key) {
var i, j, t;
for(i = 0; i this.S[i] = i;
j = 0;
for(i = 0; i j = (j + this.S[i] + key[i % key.length]) & 255;
t = this.S[i];
this.S[i] = this.S[j];
this.S[j] = t;
}
this.i = 0;
this.j = 0;
}
function ARC4next() {
var t;
this.i = (this.i + 1) & 255;
this.j = (this.j + this.S[this.i]) & 255;
t = this.S[this.i];
this.S[this.i] = this.S[this.j];
this.S[this.j] = t;
return this.S[(t + this.S[this.i]) & 255];
}
Arcfour.prototype.init = ARC4init;
Arcfour.prototype.next = ARC4next;
// Plug in your RNG constructor here
function prng_newstate() {
return new Arcfour();
}
// Pool size must be a multiple of 4 and greater than 32.
// An array of bytes the size of the pool will be passed to init()
var rng_psize = 256;
文件:rng.js
// Random number generator - requires a PRNG backend, e.g. prng4.js
// For best results, put code like
//
// in your main HTML document.
var rng_state;
var rng_pool;
var rng_pptr;
// Mix in a 32-bit integer into the pool
function rng_seed_int(x) {
rng_pool[rng_pptr++] ^= x & 255;
rng_pool[rng_pptr++] ^= (x >> 8) & 255;
rng_pool[rng_pptr++] ^= (x >> 16) & 255;
rng_pool[rng_pptr++] ^= (x >> 24) & 255;
if(rng_pptr >= rng_psize) rng_pptr -= rng_psize;
}
// Mix in the current time (w/milliseconds) into the pool
function rng_seed_time() {
rng_seed_int(new Date().getTime());
}
// Initialize the pool with junk if needed.
if(rng_pool == null) {
rng_pool = new Array();
rng_pptr = 0;
var t;
if(navigator.appName == "Netscape" && navigator.appVersion // Extract entropy (256 bits) from NS4 RNG if available
var z = window.crypto.random(32);
for(t = 0; t rng_pool[rng_pptr++] = z.charCodeAt(t) & 255;
}
while(rng_pptr t = Math.floor(65536 * Math.random());
rng_pool[rng_pptr++] = t >>> 8;
rng_pool[rng_pptr++] = t & 255;
}
rng_pptr = 0;
rng_seed_time();
//rng_seed_int(window.screenX);
//rng_seed_int(window.screenY);
}
function rng_get_byte() {
if(rng_state == null) {
rng_seed_time();
rng_state = prng_newstate();
rng_state.init(rng_pool);
for(rng_pptr = 0; rng_pptr rng_pool[rng_pptr] = 0;
rng_pptr = 0;
//rng_pool = null;
}
// TODO: allow reseeding after first request
return rng_state.next();
}
function rng_get_bytes(ba) {
var i;
for(i = 0; i }
function SecureRandom() {}
SecureRandom.prototype.nextBytes = rng_get_bytes;
#文件:rsa.js
// Depends on jsbn.js and rng.js
// Version 1.1: support utf-8 encoding in pkcs1pad2
// convert a (hex) string to a bignum object
function parseBigInt(str,r) {
return new BigInteger(str,r);
}
function linebrk(s,n) {
var ret = "";
var i = 0;
while(i + n ret += s.substring(i,i+n) + "\n";
i += n;
}
return ret + s.substring(i,s.length);
}
function byte2Hex(b) {
if(b return "0" + b.toString(16);
else
return b.toString(16);
}
// PKCS#1 (type 2, random) pad input string s to n bytes, and return a bigint
function pkcs1pad2(s,n) {
if(n alert("Message too long for RSA");
return null;
}
var ba = new Array();
var i = s.length - 1;
while(i >= 0 && n > 0) {
var c = s.charCodeAt(i--);
if(c ba[--n] = c;
}
else if((c > 127) && (c ba[--n] = (c & 63) | 128;
ba[--n] = (c >> 6) | 192;
}
else {
ba[--n] = (c & 63) | 128;
ba[--n] = ((c >> 6) & 63) | 128;
ba[--n] = (c >> 12) | 224;
}
}
ba[--n] = 0;
var rng = new SecureRandom();
var x = new Array();
while(n > 2) { // random non-zero pad
x[0] = 0;
while(x[0] == 0) rng.nextBytes(x);
ba[--n] = x[0];
}
ba[--n] = 2;
ba[--n] = 0;
return new BigInteger(ba);
}
// "empty" RSA key constructor
function RSAKey() {
this.n = null;
this.e = 0;
this.d = null;
this.p = null;
this.q = null;
this.dmp1 = null;
this.dmq1 = null;
this.coeff = null;
}
// Set the public key fields N and e from hex strings
function RSASetPublic(N,E) {
if(N != null && E != null && N.length > 0 && E.length > 0) {
this.n = parseBigInt(N,16);
this.e = parseInt(E,16);
}
else
alert("Invalid RSA public key");
}
// Perform raw public operation on "x": return x^e (mod n)
function RSADoPublic(x) {
return x.modPowInt(this.e, this.n);
}
// Return the PKCS#1 RSA encryption of "text" as an even-length hex string
function RSAEncrypt(text) {
var m = pkcs1pad2(text,(this.n.bitLength()+7)>>3);
if(m == null) return null;
var c = this.doPublic(m);
if(c == null) return null;
var h = c.toString(16);
if((h.length & 1) == 0) return h; else return "0" + h;
}
// Return the PKCS#1 RSA encryption of "text" as a Base64-encoded string
//function RSAEncryptB64(text) {
// var h = this.encrypt(text);
// if(h) return hex2b64(h); else return null;
//}
// protected
RSAKey.prototype.doPublic = RSADoPublic;
// public
RSAKey.prototype.setPublic = RSASetPublic;
RSAKey.prototype.encrypt = RSAEncrypt;
//RSAKey.prototype.encrypt_b64 = RSAEncryptB64;
HTML代码部分:
后端PHP部分:
RSA库:
/*
* PHP implementation of the RSA algorithm
* (C) Copyright 2004 Edsko de Vries, Ireland
*
* Licensed under the GNU Public License (GPL)
*
* This implementation has been verified against [3]
* (tested Java/PHP interoperability).
*
* References:
* [1] "Applied Cryptography", Bruce Schneier, John Wiley & Sons, 1996
* [2] "Prime Number Hide-and-Seek", Brian Raiter, Muppetlabs (online)
* [3] "The Bouncy Castle Crypto Package", Legion of the Bouncy Castle,
* (open source cryptography library for Java, online)
* [4] "PKCS #1: RSA Encryption Standard", RSA Laboratories Technical Note,
* version 1.5, revised November 1, 1993
*/
/*
* Functions that are meant to be used by the user of this PHP module.
*
* Notes:
* - $key and $modulus should be numbers in (decimal) string format
* - $message is expected to be binary data
* - $keylength should be a multiple of 8, and should be in bits
* - For rsa_encrypt/rsa_sign, the length of $message should not exceed
* ($keylength / 8) - 11 (as mandated by [4]).
* - rsa_encrypt and rsa_sign will automatically add padding to the message.
* For rsa_encrypt, this padding will consist of random values; for rsa_sign,
* padding will consist of the appropriate number of 0xFF values (see [4])
* - rsa_decrypt and rsa_verify will automatically remove message padding.
* - Blocks for decoding (rsa_decrypt, rsa_verify) should be exactly
* ($keylength / 8) bytes long.
* - rsa_encrypt and rsa_verify expect a public key; rsa_decrypt and rsa_sign
* expect a private key.
*/
/**
* 于2010-11-12 1:06分于LONELY修改
*/
function rsa_encrypt($message, $public_key, $modulus, $keylength)
{
$padded = add_PKCS1_padding($message, true, $keylength / 8);
$number = binary_to_number($padded);
$encrypted = pow_mod($number, $public_key, $modulus);
$result = number_to_binary($encrypted, $keylength / 8);
return $result;
}
function rsa_decrypt($message, $private_key, $modulus, $keylength)
{
$number = binary_to_number($message);
$decrypted = pow_mod($number, $private_key, $modulus);
$result = number_to_binary($decrypted, $keylength / 8);
return remove_PKCS1_padding($result, $keylength / 8);
}
function rsa_sign($message, $private_key, $modulus, $keylength)
{
$padded = add_PKCS1_padding($message, false, $keylength / 8);
$number = binary_to_number($padded);
$signed = pow_mod($number, $private_key, $modulus);
$result = number_to_binary($signed, $keylength / 8);
return $result;
}
function rsa_verify($message, $public_key, $modulus, $keylength)
{
return rsa_decrypt($message, $public_key, $modulus, $keylength);
}
function rsa_kyp_verify($message, $public_key, $modulus, $keylength)
{
$number = binary_to_number($message);
$decrypted = pow_mod($number, $public_key, $modulus);
$result = number_to_binary($decrypted, $keylength / 8);
return remove_KYP_padding($result, $keylength / 8);
}
/*
* Some constants
*/
define("BCCOMP_LARGER", 1);
/*
* The actual implementation.
* Requires BCMath support in PHP (compile with --enable-bcmath)
*/
//--
// Calculate (p ^ q) mod r
//
// We need some trickery to [2]:
// (a) Avoid calculating (p ^ q) before (p ^ q) mod r, because for typical RSA
// applications, (p ^ q) is going to be _WAY_ too large.
// (I mean, __WAY__ too large - won't fit in your computer's memory.)
// (b) Still be reasonably efficient.
//
// We assume p, q and r are all positive, and that r is non-zero.
//
// Note that the more simple algorithm of multiplying $p by itself $q times, and
// applying "mod $r" at every step is also valid, but is O($q), whereas this
// algorithm is O(log $q). Big difference.
//
// As far as I can see, the algorithm I use is optimal; there is no redundancy
// in the calculation of the partial results.
//--
function pow_mod($p, $q, $r)
{
// Extract powers of 2 from $q
$factors = array();
$div = $q;
$power_of_two = 0;
while(bccomp($div, "0") == BCCOMP_LARGER)
{
$rem = bcmod($div, 2);
$div = bcdiv($div, 2);
if($rem) array_push($factors, $power_of_two);
$power_of_two++;
}
// Calculate partial results for each factor, using each partial result as a
// starting point for the next. This depends of the factors of two being
// generated in increasing order.
$partial_results = array();
$part_res = $p;
$idx = 0;
foreach($factors as $factor)
{
while($idx {
$part_res = bcpow($part_res, "2");
$part_res = bcmod($part_res, $r);
$idx++;
}
array_push($partial_results, $part_res);
}
// Calculate final result
$result = "1";
foreach($partial_results as $part_res)
{
$result = bcmul($result, $part_res);
$result = bcmod($result, $r);
}
return $result;
}
//--
// Function to add padding to a decrypted string
// We need to know if this is a private or a public key operation [4]
//--
function add_PKCS1_padding($data, $isPublicKey, $blocksize)
{
$pad_length = $blocksize - 3 - strlen($data);
if($isPublicKey)
{
$block_type = "\x02";
$padding = "";
for($i = 0; $i {
$rnd = mt_rand(1, 255);
$padding .= chr($rnd);
}
}
else
{
$block_type = "\x01";
$padding = str_repeat("\xFF", $pad_length);
}
return "\x00" . $block_type . $padding . "\x00" . $data;
}
//--
// Remove padding from a decrypted string
// See [4] for more details.
//--
function remove_PKCS1_padding($data, $blocksize)
{
//以下部分于原版的RSA有所不同,修复了原版的一个BUG
//assert(strlen($data) == $blocksize);
$data = substr($data, 1);
// We cannot deal with block type 0
if($data{0} == '\0')
die("Block type 0 not implemented.");
// Then the block type must be 1 or 2
//assert(($data{0} == "\x01") || ($data{0} == "\x02"));
// echo $data;
// Remove the padding
$i=1;
while (1){
$offset = strpos($data, "\0", $i);
if(!$offset){
$offset=$i;
break;
}
$i=$offset+1;
}
//$offset = strpos($data, "\0", 100);
return substr($data, $offset);
}
//--
// Remove "kyp" padding
// (Non standard)
//--
function remove_KYP_padding($data, $blocksize)
{
assert(strlen($data) == $blocksize);
$offset = strpos($data, "\0");
return substr($data, 0, $offset);
}
//--
// Convert binary data to a decimal number
//--
function binary_to_number($data)
{
$base = "256";
$radix = "1";
$result = "0";
for($i = strlen($data) - 1; $i >= 0; $i--)
{
$digit = ord($data{$i});
$part_res = bcmul($digit, $radix);
$result = bcadd($result, $part_res);
$radix = bcmul($radix, $base);
}
return $result;
}
//--
// Convert a number back into binary form
//--
function number_to_binary($number, $blocksize)
{
$base = "256";
$result = "";
$div = $number;
while($div > 0)
{
$mod = bcmod($div, $base);
$div = bcdiv($div, $base);
$result = chr($mod) . $result;
}
return str_pad($result, $blocksize, "\x00", STR_PAD_LEFT);
}
?>
处理的PHP代码:
//Decimal Data
include "rsa.php";
$modulus='124124790696783899579957666732205416556275207289308772677367395397704314099727565633927507139389670490184904760526156031441045563225987129220634807383637837918320623518532877734472159024203477820731033762885040862183213160281165618500092483026873487507336293388981515466164416989192069833140532570993394388051.0000000000';
$private='59940207454900542501281722336097731406274284149290386158861762508911700758780200454438527029729836453810395133453343700246367853044479311924174899432036400630350527132581124575735909908195078492323048176864577497230467497768502277772070557874686662727818507841304646138785432507752788647631021854537869399041.0000000000';
$public="65537";
$keylength="1024";
//php encrypt create
//$encrypted = rsa_encrypt("vzxcvz bdxf", $public, $modulus, $keylength);
//$str= bin2hex($encrypted);//bin data to hex data
$str=$_POST['ciphertext'];
//echo $str."
";
$encrypted=convert($str); //hex data to bin data
$decrypted = rsa_decrypt($encrypted, $private, $modulus, $keylength);
echo $decrypted."
";
/**
* 16 to 2
* @param unknown_type $hexString
* @return string|unknown
*/
function convert($hexString)
{
$hexLenght = strlen($hexString);
// only hex numbers is allowed
if ($hexLenght % 2 != 0 || preg_match("/[^\da-fA-F]/",$hexString)) return FALSE;
unset($binString);
for ($x = 1; $x {
$binString .= chr(hexdec(substr($hexString,2 * $x - 2,2)));
}
return $binString;
}
?>
生成PRM文件及生产需要的密钥及公钥的PHP文件:
//create pem file
//run openssl genrsa -out key.pem 1024
//This file is generated variables needed for the operation
list($keylength, $modulus, $public, $private,$modulus_js,$private_js) = read_ssl_key("key.pem");
echo "keylength:(php and js)(private length)
";
echo $keylength;
echo "
";
echo "modulus:(php)(10)(pubic key)
";
echo $modulus;
echo "
";
echo "modulus:(js)(16)(pubic key)
";
echo $modulus_js;
echo "
";
echo "public:(php)(10)(public exponent)
";
echo $public;
echo "
";
echo "public:(js)(16)(public exponent)
";
echo "10001";
echo "
";
echo "private:(php)(10)(private key)
";
echo $private;
echo "
";
echo "private:(js)(16)(private key)
";
echo $private_js;
//function
function read_ssl_key($filename)
{
exec("openssl rsa -in $filename -text -noout", $raw);
// read the key length
$keylength = (int) expect($raw[0], "Private-Key: (");
// read the modulus
expect($raw[1], "modulus:");
for($i = 2; $raw[$i][0] == ' '; $i++) $modulusRaw .= trim($raw[$i]);
// read the public exponent
$public = (int) expect($raw[$i], "publicExponent: ");
// read the private exponent
expect($raw[$i + 1], "privateExponent:");
for($i += 2; $raw[$i][0] == ' '; $i++) $privateRaw .= trim($raw[$i]);
// Just to make sure
expect($raw[$i], "prime1:");
// Conversion to decimal format for bcmath
$modulus = bc_hexdec($modulusRaw);
$private = bc_hexdec($privateRaw);
return array($keylength, $modulus['php'], $public, $private['php'],$modulus['js'], $private['js']);
}
/*
* Convert a hexadecimal number of the form "XX:YY:ZZ:..." to decimal
* Uses BCmath, but the standard normal hexdec function for the components
*/
function bc_hexdec($hex)
{
$coefficients = explode(":", $hex);
$result_js= implode("",$coefficients);
$i = 0;
$result = 0;
foreach(array_reverse($coefficients) as $coefficient)
{
$mult = bcpow(256, $i++);
$result = bcadd($result, bcmul(hexdec($coefficient), $mult));
}
return array('php'=>$result,'js'=>$result_js);
}
/*
* If the string has the given prefix, return the remainder.
* If not, die with an error
*/
function expect($str, $prefix)
{
if(substr($str, 0, strlen($prefix)) == $prefix)
return substr($str, strlen($prefix));
else
die("Error: expected $prefix");
}
整套加密及解密的方法都在上面了,本人的测试环境为php5.3+WIN7
上面所有文件下载:RSAFILE

Outils d'IA chauds

Undresser.AI Undress
Application basée sur l'IA pour créer des photos de nu réalistes

AI Clothes Remover
Outil d'IA en ligne pour supprimer les vêtements des photos.

Undress AI Tool
Images de déshabillage gratuites

Clothoff.io
Dissolvant de vêtements AI

Video Face Swap
Échangez les visages dans n'importe quelle vidéo sans effort grâce à notre outil d'échange de visage AI entièrement gratuit !

Article chaud

Outils chauds

Bloc-notes++7.3.1
Éditeur de code facile à utiliser et gratuit

SublimeText3 version chinoise
Version chinoise, très simple à utiliser

Envoyer Studio 13.0.1
Puissant environnement de développement intégré PHP

Dreamweaver CS6
Outils de développement Web visuel

SublimeText3 version Mac
Logiciel d'édition de code au niveau de Dieu (SublimeText3)

Sujets chauds
 1662
1662
 14
14
 1419
1419
 52
52
 1313
1313
 25
25
 1262
1262
 29
29
 1235
1235
 24
24
 Open source! Au-delà de ZoeDepth ! DepthFM : estimation rapide et précise de la profondeur monoculaire !
Apr 03, 2024 pm 12:04 PM
Open source! Au-delà de ZoeDepth ! DepthFM : estimation rapide et précise de la profondeur monoculaire !
Apr 03, 2024 pm 12:04 PM
0. À quoi sert cet article ? Nous proposons DepthFM : un modèle d'estimation de profondeur monoculaire génératif de pointe, polyvalent et rapide. En plus des tâches traditionnelles d'estimation de la profondeur, DepthFM démontre également des capacités de pointe dans les tâches en aval telles que l'inpainting en profondeur. DepthFM est efficace et peut synthétiser des cartes de profondeur en quelques étapes d'inférence. Lisons ce travail ensemble ~ 1. Titre des informations sur l'article : DepthFM : FastMonocularDepthEstimationwithFlowMatching Auteur : MingGui, JohannesS.Fischer, UlrichPrestel, PingchuanMa, Dmytr
 Google est ravi : les performances de JAX surpassent Pytorch et TensorFlow ! Cela pourrait devenir le choix le plus rapide pour la formation à l'inférence GPU
Apr 01, 2024 pm 07:46 PM
Google est ravi : les performances de JAX surpassent Pytorch et TensorFlow ! Cela pourrait devenir le choix le plus rapide pour la formation à l'inférence GPU
Apr 01, 2024 pm 07:46 PM
Les performances de JAX, promu par Google, ont dépassé celles de Pytorch et TensorFlow lors de récents tests de référence, se classant au premier rang sur 7 indicateurs. Et le test n’a pas été fait sur le TPU présentant les meilleures performances JAX. Bien que parmi les développeurs, Pytorch soit toujours plus populaire que Tensorflow. Mais à l’avenir, des modèles plus volumineux seront peut-être formés et exécutés sur la base de la plate-forme JAX. Modèles Récemment, l'équipe Keras a comparé trois backends (TensorFlow, JAX, PyTorch) avec l'implémentation native de PyTorch et Keras2 avec TensorFlow. Premièrement, ils sélectionnent un ensemble de
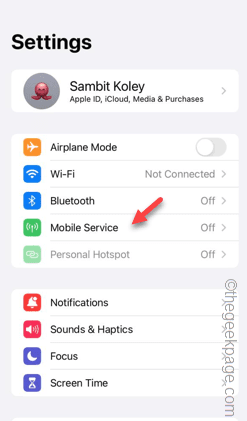 Vitesse Internet lente des données cellulaires sur iPhone : correctifs
May 03, 2024 pm 09:01 PM
Vitesse Internet lente des données cellulaires sur iPhone : correctifs
May 03, 2024 pm 09:01 PM
Vous êtes confronté à un décalage et à une connexion de données mobile lente sur iPhone ? En règle générale, la puissance de l'Internet cellulaire sur votre téléphone dépend de plusieurs facteurs tels que la région, le type de réseau cellulaire, le type d'itinérance, etc. Vous pouvez prendre certaines mesures pour obtenir une connexion Internet cellulaire plus rapide et plus fiable. Correctif 1 – Forcer le redémarrage de l'iPhone Parfois, le redémarrage forcé de votre appareil réinitialise simplement beaucoup de choses, y compris la connexion cellulaire. Étape 1 – Appuyez simplement une fois sur la touche d’augmentation du volume et relâchez-la. Ensuite, appuyez sur la touche de réduction du volume et relâchez-la à nouveau. Étape 2 – La partie suivante du processus consiste à maintenir le bouton sur le côté droit. Laissez l'iPhone finir de redémarrer. Activez les données cellulaires et vérifiez la vitesse du réseau. Vérifiez à nouveau Correctif 2 – Changer le mode de données Bien que la 5G offre de meilleures vitesses de réseau, elle fonctionne mieux lorsque le signal est plus faible
 Les robots Tesla travaillent dans les usines, Musk : Le degré de liberté des mains atteindra 22 cette année !
May 06, 2024 pm 04:13 PM
Les robots Tesla travaillent dans les usines, Musk : Le degré de liberté des mains atteindra 22 cette année !
May 06, 2024 pm 04:13 PM
La dernière vidéo du robot Optimus de Tesla est sortie, et il peut déjà fonctionner en usine. À vitesse normale, il trie les batteries (les batteries 4680 de Tesla) comme ceci : Le responsable a également publié à quoi cela ressemble à une vitesse 20 fois supérieure - sur un petit "poste de travail", en sélectionnant et en sélectionnant et en sélectionnant : Cette fois, il est publié L'un des points forts de la vidéo est qu'Optimus réalise ce travail en usine, de manière totalement autonome, sans intervention humaine tout au long du processus. Et du point de vue d'Optimus, il peut également récupérer et placer la batterie tordue, en se concentrant sur la correction automatique des erreurs : concernant la main d'Optimus, le scientifique de NVIDIA Jim Fan a donné une évaluation élevée : la main d'Optimus est l'un des robots à cinq doigts du monde. le plus adroit. Ses mains ne sont pas seulement tactiles
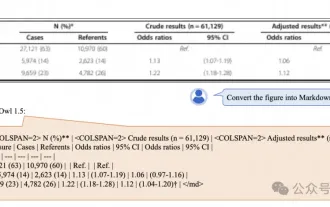 Le document multimodal Alibaba 7B comprenant le grand modèle remporte le nouveau SOTA
Apr 02, 2024 am 11:31 AM
Le document multimodal Alibaba 7B comprenant le grand modèle remporte le nouveau SOTA
Apr 02, 2024 am 11:31 AM
Nouveau SOTA pour des capacités de compréhension de documents multimodaux ! L'équipe Alibaba mPLUG a publié le dernier travail open source mPLUG-DocOwl1.5, qui propose une série de solutions pour relever les quatre défis majeurs que sont la reconnaissance de texte d'image haute résolution, la compréhension générale de la structure des documents, le suivi des instructions et l'introduction de connaissances externes. Sans plus tarder, examinons d’abord les effets. Reconnaissance et conversion en un clic de graphiques aux structures complexes au format Markdown : Des graphiques de différents styles sont disponibles : Une reconnaissance et un positionnement de texte plus détaillés peuvent également être facilement traités : Des explications détaillées sur la compréhension du document peuvent également être données : Vous savez, « Compréhension du document " est actuellement un scénario important pour la mise en œuvre de grands modèles linguistiques. Il existe de nombreux produits sur le marché pour aider à la lecture de documents. Certains d'entre eux utilisent principalement des systèmes OCR pour la reconnaissance de texte et coopèrent avec LLM pour le traitement de texte.
 La vitalité de la super intelligence s'éveille ! Mais avec l'arrivée de l'IA qui se met à jour automatiquement, les mères n'ont plus à se soucier des goulots d'étranglement des données.
Apr 29, 2024 pm 06:55 PM
La vitalité de la super intelligence s'éveille ! Mais avec l'arrivée de l'IA qui se met à jour automatiquement, les mères n'ont plus à se soucier des goulots d'étranglement des données.
Apr 29, 2024 pm 06:55 PM
Je pleure à mort. Le monde construit à la folie de grands modèles. Les données sur Internet ne suffisent pas du tout. Le modèle de formation ressemble à « The Hunger Games », et les chercheurs en IA du monde entier se demandent comment nourrir ces personnes avides de données. Ce problème est particulièrement important dans les tâches multimodales. À une époque où rien ne pouvait être fait, une équipe de start-up du département de l'Université Renmin de Chine a utilisé son propre nouveau modèle pour devenir la première en Chine à faire de « l'auto-alimentation des données générées par le modèle » une réalité. De plus, il s’agit d’une approche à deux volets, du côté compréhension et du côté génération, les deux côtés peuvent générer de nouvelles données multimodales de haute qualité et fournir un retour de données au modèle lui-même. Qu'est-ce qu'un modèle ? Awaker 1.0, un grand modèle multimodal qui vient d'apparaître sur le Forum Zhongguancun. Qui est l'équipe ? Moteur Sophon. Fondé par Gao Yizhao, doctorant à la Hillhouse School of Artificial Intelligence de l’Université Renmin.
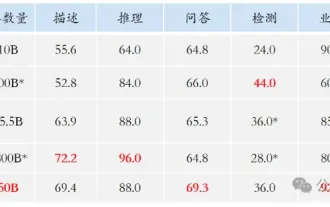
 Une seule carte exécute Llama 70B plus rapidement que deux cartes, Microsoft vient de mettre le FP6 dans l'Open source A100 |
Apr 29, 2024 pm 04:55 PM
Une seule carte exécute Llama 70B plus rapidement que deux cartes, Microsoft vient de mettre le FP6 dans l'Open source A100 |
Apr 29, 2024 pm 04:55 PM
Le FP8 et la précision de quantification inférieure en virgule flottante ne sont plus le « brevet » du H100 ! Lao Huang voulait que tout le monde utilise INT8/INT4, et l'équipe Microsoft DeepSpeed a commencé à exécuter FP6 sur A100 sans le soutien officiel de NVIDIA. Les résultats des tests montrent que la quantification FP6 de la nouvelle méthode TC-FPx sur A100 est proche ou parfois plus rapide que celle de INT4, et a une précision supérieure à celle de cette dernière. En plus de cela, il existe également une prise en charge de bout en bout des grands modèles, qui ont été open source et intégrés dans des cadres d'inférence d'apprentissage profond tels que DeepSpeed. Ce résultat a également un effet immédiat sur l'accélération des grands modèles : dans ce cadre, en utilisant une seule carte pour exécuter Llama, le débit est 2,65 fois supérieur à celui des cartes doubles. un
 L'US Air Force présente son premier avion de combat IA de grande envergure ! Le ministre a personnellement effectué l'essai routier sans intervenir pendant tout le processus, et 100 000 lignes de code ont été testées 21 fois.
May 07, 2024 pm 05:00 PM
L'US Air Force présente son premier avion de combat IA de grande envergure ! Le ministre a personnellement effectué l'essai routier sans intervenir pendant tout le processus, et 100 000 lignes de code ont été testées 21 fois.
May 07, 2024 pm 05:00 PM
Récemment, le milieu militaire a été submergé par la nouvelle : les avions de combat militaires américains peuvent désormais mener des combats aériens entièrement automatiques grâce à l'IA. Oui, tout récemment, l’avion de combat IA de l’armée américaine a été rendu public pour la première fois, dévoilant ainsi son mystère. Le nom complet de ce chasseur est Variable Stability Simulator Test Aircraft (VISTA). Il a été personnellement piloté par le secrétaire de l'US Air Force pour simuler une bataille aérienne en tête-à-tête. Le 2 mai, le secrétaire de l'US Air Force, Frank Kendall, a décollé à bord d'un X-62AVISTA à la base aérienne d'Edwards. Notez que pendant le vol d'une heure, toutes les actions de vol ont été effectuées de manière autonome par l'IA ! Kendall a déclaré : "Au cours des dernières décennies, nous avons réfléchi au potentiel illimité du combat air-air autonome, mais cela a toujours semblé hors de portée." Mais maintenant,




