 Java
Java
 javaDidacticiel
javaDidacticiel
 Comment implémenter l'algorithme de recherche prioritaire en largeur de file d'attente en Java
Comment implémenter l'algorithme de recherche prioritaire en largeur de file d'attente en Java
Comment implémenter l'algorithme de recherche prioritaire en largeur de file d'attente en Java
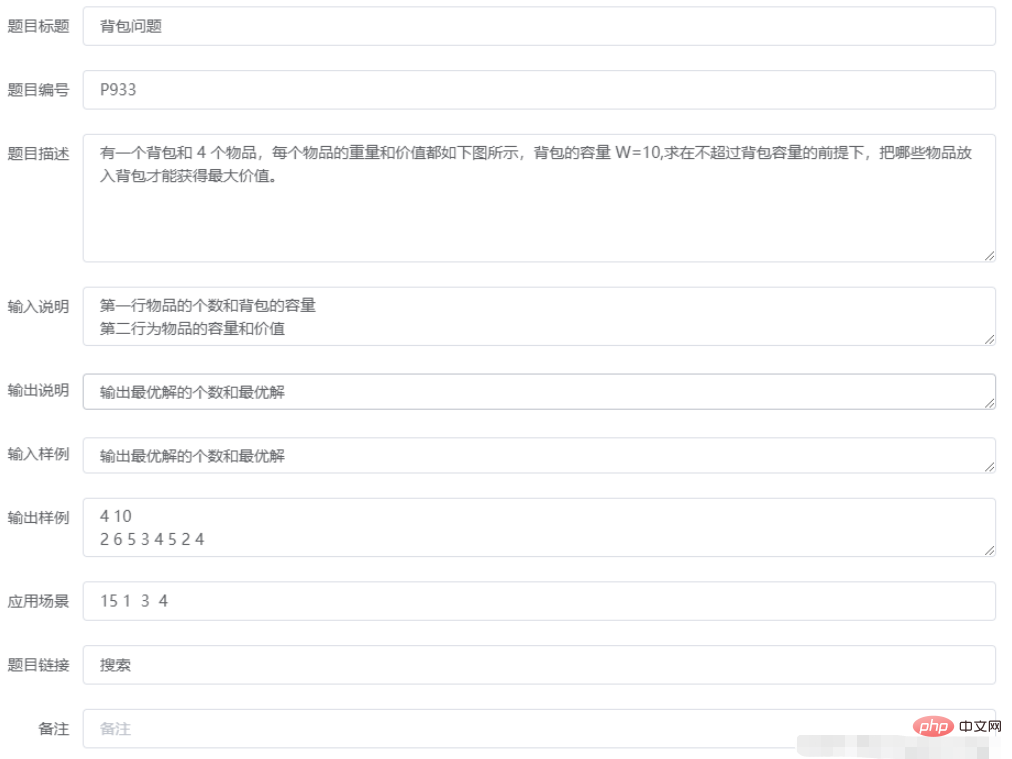
1.Description du problème
2.Mise en œuvre
package com.platform.modules.alg.alglib.p933;
import java.util.Arrays;
import java.util.PriorityQueue;
public class P933 {
public static final int N = 10;
// 记录最优解
boolean bestx[] = new boolean[N];
// 辅助数组,用于存储排序后的重量和价值
private int w[] = new int[N];
private int v[] = new int[N];
Goods goods[] = new Goods[N];
Object S[] = new Object[N];
// 用来记录最优解
Integer bestp;
// 为背包的最大容量
int W;
// 为物品的个数。
int n;
// 为所有物品的总重量。
int sumw;
// 为所有物品的总价值
int sumv;
public String output = "";
public P933() {
for (int i = 0; i < goods.length; i++) {
goods[i] = new Goods();
}
for (int i = 0; i < S.length; i++) {
S[i] = new Object();
}
}
// 计算节点的上界
double Bound(Node tnode) {
// 已装入背包物品价值
double maxvalue = tnode.cp;
int t = tnode.id; // 排序后序号
double left = tnode.rw; // 剩余容量
while (t <= n && w[t] <= left) {
maxvalue += v[t];
left -= w[t++];
}
if (t <= n)
maxvalue += ((double) (v[t])) / w[t] * left;
return maxvalue;
}
public String cal(String input) {
String[] line = input.split("\n");
String[] words = line[0].split(" ");
// 物品的个数和背包的容量
n = Integer.parseInt(words[0]);
W = Integer.parseInt(words[1]);
bestp = 0; // 用来记录最优解
sumw = 0; // sumw 为所有物品的总重量。
sumv = 0; // sumv为所有物品的总价值
words = line[1].split(" ");
for (int i = 1; i <= words.length / 2; i++) { // 输入每个物品的重量和价值,用空格分开
goods[i].weight = Integer.parseInt(words[2 * i - 2]);
goods[i].value = Integer.parseInt(words[2 * i - 1]);
sumw += goods[i].weight;
sumv += goods[i].value;
S[i - 1].id = i;
S[i - 1].d = 1.0 * goods[i].value / goods[i].weight;
}
if (sumw <= W) {
bestp = sumv;
output = bestp.toString();
return output;
}
Arrays.sort(S); // 按价值重量比非递增排序
for (int i = 1; i <= n; i++) {//把排序后的数据传递给辅助数组
w[i] = goods[S[i - 1].id].weight;
v[i] = goods[S[i - 1].id].value;
}
priorbfs();//优先队列分支限界法
output += bestp + "\n";
for (int i = 1; i <= n; i++) { // 输出最优解
if (bestx[i])
output += S[i - 1].id + " "; // 输出原物品序号(排序前的)
}
return output;
}
// 优先队列式分支限界法
int priorbfs() {
// 当前处理的物品序号t,当前装入背包物品价值tcp,当前剩余容量trw
int t, tcp, trw;
double tup; // 当前价值上界 tup
PriorityQueue<Node> q = new PriorityQueue<>(); // 优先队列
q.add(new Node(0, sumv, W, 1)); // 初始化,根结点加入优先队列
while (!q.isEmpty()) {
// 定义三个结点型变量
Node livenode;
Node lchild = new Node();
Node rchild = new Node();
livenode = q.peek(); // 取出队头元素作为当前扩展结点 livenode
q.poll(); // 队头元素出队
t = livenode.id; // 当前处理的物品序号
// 搜到最后一个物品的时候不需要往下搜索。
// 如果当前的背包没有剩余容量(已经装满)了,不再扩展。
if (t > n || livenode.rw == 0) {
if (livenode.cp >= bestp) { // 更新最优解和最优值
for (int i = 1; i <= n; i++)
bestx[i] = livenode.x[i];
bestp = livenode.cp;
}
continue;
}
if (livenode.up < bestp)//如果不满足不再扩展
continue;
tcp = livenode.cp; //当前背包中的价值
trw = livenode.rw; //背包剩余容量
if (trw >= w[t]) { //扩展左孩子,满足约束条件,可以放入背包
lchild.cp = tcp + v[t];
lchild.rw = trw - w[t];
lchild.id = t + 1;
tup = Bound(lchild); //计算左孩子上界
lchild = new Node(lchild.cp, tup, lchild.rw, lchild.id);
for (int i = 1; i <= n; i++)//复制以前的解向量
lchild.x[i] = livenode.x[i];
lchild.x[t] = true;
if (lchild.cp > bestp)//比最优值大才更新
bestp = lchild.cp;
q.add(lchild);//左孩子入队
}
rchild.cp = tcp;
rchild.rw = trw;
rchild.id = t + 1;
tup = Bound(rchild);//计算右孩子上界
if (tup >= bestp) {//扩展右孩子,满足限界条件,不放入
rchild = new Node(tcp, tup, trw, t + 1);
for (int i = 1; i <= n; i++)//复制以前的解向量
rchild.x[i] = livenode.x[i];
rchild.x[t] = false;
q.add(rchild);//右孩子入队
}
}
return bestp;//返回最优值。
}
}
// 定义结点。每个节点来记录当前的解。
class Node implements Comparable<Node> {
int cp; // cp 为当前装入背包的物品总价值
double up; // 价值上界
int rw; // 剩余容量
int id; // 物品号
boolean x[] = new boolean[P933.N]; // 解向量
Node() {
}
Node(int _cp, double _up, int _rw, int _id) {
cp = _cp;
up = _up;
rw = _rw;
id = _id;
}
@Override
public int compareTo(Node o) {
return (this.up - o.up) > 0 ? 1 : -1;
}
}
// 物品
class Goods {
int weight; // 重量
int value; // 价值
}
// 辅助物品结构体,用于按单位重量价值(价值/重量比)排序
class Object implements Comparable {
int id; // 序号
double d; // 单位重量价值
@Override
public int compareTo(java.lang.Object o) {
return this.d > ((Object) o).d ? -1 : 1;
}
}3.Test
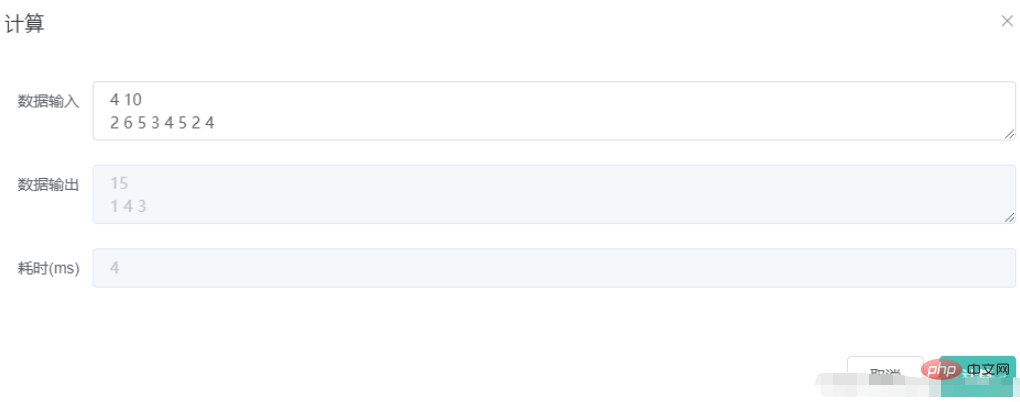
Ce qui précède est le contenu détaillé de. pour plus d'informations, suivez d'autres articles connexes sur le site Web de PHP en chinois!

Outils d'IA chauds

Undresser.AI Undress
Application basée sur l'IA pour créer des photos de nu réalistes

AI Clothes Remover
Outil d'IA en ligne pour supprimer les vêtements des photos.

Undress AI Tool
Images de déshabillage gratuites

Clothoff.io
Dissolvant de vêtements AI

Video Face Swap
Échangez les visages dans n'importe quelle vidéo sans effort grâce à notre outil d'échange de visage AI entièrement gratuit !

Article chaud

Outils chauds

Bloc-notes++7.3.1
Éditeur de code facile à utiliser et gratuit

SublimeText3 version chinoise
Version chinoise, très simple à utiliser

Envoyer Studio 13.0.1
Puissant environnement de développement intégré PHP

Dreamweaver CS6
Outils de développement Web visuel

SublimeText3 version Mac
Logiciel d'édition de code au niveau de Dieu (SublimeText3)
 Nombre parfait en Java
Aug 30, 2024 pm 04:28 PM
Nombre parfait en Java
Aug 30, 2024 pm 04:28 PM
Guide du nombre parfait en Java. Nous discutons ici de la définition, comment vérifier le nombre parfait en Java ?, des exemples d'implémentation de code.
 Weka en Java
Aug 30, 2024 pm 04:28 PM
Weka en Java
Aug 30, 2024 pm 04:28 PM
Guide de Weka en Java. Nous discutons ici de l'introduction, de la façon d'utiliser Weka Java, du type de plate-forme et des avantages avec des exemples.
 Numéro de Smith en Java
Aug 30, 2024 pm 04:28 PM
Numéro de Smith en Java
Aug 30, 2024 pm 04:28 PM
Guide du nombre de Smith en Java. Nous discutons ici de la définition, comment vérifier le numéro Smith en Java ? exemple avec implémentation de code.
 Questions d'entretien chez Java Spring
Aug 30, 2024 pm 04:29 PM
Questions d'entretien chez Java Spring
Aug 30, 2024 pm 04:29 PM
Dans cet article, nous avons conservé les questions d'entretien Java Spring les plus posées avec leurs réponses détaillées. Pour que vous puissiez réussir l'interview.
 Break or Return of Java 8 Stream Forach?
Feb 07, 2025 pm 12:09 PM
Break or Return of Java 8 Stream Forach?
Feb 07, 2025 pm 12:09 PM
Java 8 présente l'API Stream, fournissant un moyen puissant et expressif de traiter les collections de données. Cependant, une question courante lors de l'utilisation du flux est: comment se casser ou revenir d'une opération FOREAK? Les boucles traditionnelles permettent une interruption ou un retour précoce, mais la méthode Foreach de Stream ne prend pas directement en charge cette méthode. Cet article expliquera les raisons et explorera des méthodes alternatives pour la mise en œuvre de terminaison prématurée dans les systèmes de traitement de flux. Lire plus approfondie: Améliorations de l'API Java Stream Comprendre le flux Forach La méthode foreach est une opération terminale qui effectue une opération sur chaque élément du flux. Son intention de conception est
 Horodatage à ce jour en Java
Aug 30, 2024 pm 04:28 PM
Horodatage à ce jour en Java
Aug 30, 2024 pm 04:28 PM
Guide de TimeStamp to Date en Java. Ici, nous discutons également de l'introduction et de la façon de convertir l'horodatage en date en Java avec des exemples.
 Programme Java pour trouver le volume de la capsule
Feb 07, 2025 am 11:37 AM
Programme Java pour trouver le volume de la capsule
Feb 07, 2025 am 11:37 AM
Les capsules sont des figures géométriques tridimensionnelles, composées d'un cylindre et d'un hémisphère aux deux extrémités. Le volume de la capsule peut être calculé en ajoutant le volume du cylindre et le volume de l'hémisphère aux deux extrémités. Ce tutoriel discutera de la façon de calculer le volume d'une capsule donnée en Java en utilisant différentes méthodes. Formule de volume de capsule La formule du volume de la capsule est la suivante: Volume de capsule = volume cylindrique volume de deux hémisphères volume dans, R: Le rayon de l'hémisphère. H: La hauteur du cylindre (à l'exclusion de l'hémisphère). Exemple 1 entrer Rayon = 5 unités Hauteur = 10 unités Sortir Volume = 1570,8 unités cubes expliquer Calculer le volume à l'aide de la formule: Volume = π × r2 × h (4
 Créer l'avenir : programmation Java pour les débutants absolus
Oct 13, 2024 pm 01:32 PM
Créer l'avenir : programmation Java pour les débutants absolus
Oct 13, 2024 pm 01:32 PM
Java est un langage de programmation populaire qui peut être appris aussi bien par les développeurs débutants que par les développeurs expérimentés. Ce didacticiel commence par les concepts de base et progresse vers des sujets avancés. Après avoir installé le kit de développement Java, vous pouvez vous entraîner à la programmation en créant un simple programme « Hello, World ! ». Une fois que vous avez compris le code, utilisez l'invite de commande pour compiler et exécuter le programme, et « Hello, World ! » s'affichera sur la console. L'apprentissage de Java commence votre parcours de programmation et, à mesure que votre maîtrise s'approfondit, vous pouvez créer des applications plus complexes.





