 développement back-end
développement back-end
 C++
C++
 Écrivez un programme en C++ pour trouver le nombre de sous-tableaux avec une somme dans une plage donnée
Écrivez un programme en C++ pour trouver le nombre de sous-tableaux avec une somme dans une plage donnée
Écrivez un programme en C++ pour trouver le nombre de sous-tableaux avec une somme dans une plage donnée

在本文中,我们将使用 C++ 程序求解总和在给定范围内的子数组的数量。我们有一个正整数数组 arr[] 和一个范围 {L, R},我们必须计算总和在给定范围 L 到 R 内的子数组的总数。所以这是该问题的简单示例 -
Input : arr[] = {1, 4, 6}, L = 3, R = 8
Output : 3
The subarrays are {1, 4}, {4}, {6}.
Input : arr[] = {2, 3, 5, 8}, L = 4, R = 13
Output : 6
The subarrays are {2, 3}, {2, 3, 5}, {3, 5},
{5}, {5, 8}, {8}.寻找解决方案的方法
我们将解释使用 C++ 问题解决此问题的两种方法 -
暴力方法
最基本的暴力方法方法用于计算每个子数组的总和,然后查找该总和是否存在于给定范围内。 (但是这种方法会花费我们很多时间,因为它的时间复杂度是 O(n*n),其中 n 是数组的大小)。
高效的方法
节省现在,有效的方法是使用滑动窗口技术,使用这种技术,我们将在 O(n) 内更快或更有效地计算结果。
示例
#include <bits/stdc++.h>
using namespace std;
int subCount(int *arr, int n, int x){
int start = 0, end = 0, sum = 0, count = 0;
while (end < n){ // we will be moving right border in this loop
sum = sum + arr[end];
while(start <= end && sum >= x){ // this loop will move our left border
sum = sum - arr[start]; // we will decrement sum while moving left border.
// For excluding the previous elements.
start++; // and move the left border.
}
count = count + ((end - start) + 1); // counting the subarrays.
end++;
}
return count;
}
int main(){
int arr[] = { 1, 4, 6 };
int n = sizeof(arr) / sizeof(arr[0]);
int L = 3;
int R = 8;
int answer;
answer = subCount(arr, n, R) - subCount(arr, n, (L - 1)); // Final Answer.
cout << answer << "\n";
return 0;
}输出
3
上述代码说明
在这种方法中,我们计算总和小于给定范围上限的子数组的数量,然后减去总和小于给定范围上限的子数组的数量。使用 subcount 函数小于给定范围的下限。
Subcount 函数
该函数使用滑动窗口技术来查找计数小于 x 的子数组的计数.
首先,我们从“结束”和“开始”开始,其值均为 0。当我们遍历数组时,我们维护从头到尾的元素之和。之后,如果我们的开始等于结束并且总和大于或等于 x,我们开始移动开始并在从总和中取出元素时不断减少总和。
直到我们的总和变得小于 x 或者我们的开始变得大于结束。现在,我们将计数增加子数组计数,然后将右边界增加 1。现在,在外循环结束后,我们返回子数组的总计数。
结论
在本文中,我们使用滑动窗口技术解决了一个问题,即在 O(n) 时间复杂度内找到总和在给定范围内的子数组的数量。我们还从C++程序中学习了这个问题以及我们可以轻松解决这个问题的完整方法(正常且高效)。我们可以用其他语言(例如 C、java、python 等)编写相同的程序。
Ce qui précède est le contenu détaillé de. pour plus d'informations, suivez d'autres articles connexes sur le site Web de PHP en chinois!

Outils d'IA chauds

Undresser.AI Undress
Application basée sur l'IA pour créer des photos de nu réalistes

AI Clothes Remover
Outil d'IA en ligne pour supprimer les vêtements des photos.

Undress AI Tool
Images de déshabillage gratuites

Clothoff.io
Dissolvant de vêtements AI

AI Hentai Generator
Générez AI Hentai gratuitement.

Article chaud

Outils chauds

Bloc-notes++7.3.1
Éditeur de code facile à utiliser et gratuit

SublimeText3 version chinoise
Version chinoise, très simple à utiliser

Envoyer Studio 13.0.1
Puissant environnement de développement intégré PHP

Dreamweaver CS6
Outils de développement Web visuel

SublimeText3 version Mac
Logiciel d'édition de code au niveau de Dieu (SublimeText3)
 Utilisez C++ pour écrire du code afin de trouver le Nième nombre non carré
Aug 30, 2023 pm 10:41 PM
Utilisez C++ pour écrire du code afin de trouver le Nième nombre non carré
Aug 30, 2023 pm 10:41 PM
Nous connaissons tous des nombres qui ne sont le carré d’aucun nombre, comme 2, 3, 5, 7, 8, etc. Il existe N nombres non carrés et il est impossible de connaître tous les nombres. Ainsi, dans cet article, nous expliquerons tout sur les nombres sans carrés ou non carrés et les moyens de trouver le Nième nombre non carré en C++. Nième nombre non carré Si un nombre est le carré d'un entier, alors ce nombre est appelé un carré parfait. Quelques exemples de nombres carrés parfaits sont -1iscarréde14iscarréde29iscarréde316iscarréde425iscarréde5 Si un nombre n'est le carré d'aucun entier, alors le nombre est appelé non carré. Par exemple, les 15 premiers nombres non carrés sont -2,3,5,6,
 Algorithme d'inversion pour la rotation à droite du tableau écrit en C++
Sep 08, 2023 pm 08:17 PM
Algorithme d'inversion pour la rotation à droite du tableau écrit en C++
Sep 08, 2023 pm 08:17 PM
Dans cet article, nous découvrirons l'algorithme d'inversion pour faire pivoter le tableau donné vers la droite de k éléments, par exemple −Input:arr[]={4,6,2,6,43,7,3,7}, k= 4Sortie :{43,7,3,7,4,6,2,6}Explication : La rotation de chaque élément du tableau par 4 éléments vers la droite donne{43,7,3,7,4,6,2,6}.Entrée :arr[]= {8 ,5,8,2,1,4,9,3},k=3Sortie :{4,9,3,8,5,8,2,1} Trouver la solution
 En programmation C, trouver l'aire d'un cercle
Aug 25, 2023 pm 10:57 PM
En programmation C, trouver l'aire d'un cercle
Aug 25, 2023 pm 10:57 PM
Un cercle est une figure fermée. Tous les points d'un cercle sont équidistants d'un point à l'intérieur du cercle. Le point central est appelé le centre du cercle. La distance d’un point au centre d’un cercle s’appelle le rayon. L'aire est une représentation quantitative de l'étendue des dimensions d'une figure fermée. L'aire d'un cercle est l'aire délimitée par les dimensions du cercle. La formule pour calculer l'aire d'un cercle, Aire=π*r*r Pour calculer l'aire, nous donnons le rayon du cercle en entrée, nous utiliserons la formule pour calculer l'aire, algorithme ÉTAPE 1 : Prendre le rayon comme entrée de l'utilisateur utilisant st dinput.ÉTAPE 2 : Calculez l'aire du cercle en utilisant, aire = (
 En Java, recherchez la somme maximale des sous-tableaux après avoir divisé un tableau en sous-tableaux en fonction d'une requête donnée.
Aug 29, 2023 am 11:21 AM
En Java, recherchez la somme maximale des sous-tableaux après avoir divisé un tableau en sous-tableaux en fonction d'une requête donnée.
Aug 29, 2023 am 11:21 AM
Nous avons deux tableaux d'entiers, l'un avec les éléments calculés et l'autre avec les points de division nécessaires pour diviser le tableau afin de générer des sous-ensembles, nous devons calculer la somme de chaque sous-ensemble dans chaque division et renvoyer le sous-ensemble maximum. Passons en revue l'exemple Comprendre : - input −intarr[]=intarr[]={9,4,5,6,7}intsplitPoints[]={0,2,3,1} ; sortie−la somme maximale du sous-tableau après chaque division [ 22, 13,9,9] Explication - Ici, nous décomposons le tableau en fonction de ses points de division et obtenons le sous-ensemble maximum après chaque division et après la première division → {9} et {4,5,6,7 }>> La somme maximale des sous-tableaux est de -22 après la deuxième division →{9},{4
 Trouver le nombre de paires uniques dans un tableau en utilisant C++
Sep 07, 2023 am 11:53 AM
Trouver le nombre de paires uniques dans un tableau en utilisant C++
Sep 07, 2023 am 11:53 AM
Nous avons besoin de connaissances appropriées pour créer plusieurs paires uniques dans la syntaxe des tableaux de C++. Tout en trouvant le nombre de paires uniques, nous comptons toutes les paires uniques dans le tableau donné, c'est-à-dire que toutes les paires possibles peuvent être formées où chaque paire doit être unique. Par exemple -Input:array[]={5,5,9}Output:4Explication:Thenumberoffalluniquepairsare(5,5),(5,9),(9,5)and(9,9).Input:array[] = {5,4,3,2,2}Sortie : 16 façons de trouver une solution Il existe deux façons de résoudre ce problème, ce sont -
 Écrivez un code en C++ pour trouver le nombre de sous-tableaux avec les mêmes valeurs minimales et maximales
Aug 25, 2023 pm 11:33 PM
Écrivez un code en C++ pour trouver le nombre de sous-tableaux avec les mêmes valeurs minimales et maximales
Aug 25, 2023 pm 11:33 PM
Dans cet article, nous utiliserons C++ pour résoudre le problème de trouver le nombre de sous-tableaux dont les valeurs maximales et minimales sont les mêmes. Voici un exemple du problème −Input:array={2,3,6,6,2,4,4,4}Output:12Explication :{2},{3},{6},{6}, {2 },{4},{4},{4},{6,6},{4,4},{4,4}et{4,4,4}sont les sous-tableaux qui peuvent être formés avec les mêmes éléments maximum et minimum. Entrée : tableau = {3, 3, 1,5,
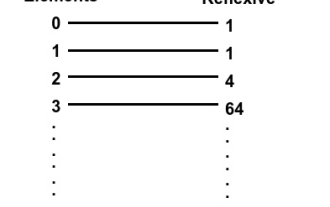 Écrit en C++, trouver le nombre de relations réflexives sur un ensemble
Aug 26, 2023 pm 08:17 PM
Écrit en C++, trouver le nombre de relations réflexives sur un ensemble
Aug 26, 2023 pm 08:17 PM
Dans cet article, nous expliquerons comment trouver des relations réflexives sur un ensemble. Dans ce problème, on nous donne un nombre n et un ensemble de n nombres naturels, et nous devons déterminer le nombre de relations réflexives. Relation réflexive - Une relation R est dite être une relation réflexive sur l'ensemble A si pour chaque 'a' dans l'ensemble A, (a, a) appartient à la relation R. Par exemple -Input:x=1Output:1Explanation:set={1},reflexiverelationsonA*A:{{1}}Input:x=2Output:4Explanation:set={1,2},reflexiverelationsonA*
 Inverser le regroupement de listes doublement chaînées par taille donnée en utilisant C++
Sep 04, 2023 am 09:49 AM
Inverser le regroupement de listes doublement chaînées par taille donnée en utilisant C++
Sep 04, 2023 am 09:49 AM
Dans ce problème, on nous donne un pointeur vers la tête de la liste chaînée et un entier k. Dans un groupe de taille k, nous devons inverser la liste chaînée. Par exemple -Input:1<->2<->3<->4<->5(doublelylinkedlist),k=3Output:3<->2<->1<->5<->4 à la recherche de solutions Méthode Dans ce problème, nous formulerons un algorithme récursif pour résoudre ce problème. Dans cette méthode, nous utiliserons la récursivité et résoudrons le problème en utilisant la récursivité. Exemple#include<iostream&





