多項式回帰アルゴリズムを計算する C プログラム

回帰は、従属変数と非従属変数の間の関係を研究する予測モデリング手法です。
多項式回帰
これは、独立変数 x と従属変数 y の関係を示す回帰分析の形式であり、x の n 次多項式モデルです。
例
次は、多項式回帰アルゴリズムを計算するための C プログラムです -
#include<math.h>
#include<stdio.h>
#include<conio.h>
main(){
int i,j,k,m,n;
float x[20],y[20],u,a[10],c[20][20],power,r;
printf("enter m,n:");
scanf("%d%d",&m,&n);
for(i=1;i<=n;i++){
printf("enter values of x and y");
scanf("%f%f",&x[i],&y[i]);
}
for(j=1;j<=m+1;j++)
for(k=1;k<=m+1;k++){
c[j][k]=0;
for(i=1;i<=n;i++){
power=pow(x[i],j+k-2);
c[j][k]=c[j][k]+power;
}
}
for(j=1;j<=m+1;j++){
c[j][m+2]=0;
for(i=1;i<=n;i++){
r=pow(x[i],j-1);
c[j][m+2]=c[j][m+2]+y[i]*r;
}
}
for(i=1;i<=m+1;i++){
for(j=1;j<=m+2;j++){
printf("%.2f\t",c[i][j]);
}
printf("</p><p>");
}
for(k=1;k<=m+1;k++)
for(i=1;i<=m+1;i++){
if(i!=k){
u=c[i][k]/c[k][k];
for(j=k;j<=m+2;j++){
c[i][j]=c[i][j]-u*c[k][j];
}
}
}
for(i=1;i<=m+1;i++){
a[i]=c[i][m+2]/c[i][i];
printf("a[%d]=%f</p><p>",i,a[i]);
}
getch();
}enter m,n:4 5 enter values of x and y1 1 enter values of x and y2 3 enter values of x and y1 2 enter values of x and y1 2 enter values of x and y1 1 5.00 6.00 8.00 12.00 20.00 9.00 6.00 8.00 12.00 20.00 36.00 12.00 8.00 12.00 20.00 36.00 68.00 18.00 12.00 20.00 36.00 68.00 132.00 30.00 20.00 36.00 68.00 132.00 260.00 54.00 a[1]=1.750000 a[2]=-2.375000 a[3]=2.000000 a[4]=0.500000 a[5]=-0.375000
以上が多項式回帰アルゴリズムを計算する C プログラムの詳細内容です。詳細については、PHP 中国語 Web サイトの他の関連記事を参照してください。

ホットAIツール

Undresser.AI Undress
リアルなヌード写真を作成する AI 搭載アプリ

AI Clothes Remover
写真から衣服を削除するオンライン AI ツール。

Undress AI Tool
脱衣画像を無料で

Clothoff.io
AI衣類リムーバー

Video Face Swap
完全無料の AI 顔交換ツールを使用して、あらゆるビデオの顔を簡単に交換できます。

人気の記事

ホットツール

メモ帳++7.3.1
使いやすく無料のコードエディター

SublimeText3 中国語版
中国語版、とても使いやすい

ゼンドスタジオ 13.0.1
強力な PHP 統合開発環境

ドリームウィーバー CS6
ビジュアル Web 開発ツール

SublimeText3 Mac版
神レベルのコード編集ソフト(SublimeText3)

ホットトピック
 7662
7662
 15
15
 1393
1393
 52
52
 1205
1205
 24
24
 91
91
 11
11
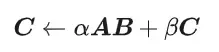 CUDA の汎用行列乗算: 入門から習熟まで!
Mar 25, 2024 pm 12:30 PM
CUDA の汎用行列乗算: 入門から習熟まで!
Mar 25, 2024 pm 12:30 PM
General Matrix Multiplication (GEMM) は、多くのアプリケーションやアルゴリズムの重要な部分であり、コンピューター ハードウェアのパフォーマンスを評価するための重要な指標の 1 つでもあります。 GEMM の実装に関する徹底的な調査と最適化は、ハイ パフォーマンス コンピューティングとソフトウェア システムとハードウェア システムの関係をより深く理解するのに役立ちます。コンピューター サイエンスでは、GEMM を効果的に最適化すると、計算速度が向上し、リソースが節約されます。これは、コンピューター システムの全体的なパフォーマンスを向上させるために非常に重要です。 GEMM の動作原理と最適化方法を深く理解することは、最新のコンピューティング ハードウェアの可能性をより有効に活用し、さまざまな複雑なコンピューティング タスクに対してより効率的なソリューションを提供するのに役立ちます。 GEMMのパフォーマンスを最適化することで
 Word文書で足し算、引き算、掛け算、割り算を計算する方法
Mar 19, 2024 pm 08:13 PM
Word文書で足し算、引き算、掛け算、割り算を計算する方法
Mar 19, 2024 pm 08:13 PM
WORD は強力なワード プロセッサです。Word を使用してさまざまなテキストを編集できます。Excel の表では、足し算、引き算、乗算の計算方法をマスターしました。そのため、Word の表で数値の足し算を計算する必要がある場合は、乗数を引くにはどうすればよいですか? 計算には電卓しか使用できませんか?答えはもちろん「いいえ」です。WORD でも実行できます。今日は、Word文書の表で加算、減算、乗算、除算などの基本的な演算を数式を使って計算する方法を説明しますので、一緒に学びましょう。そこで、今日は、WORD 文書で加算、減算、乗算、除算を計算する方法を詳しく説明します。ステップ 1: WORD を開き、ツールバーの [挿入] の下にある [表] をクリックし、ドロップダウン メニューに表を挿入します。
 Python の count() 関数を使用してリスト内の要素の数を数える方法
Nov 18, 2023 pm 02:53 PM
Python の count() 関数を使用してリスト内の要素の数を数える方法
Nov 18, 2023 pm 02:53 PM
Python の count() 関数を使用してリスト内の要素の数を計算する方法には、特定のコード サンプルが必要です。Python は強力で習得しやすいプログラミング言語として、さまざまなデータ構造を処理するための組み込み関数を多数提供しています。その 1 つは count() 関数で、リスト内の要素の数をカウントするために使用できます。この記事では、count()関数の使い方と具体的なコード例を詳しく説明します。 count() 関数は Python の組み込み関数であり、特定の値を計算するために使用されます。
 Java で部分文字列の出現数を再帰的にカウントする
Sep 17, 2023 pm 07:49 PM
Java で部分文字列の出現数を再帰的にカウントする
Sep 17, 2023 pm 07:49 PM
2 つの文字列 str_1 と str_2 を指定します。目的は、再帰的プロシージャを使用して、文字列 str1 内の部分文字列 str2 の出現数をカウントすることです。再帰関数は、その定義内で自分自身を呼び出す関数です。 str1 が「Iknowthatyouknowthatiknow」、str2 が「know」の場合、出現回数は -3 になります。例を通して理解しましょう。たとえば、入力 str1="TPisTPareTPamTP"、str2="TP"; 出力 Countofoccurrencesofasubstringrecursi
 C# で Math.Pow 関数を使用して指定した数値のべき乗を計算する方法
Nov 18, 2023 am 11:32 AM
C# で Math.Pow 関数を使用して指定した数値のべき乗を計算する方法
Nov 18, 2023 am 11:32 AM
C# には、多くの数学関数が含まれる Math クラス ライブラリがあります。これらには、累乗を計算する関数 Math.Pow が含まれており、指定された数値の累乗を計算するのに役立ちます。 Math.Pow 関数の使用法は非常に簡単で、基数と指数を指定するだけです。構文は次のとおりです: Math.Pow(base,exponent); ここで、base は基数を表し、exponent は指数を表します。この関数は double 型の結果、つまりべき乗の計算結果を返します。しましょう
 C プログラムは rename() 関数を使用してファイル名を変更します
Sep 21, 2023 pm 10:01 PM
C プログラムは rename() 関数を使用してファイル名を変更します
Sep 21, 2023 pm 10:01 PM
名前変更機能は、ファイルまたはディレクトリを古い名前から新しい名前に変更します。この操作は移動操作と似ています。したがって、この名前変更機能を使用してファイルを移動することもできます。この関数は、stdio.h ライブラリ ヘッダー ファイルに存在します。 rename 関数の構文は次のとおりです: intrename(constchar*oldname,constchar*newname); rename() 関数は 2 つのパラメータを受け取ります。 1 つは古い名前、もう 1 つは新しい名前です。どちらのパラメータも、ファイルの古い名前と新しい名前を定義する定数文字へのポインタです。ファイルの名前が正常に変更された場合はゼロを返し、それ以外の場合はゼロ以外の整数を返します。名前変更操作中
 指定された値を引数として受け取る逆双曲線正弦関数の値を見つける C++ プログラム
Sep 17, 2023 am 10:49 AM
指定された値を引数として受け取る逆双曲線正弦関数の値を見つける C++ プログラム
Sep 17, 2023 am 10:49 AM
双曲線関数は、円の代わりに双曲線を使用して定義され、通常の三角関数と同等です。ラジアン単位で指定された角度から双曲線正弦関数の比率パラメーターを返します。しかし、その逆、つまり別の言い方をすればいいのです。双曲線正弦から角度を計算したい場合は、双曲線逆正弦演算のような逆双曲線三角関数演算が必要です。このコースでは、C++ で双曲線逆サイン (asinh) 関数を使用し、ラジアン単位の双曲線サイン値を使用して角度を計算する方法を説明します。双曲線逆正弦演算は次の式に従います -$$\mathrm{sinh^{-1}x\:=\:In(x\:+\:\sqrt{x^2\:+\:1})}ここで\:In\:is\:自然対数\:(log_e\:k)
 辞書を印刷する C++ プログラム
Sep 11, 2023 am 10:33 AM
辞書を印刷する C++ プログラム
Sep 11, 2023 am 10:33 AM
マップは C++ の特別なタイプのコンテナで、各要素は 2 つの値、つまりキー値とマップ値のペアです。キー値は各項目のインデックス付けに使用され、マップされた値はキーに関連付けられた値です。マップされた値が一意であるかどうかに関係なく、キーは常に一意です。 C++ でマップ要素を出力するには、反復子を使用する必要があります。項目のセット内の要素は、反復子オブジェクトによって示されます。イテレータは主に配列や他のタイプのコンテナ (ベクトルなど) で使用され、特定の範囲内の特定の要素を識別するために使用できる特定の操作セットを備えています。イテレータをインクリメントまたはデクリメントして、範囲またはコンテナ内に存在するさまざまな要素を参照できます。イテレータは、範囲内の特定の要素のメモリ位置を指します。イテレータを使用して C++ でマップを出力する まず、定義方法を見てみましょう。




