A[]= { 0,0,1,1 } B[]= { 0,0,1,1 } C= {0,0,1,1}Required flips : 2
C++ で、次を中国語に翻訳します。 A と B の XOR 結果が C と等しくなるように、ビット 反転の最小数を計算します。

長さ N の 3 つのバイナリ シーケンス A、B、C が与えられます。各シーケンスは、 2 進数。私たちはそうではないことを見つけなければなりません。 A と B の XOR が C になるように、A と B のビットに必要なフリップの数。 XOR B は C になります。
まず、XOR 演算の真理値表を理解しましょう。
| XX | Y | XX XOR Y |
|---|---|---|
| 0 | 0 | |
| 1 | 1 | |
| 0 | 1 | |
| 1 | 0 |
- A[i]==B[i] および C[i]==0 の場合、反転は必要ありません。
- if A[i]= = B[i] かつ C[i]==1 の場合、A[i] または B[i] を反転して反転回数を 1
- If A[i]!=B[i] およびC[i]==0 の場合、A[i] または B[i] を反転してフリップ数を 1 ずつ増やします
- If A[i]!=B[i] and C[i]== 1、その後、裏返す必要はありません。
A[]= { 0,0,0,0 } B[]= { 1,0,1,0 } C= {1,1,1,1}ログイン後にコピー
出力A[]= { 0,0,0,0 } B[]= { 1,0,1,0 } C= {1,1,1,1}Required flips : 2
ログイン後にコピーログイン後にコピー
説明Required flips : 2
の中国語翻訳は次のとおりです:
説明A[0] xor B[0] 0 xor 1 = 1 C[0]=1 no flip
A[1] xor B[1] 0 xor 0 = 0 C[0]=1 flip count=1
A[2] xor B[2] 0 xor 1 = 1 C[0]=1 no flip
A[3] xor B[3] 0 xor 0 = 0 C[0]=1flip count=2
ログイン後にコピー
入力A[0] xor B[0] 0 xor 1 = 1 C[0]=1 no flip A[1] xor B[1] 0 xor 0 = 0 C[0]=1 flip count=1 A[2] xor B[2] 0 xor 1 = 1 C[0]=1 no flip A[3] xor B[3] 0 xor 0 = 0 C[0]=1flip count=2
A[]= { 0,0,1,1 } B[]= { 0,0,1,1 } C= {0,0,1,1}ログイン後にコピー
出力A[]= { 0,0,1,1 } B[]= { 0,0,1,1 } C= {0,0,1,1}Required flips : 2
ログイン後にコピーログイン後にコピー
説明Required flips : 2
の中国語翻訳は次のとおりです。
説明A[0] xor B[0] 0 xor 0 = 0 C[0]=0 no flip
A[1] xor B[1] 0 xor 0 = 0 C[0]=0 no flip
A[2] xor B[2] 1 xor 1 = 0 C[0]=1 flip count=1
A[3] xor B[3] 1 xor 1 = 0 C[0]=1 flip count=2
ログイン後にコピー
次のプログラムで使用されるメソッドは次のとおりですA[0] xor B[0] 0 xor 0 = 0 C[0]=0 no flip A[1] xor B[1] 0 xor 0 = 0 C[0]=0 no flip A[2] xor B[2] 1 xor 1 = 0 C[0]=1 flip count=1 A[3] xor B[3] 1 xor 1 = 0 C[0]=1 flip count=2
- # 配列 a[]、b[]、および c[] は、2 進数を格納するために使用されます。
< /p>
関数 FlipCount(int A[], int B[], int C[], int n) は、配列 a、b、c とその長さ n を次のように受け取ります。
C[] が A XOR B と等しくなるように、A[] または B[] のビットに必要なフリップの数を入力して返します。
B- 変数 count はフリップ数を表し、0 に初期化されます。
- for ループを使用して、i = 0 から i までセル内の各ビットを反復処理します。
- 各ビット A[i ] についてそしてB[i]。それらが等しく、C[i] が 1 の場合、カウントをインクリメントします。
- 各ビット A[i] および B[i]。それらが等しくなく、C[i] が 0 の場合、カウントをインクリメントします。
- 必要な結果の数を返します。
- 例
ライブ デモンストレーション
#include<bits/stdc++.h>
using namespace std;
int flipCount(int A[], int B[], int C[], int N){
int count = 0;
for (int i=0; i < N; ++i){
// If both A[i] and B[i] are equal then XOR results 0, if C[i] is 1 flip
if (A[i] == B[i] && C[i] == 1)
++count;
// If Both A and B are unequal then XOR results 1 , if C[i] is 0 flip
else if (A[i] != B[i] && C[i] == 0)
++count;
}
return count;
}
int main(){
//N represent total count of Bits
int N = 5;
int a[] ={1,0,0,0,0};
int b[] ={0,0,0,1,0};
int c[] ={1,0,1,1,1};
cout <<"Minimum bits to flip such that XOR of A and B equal to C :"<<flipCount(a, b, c,N);
return 0;
}出力
Minimum bits to flip such that XOR of A and B equal to C :2
以上がC++ で、次を中国語に翻訳します。 A と B の XOR 結果が C と等しくなるように、ビット 反転の最小数を計算します。の詳細内容です。詳細については、PHP 中国語 Web サイトの他の関連記事を参照してください。

ホットAIツール

Undresser.AI Undress
リアルなヌード写真を作成する AI 搭載アプリ

AI Clothes Remover
写真から衣服を削除するオンライン AI ツール。

Undress AI Tool
脱衣画像を無料で

Clothoff.io
AI衣類リムーバー

AI Hentai Generator
AIヘンタイを無料で生成します。

人気の記事

ホットツール

メモ帳++7.3.1
使いやすく無料のコードエディター

SublimeText3 中国語版
中国語版、とても使いやすい

ゼンドスタジオ 13.0.1
強力な PHP 統合開発環境

ドリームウィーバー CS6
ビジュアル Web 開発ツール

SublimeText3 Mac版
神レベルのコード編集ソフト(SublimeText3)

ホットトピック
 7466
7466
 15
15
 1376
1376
 52
52
 77
77
 11
11
 18
18
 19
19
 C 言語の定数とは何ですか?例を挙げていただけますか?
Aug 28, 2023 pm 10:45 PM
C 言語の定数とは何ですか?例を挙げていただけますか?
Aug 28, 2023 pm 10:45 PM
定数は変数とも呼ばれ、一度定義されると、その値はプログラムの実行中に変更されません。したがって、変数を固定値を参照する定数として宣言できます。テキストとも呼ばれます。定数は、Const キーワードを使用して定義する必要があります。構文 C プログラミング言語で使用される定数の構文は次のとおりです - consttypeVariableName; (または) consttype*VariableName; さまざまなタイプの定数 C プログラミング言語で使用されるさまざまなタイプの定数は次のとおりです: 整数定数 - 例: 1,0 、34、4567 浮動小数点定数 - 例: 0.0、156.89、23.456 8 進数および 16 進数の定数 - 例: 16 進数: 0x2a、0xaa.. 8 進数
 VSCode および VS C++ IntelliSense が機能しない、またはライブラリを選択しない
Feb 29, 2024 pm 01:28 PM
VSCode および VS C++ IntelliSense が機能しない、またはライブラリを選択しない
Feb 29, 2024 pm 01:28 PM
VS Code および Visual Studio C++ IntelliSense は、特に大規模なプロジェクトで作業している場合、ライブラリを選択できない場合があります。 #Include<wx/wx.h> の上にマウスを移動すると、「ソース ファイル 'string.h' を開けません」というエラー メッセージが表示され (「wx/wx.h」に応じて異なります)、オートコンプリート関数が応答しなくなることがあります。この記事では、VSCode および VSC++ IntelliSense が機能しない場合、またはライブラリを抽出できない場合の対処法を説明します。私のインテリセンスが C++ で動作しないのはなぜですか?大きなファイルを扱う場合、IntelliSense が機能しないことがあります。
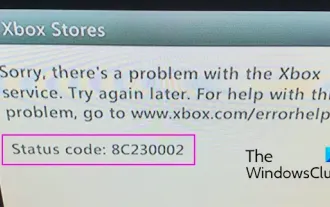 Xboxエラーコード8C230002を修正
Feb 27, 2024 pm 03:55 PM
Xboxエラーコード8C230002を修正
Feb 27, 2024 pm 03:55 PM
エラー コード 8C230002 が原因で、Xbox でコンテンツを購入または視聴できませんか?一部のユーザーは、本体でコンテンツを購入または視聴しようとすると、引き続きこのエラーが発生します。申し訳ありませんが、Xbox サービスに問題があります。後でもう一度お試しください。この問題のヘルプが必要な場合は、www.xbox.com/errorhelp にアクセスしてください。ステータス コード: 8C230002 このエラー コードは通常、サーバーまたはネットワークの一時的な問題によって発生します。ただし、アカウントのプライバシー設定や保護者による制限など、他の理由により、特定のコンテンツの購入または表示が妨げられる場合があります。 Xbox エラー コード 8C230002 を修正する Xbox 本体でコンテンツを視聴または購入しようとしたときにエラー コード 8C が表示された場合
 C++ で配列の最小要素と最大要素を見つける再帰的プログラム
Aug 31, 2023 pm 07:37 PM
C++ で配列の最小要素と最大要素を見つける再帰的プログラム
Aug 31, 2023 pm 07:37 PM
整数配列 Arr[] を入力として受け取ります。目標は、再帰的メソッドを使用して配列内の最大要素と最小要素を見つけることです。再帰を使用しているため、長さ = 1 に達するまで配列全体を反復処理し、基本ケースを形成する A[0] を返します。それ以外の場合、現在の要素は現在の最小値または最大値と比較され、その値は後続の要素に対して再帰的に更新されます。この場合のさまざまな入出力シナリオを見てみましょう −入力 −Arr={12,67,99,76,32}; 出力 −配列内の最大値: 99 説明 &mi
 中国東方航空、C919旅客機が間もなく実運用に入ると発表
May 28, 2023 pm 11:43 PM
中国東方航空、C919旅客機が間もなく実運用に入ると発表
May 28, 2023 pm 11:43 PM
5月25日のニュースによると、中国東方航空は性能説明会でC919旅客機の最新の進捗状況を明らかにした。同社によると、COMACと締結したC919購入契約は2021年3月に正式に発効し、最初のC919航空機は2022年末までに引き渡される予定だという。近く正式に実運用が開始される見通しだ。中国東方航空は上海をC919の商業運航の主拠点とし、2022年と2023年に計5機のC919旅客機を導入する計画だ。同社は、今後の導入計画については、運行実態や路線網計画を踏まえて決定するとしている。編集者の理解によれば、C919は世界で完全に独立した知的財産権を有する中国の新世代の単通路本線旅客機であり、国際的に認められた耐空基準に準拠している。すべき
 数字の螺旋パターンを出力する C++ プログラム
Sep 05, 2023 pm 06:25 PM
数字の螺旋パターンを出力する C++ プログラム
Sep 05, 2023 pm 06:25 PM
数値をさまざまな形式で表示することは、学習における基本的なコーディング問題の 1 つです。条件文やループ文などのさまざまなコーディング概念。アスタリスクなどの特殊文字を使用して三角形や四角形を印刷するさまざまなプログラムがあります。この記事では、C++ の正方形と同じように、数値をスパイラル形式で出力します。行数 n を入力として受け取り、左上隅から開始して右、次に下、次に左、次に上、そして再び右、というように移動します。数字付きスパイラル パターン 123456724252627282982340414243309223948494431102138474645321120373635343312191817161514
 23 年ぶりに C# が TIOBE 2023 年間最優秀プログラミング言語賞を受賞
Jan 11, 2024 pm 04:45 PM
23 年ぶりに C# が TIOBE 2023 年間最優秀プログラミング言語賞を受賞
Jan 11, 2024 pm 04:45 PM
プログラミング言語の人気を測るベンチマークの 1 つである TIOBE プログラミング コミュニティ インデックスによると、世界中のエンジニア、コース、ベンダー、検索エンジンからデータを収集することによって評価されています。先日、2024年1月のTIOBE Indexが発表され、2023年の公式プログラミング言語ランキングが発表され、C#がTIOBE 2023 Programming Language of the Yearを受賞し、23年ぶりにC#がこの栄誉を獲得しました。 TIOBE の公式プレスリリースによると、C# は 20 年以上トップ 10 内にランクインしていましたが、現在では主要 4 言語に追いつき、1 年間で最大の成長率 (+1.43%) を示したプログラミング言語となっています。この賞を受賞するのは当然のことです。 2 位は Scratch (+0.83%) と Fortran (+0)
 C言語におけるvoidキーワードの機能
Feb 19, 2024 pm 11:33 PM
C言語におけるvoidキーワードの機能
Feb 19, 2024 pm 11:33 PM
C の void は、空の型、つまり特定の型を持たないデータを表すために使用される特別なキーワードです。 C言語ではvoidは主に以下の3つの場面で使われます。関数の戻り値の型は void です。C 言語では、関数は int、float、char などのさまざまな戻り値の型を持つことができます。ただし、関数が値を返さない場合は、戻り値の型を void に設定できます。これは、関数が実行された後、特定の値を返さないことを意味します。例: voidhelloWorld()




