C#의 피보나치 수열
피보나치 시리즈 중 C#의 피보나치 시리즈는 유명한 시퀀스 시리즈 중 하나입니다. 순서는 0, 1, 1, 2, 3, 5, 8… 피보나치 수열은 0과 1부터 시작하며 다음 숫자는 이전 두 숫자의 합입니다. 피보나치 수열은 13세기세기에 레오나르도 피사노 비골로(Leonardo Pisano Bigollo)가 창안했다고 합니다. 피보나치 수열은 일부 시나리오에 유용합니다. 기본적으로 이것은 원래 토끼 문제, 즉 한 쌍에서 태어난 토끼의 수를 해결하는 데 사용되었습니다. 피보나치 수열이 유용한 다른 문제도 있습니다.
피보나치 수열 논리
피보나치 수열과 마찬가지로 숫자는 이전 두 숫자의 합입니다. 따라서 피보나치 수열이 0, 1, 1, 2, 3, 5, 8, 13, 21이라고 말하면… 이 다음 숫자에 따르면 13과 21과 같은 이전 두 숫자의 합이 됩니다. 따라서 다음 숫자는 13입니다. +21=34.
피보나치 수열을 생성하는 논리는 다음과 같습니다
F(n)= F(n-1) +F(n-2)
F(n)은 항 번호이고 F(n-1) +F(n-2)는 이전 값의 합입니다.
그래서 시리즈 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89…
논리에 따르면 F(n)= F(n-1) +F(n-2)
F(n)= 55+89
F(n)= 144
다음 학기는 144입니다.
피보나치 수열을 만드는 다양한 방법
피보나치 수열은 다양한 방법으로 생성할 수 있습니다.
1. 반복적 접근
이 방법이 시리즈를 생성하는 가장 쉬운 방법입니다.
코드:
using System;
using System.Collections.Generic;
using System.Linq;
using System.Text;
namespaceFibonacciDemo
{
classProgram
{
staticint Fibonacci(int n)
{
intfirstnumber = 0, secondnumber = 1, result = 0;
if (n == 0) return 0; //It will return the first number of the series
if (n == 1) return 1; // it will return the second number of the series
for (int i = 2; i<= n; i++) // main processing starts from here
{
result = firstnumber + secondnumber;
firstnumber = secondnumber;
secondnumber = result;
}
return result;
}
staticvoid Main(string[] args)
{
Console.Write("Length of the Fibonacci Series: ");
int length = Convert.ToInt32(Console.ReadLine());
for(int i = 0; i< length; i++)
{
Console.Write("{0} ", Fibonacci(i));
}
Console.ReadKey();
}
}
}2. 재귀적 방법
이 문제를 해결하는 또 다른 방법입니다.
방법 1
using System;
using System.Collections.Generic;
using System.Linq;
using System.Text;
namespaceFibonacciDemo
{
classProgram
{
staticint Fibonacci(int n)
{
intfirstnumber = 0, secondnumber = 1, result = 0;
if (n == 0) return 0; //it will return the first number of the series
if (n == 1) return 1; // it will return the second number of the series
return Fibonacci(n-1) + Fibonacci(n-2);
}
staticvoid Main(string[] args)
{
Console.Write("Length of the Fibonacci Series: ");
int length = Convert.ToInt32(Console.ReadLine());
for(int i = 0; i< length; i++)
{
Console.Write("{0} ", Fibonacci(i));
}
Console.ReadKey();
}
}
}방법 2
using System.Collections.Generic;
using System.Linq;
using System.Text;
namespace FibonacciSeries
{
class Program
{
public static void Fibonacci
(
int firstnumber,
int secondnumber,
int count,
int length,
)
{
if (count <= length)
{
Console.Write("{0} ", firstnumber);
Fibonacci(secondnumber, firstnumber + secondnumber, count + 1, length);
}
}
public static void Main(string[] args)
{
Console.Write("Length of the Fibonacci Series: ");
int length = Convert.ToInt32(Console.ReadLine());
Fibonacci(0, 1, 1, length);
Console.ReadKey();
}
}
}출력:
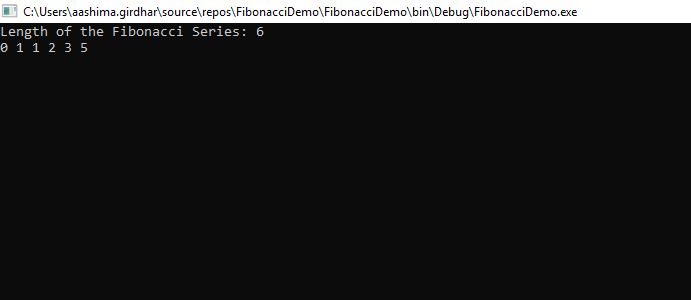
3. 배열을 이용한 피보나치
코드:
using System;
using System.Collections.Generic;
using System.Linq;
using System.Text;
public class Program
{
public static int[] Fibonacci(int number)
{
int[] a = new int[number];
a[0] = 0;
a[1] = 1;
for (int i = 2; i < number; i++)
{
a[i] = a[i - 2] + a[i - 1];
}
return a;
}
public static void Main(string[] args)
{
var b = Fibonacci(10);
foreach (var elements in b)
{
Console.WriteLine(elements);
}
}
}출력:
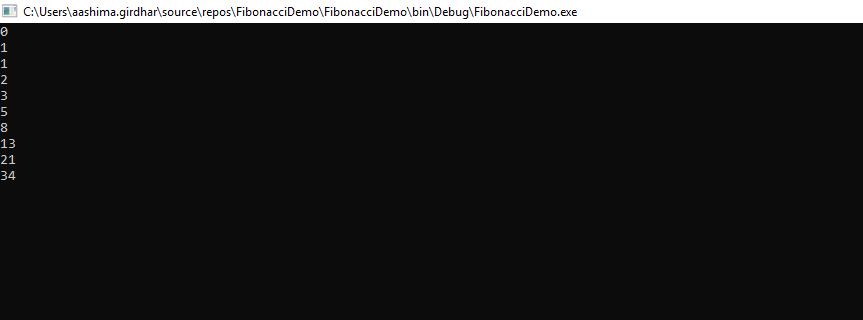
피보나치 수열의 N번째 항을 찾는 방법은 무엇인가요?
방법은 다음과 같습니다
방법 1
코드:
using System;
namespace FibonacciSeries
{
class Program {
public static int NthTerm(int n)
{
if ((n == 0) || (n == 1))
{
return n;
}
else
{
return (NthTerm(n - 1) + NthTerm(n - 2));
}
}
public static void Main(string[] args)
{
Console.Write("Enter the nth term of the Fibonacci Series: ");
int number = Convert.ToInt32(Console.ReadLine());
number = number - 1;
Console.Write(NthTerm(number));
Console.ReadKey();
}
}
}위 코드는 피보나치 수열의 n번째 항을 찾는 코드입니다. 예를 들어, 시리즈에서 12번째 용어를 찾으려면 결과는 89가 됩니다.
방법 2
(O(Log t) 시간).
t번째 피보나치 수를 찾는 데 사용할 수 있는 또 다른 반복 공식이 있습니다. t가 짝수이면 = t/2:
F(t) = [2*F(k-1) + F(k)]*F(k)
t가 홀수이면 k = (t + 1)/2
F(t) = F(k)*F(k) + F(k-1)*F(k-1)
피보나치 행렬
행렬을 얻은 후에는 (-1)t = Ft+1Ft-1 – Ft2를 얻습니다.
FmFt + Fm-1Ft-1 = Fm+t-1
t = t+1이라고 하면
FmFt+1 + Fm-1Ft = Fm+t
m = t
F2t-1 = Ft2 + Ft-12
F2t = (Ft-1 + Ft+1)Ft = (2Ft-1 + Ft)Ft
공식을 얻기 위해 다음을 수행합니다
t가 짝수이면 k = t/2를 입력하세요
t가 홀수이면 k = (t+1)/2를 입력하세요
이러한 숫자를 정렬하면 STACK의 메모리 공간이 지속적으로 사용되는 것을 방지할 수 있습니다. 이는 O(n)의 시간 복잡도를 제공합니다. 재귀 알고리즘은 효율성이 떨어집니다.
코드:
int f(n) : if( n==0 || n==1 ) return n; else return f(n-1) + f(n-2)
이제 위의 알고리즘이 n=4에 대해 실행될 때
fn(4)
f(3) f(2)
f(2) f(1) f(1) f(0)
f(1) f(0)
그럼 나무군요. f(4)를 계산하려면 f(3)과 f(2) 등을 계산해야 합니다. 4라는 작은 값의 경우 f(2)는 두 번 계산되고 f(1)은 세 번 계산됩니다. 이 추가 항목 수는 대량으로 증가할 것입니다.
f(n)을 계산하는데 필요한 덧셈의 개수는 f(n+1)-1이라는 추측이 있습니다.
결론
여기서 반복 방법은 이러한 종류의 문제를 해결하는 데 더 빠른 접근 방식을 제공하므로 항상 선호됩니다. 여기서는 이전 숫자와 이전 숫자(두 개의 변수)에 피보나치 수열의 첫 번째와 두 번째 숫자를 저장하고 또한 현재 숫자를 사용하여 피보나치 수를 저장합니다.
위 내용은 C#의 피보나치 수열의 상세 내용입니다. 자세한 내용은 PHP 중국어 웹사이트의 기타 관련 기사를 참조하세요!

핫 AI 도구

Undresser.AI Undress
사실적인 누드 사진을 만들기 위한 AI 기반 앱

AI Clothes Remover
사진에서 옷을 제거하는 온라인 AI 도구입니다.

Undress AI Tool
무료로 이미지를 벗다

Clothoff.io
AI 옷 제거제

Video Face Swap
완전히 무료인 AI 얼굴 교환 도구를 사용하여 모든 비디오의 얼굴을 쉽게 바꾸세요!

인기 기사

뜨거운 도구

메모장++7.3.1
사용하기 쉬운 무료 코드 편집기

SublimeText3 중국어 버전
중국어 버전, 사용하기 매우 쉽습니다.

스튜디오 13.0.1 보내기
강력한 PHP 통합 개발 환경

드림위버 CS6
시각적 웹 개발 도구

SublimeText3 Mac 버전
신 수준의 코드 편집 소프트웨어(SublimeText3)

뜨거운 주제
 7913
7913
 15
15
 1652
1652
 14
14
 1411
1411
 52
52
 1303
1303
 25
25
 1248
1248
 29
29
 C#을 사용한 Active Directory
Sep 03, 2024 pm 03:33 PM
C#을 사용한 Active Directory
Sep 03, 2024 pm 03:33 PM
C#을 사용한 Active Directory 가이드. 여기에서는 소개와 구문 및 예제와 함께 C#에서 Active Directory가 작동하는 방식에 대해 설명합니다.
 C#의 난수 생성기
Sep 03, 2024 pm 03:34 PM
C#의 난수 생성기
Sep 03, 2024 pm 03:34 PM
C#의 난수 생성기 가이드입니다. 여기서는 난수 생성기의 작동 방식, 의사 난수 및 보안 숫자의 개념에 대해 설명합니다.
 C# 데이터 그리드 보기
Sep 03, 2024 pm 03:32 PM
C# 데이터 그리드 보기
Sep 03, 2024 pm 03:32 PM
C# 데이터 그리드 뷰 가이드. 여기서는 SQL 데이터베이스 또는 Excel 파일에서 데이터 그리드 보기를 로드하고 내보내는 방법에 대한 예를 설명합니다.
 멀티 스레딩과 비동기 C#의 차이
Apr 03, 2025 pm 02:57 PM
멀티 스레딩과 비동기 C#의 차이
Apr 03, 2025 pm 02:57 PM
멀티 스레딩과 비동기식의 차이점은 멀티 스레딩이 동시에 여러 스레드를 실행하는 반면, 현재 스레드를 차단하지 않고 비동기식으로 작업을 수행한다는 것입니다. 멀티 스레딩은 컴퓨팅 집약적 인 작업에 사용되며 비동기식은 사용자 상호 작용에 사용됩니다. 멀티 스레딩의 장점은 컴퓨팅 성능을 향상시키는 것이지만 비동기의 장점은 UI 스레드를 차단하지 않는 것입니다. 멀티 스레딩 또는 비동기식을 선택하는 것은 작업의 특성에 따라 다릅니다. 계산 집약적 작업은 멀티 스레딩을 사용하고 외부 리소스와 상호 작용하고 UI 응답 성을 비동기식으로 유지 해야하는 작업을 사용합니다.








