Java의 이진 트리에 대한 기본 지식과 개념은 무엇입니까?
1. 트리 구조
1.1 개념
트리는 n(n>=0)개의 제한된 노드로 구성된 계층적 관계의 집합인 비선형 데이터 구조입니다. 거꾸로 된 나무, 즉 뿌리가 위를 향하고 잎이 아래를 향하고 있기 때문에 나무라고 불립니다.
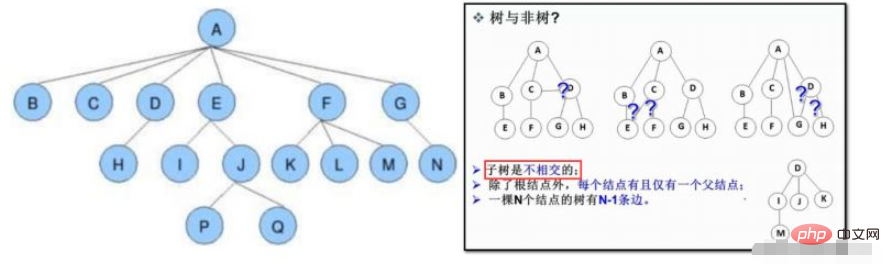
1.2 개념(중요)
a. 노드의 차수: 위와 같이 노드의 하위 트리 수: A의 차수는 6, J의 차수는 2
b입니다. 트리의 차수: 트리의 수, 가장 큰 노드의 차수는 위 그림에 표시된 대로 수의 차수입니다. 트리의 차수는 6
c입니다. 차수가 0인 노드(하위 트리가 없는 노드)
d. 부모 매듭 점/부모 노드: 위 그림과 같이: D는 H
의 부모 노드입니다. 자식 노드/자식 노드: 위 그림과 같습니다. : H는 D
e의 하위 노드입니다. 루트 노드: 위 그림과 같이 부모가 없는 노드: A
f. 노드의 수준: 루트 정의부터 시작합니다. 첫 번째 수준, 루트의 하위 노드는 두 번째 수준입니다.
g. 트리의 높이 또는 깊이: 위에 표시된 대로 트리의 최대 수준입니다. 4
2. 이진 트리(핵심)
2.1 개념
각 노드는 최대 2개의 하위 트리, 차수
2.2 이진 트리의 기본 형태
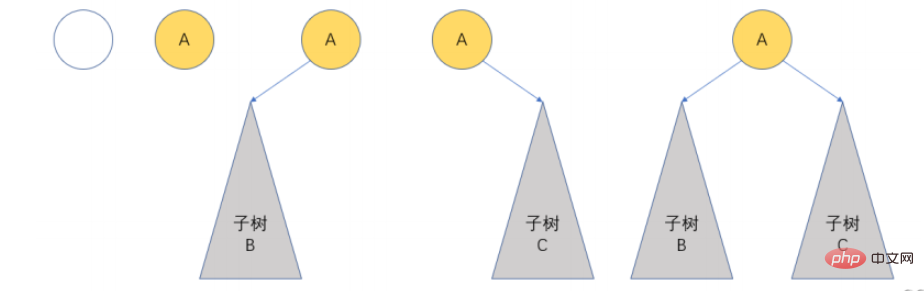
2.3 두 가지 유형 특수 이진 트리

a. 비자엽도는 2
b. 완전 이진 트리: 전체 이진 트리에 "오른쪽 하단 모서리"가 없습니다.
2.4 이진 트리의 속성
a. tree
1. 높이가 K이면 2^k-1개의 노드가 있습니다
2. 레벨이 K이면 레이어에 2^(k-1)개의 노드가 있습니다
3. 노드 수 - 1
4차수가 0인 n0과 2차가 있는 n2가 있으며, n0 = n2 + 1
b입니다. 올바른 자식이 있으면 반드시 있어야 합니다. 왼쪽 자식
2. 차수가 1인 노드는 하나만 있을 수 있습니다.2.5 이진 트리의 저장 이진 트리의 저장 구조는 연결 목록과 유사한 순차 저장과 연결 저장으로 나뉩니다. 순차 저장소: 완전한 이진 트리만 저장할 수 있습니다. 체인 저장소: 일반 이진 트리 이번에는 체인 저장소를 보여줍니다. 이진 트리의 체인 저장소는 노드별로 하나씩 참조됩니다. 삼항 표현,이 그림을 예로 들면, 세부 사항은 다음과 같습니다: 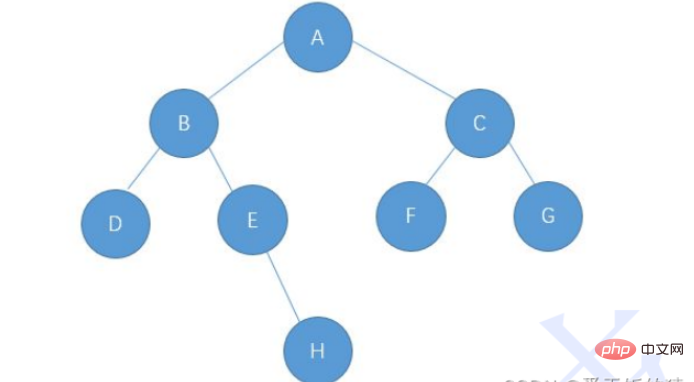
// 孩子表示法
private static class TreeNode{
char val;
TreeNode left;
TreeNode right;
public TreeNode(char val) {
this.val = val;
}
} public static TreeNode build(){
TreeNode nodeA=new TreeNode('A');
TreeNode nodeB=new TreeNode('B');
TreeNode nodeC=new TreeNode('C');
TreeNode nodeD=new TreeNode('D');
TreeNode nodeE=new TreeNode('E');
TreeNode nodeF=new TreeNode('F');
TreeNode nodeG=new TreeNode('G');
TreeNode nodeH=new TreeNode('H');
nodeA.left=nodeB;
nodeA.right=nodeC;
nodeB.left=nodeD;
nodeB.right=nodeE;
nodeE.right=nodeH;
nodeC.left=nodeF;
nodeC.right=nodeG;
return nodeA;
} //先序遍历 : 根左右
public static void preOrder(TreeNode root){
if(root==null){
return;
}
System.out.print(root.val+" ");
preOrder(root.left);
preOrder(root.right);
} //中序遍历
public static void inOrder(TreeNode root){
if(root==null){
return;
}
preOrder(root.left);
System.out.print(root.val+" ");
preOrder(root.right);
} //后序遍历
public static void postOrder(TreeNode root){
if(root==null){
return;
}
preOrder(root.left);
preOrder(root.right);
System.out.print(root.val+" ");
} //方法2(迭代)
//先序遍历 (迭代)
public static void preOrderNonRecursion(TreeNode root){
if(root==null){
return ;
}
Deque<TreeNode> stack=new LinkedList<>();
stack.push(root);
while (!stack.isEmpty()){
TreeNode cur=stack.pop();
System.out.print(cur.val+" ");
if(cur.right!=null){
stack.push(cur.right);
}
if(cur.left!=null){
stack.push(cur.left);
}
}
} //方法2(迭代)
//中序遍历 (迭代)
public static void inorderTraversalNonRecursion(TreeNode root) {
if(root==null){
return ;
}
Deque<TreeNode> stack=new LinkedList<>();
// 当前走到的节点
TreeNode cur=root;
while (!stack.isEmpty() || cur!=null){
// 不管三七二十一,先一路向左走到根儿~
while (cur!=null){
stack.push(cur);
cur=cur.left;
}
// 此时cur为空,说明走到了null,此时栈顶就存放了左树为空的节点
cur=stack.pop();
System.out.print(cur.val+" ");
// 继续访问右子树
cur=cur.right;
}
} //方法2(迭代)
//后序遍历 (迭代)
public static void postOrderNonRecursion(TreeNode root){
if(root==null){
return;
}
Deque<TreeNode> stack=new LinkedList<>();
TreeNode cur=root;
TreeNode prev=null;
while (!stack.isEmpty() || cur!=null){
while (cur!=null){
stack.push(cur);
cur=cur.left;
}
cur=stack.pop();
if(cur.right==null || prev==cur.right){
System.out.print(cur.val+" ");
prev=cur;
cur=null;
}else {
stack.push(cur);
cur=cur.right;
}
}
} //方法1(递归)
//传入一颗二叉树的根节点,就能统计出当前二叉树中一共有多少个节点,返回节点数
//此时的访问就不再是输出节点值,而是计数器 + 1操作
public static int getNodes(TreeNode root){
if(root==null){
return 0;
}
return 1+getNodes(root.left)+getNodes(root.right);
}
//方法2(迭代)
//使用层序遍历来统计当前树中的节点个数
public static int getNodesNoRecursion(TreeNode root){
if(root==null){
return 0;
}
int size=0;
Deque<TreeNode> queue=new LinkedList<>();
queue.offer(root);
while (!queue.isEmpty()) {
TreeNode cur = queue.poll();
size++;
if (cur.left != null) {
queue.offer(cur.left);
}
if (cur.right != null) {
queue.offer(cur.right);
}
}
return size;
} //方法1(递归)
//传入一颗二叉树的根节点,就能统计出当前二叉树的叶子结点个数
public static int getLeafNodes(TreeNode root){
if(root==null){
return 0;
}
if(root.left==null && root.right==null){
return 1;
}
return getLeafNodes(root.left)+getLeafNodes(root.right);
}
//方法2(迭代)
//使用层序遍历来统计叶子结点的个数
public static int getLeafNodesNoRecursion(TreeNode root){
if(root==null){
return 0;
}
int size=0;
Deque<TreeNode> queue=new LinkedList<>();
queue.offer(root);
while (!queue.isEmpty()){
TreeNode cur=queue.poll();
if(cur.left==null && cur.right==null){
size++;
}
if(cur.left!=null){
queue.offer(cur.left);
}
if(cur.right!=null){
queue.offer(cur.right);
}
}
return size;
} //求出以root为根节点的二叉树第k层的节点个数
public static int getKLevelNodes(TreeNode root,int k){
if(root==null || k<=0){
return 0;
}
if(k==1){
return 1;
}
return getKLevelNodes(root.left,k-1)+getKLevelNodes(root.right,k-1);
} //传入一个以root为根节点的二叉树,就能求出该树的高度
public static int height(TreeNode root){
if(root==null){
return 0;
}
return 1+ Math.max(height(root.left),height(root.right));
}위 내용은 Java의 이진 트리에 대한 기본 지식과 개념은 무엇입니까?의 상세 내용입니다. 자세한 내용은 PHP 중국어 웹사이트의 기타 관련 기사를 참조하세요!

핫 AI 도구

Undresser.AI Undress
사실적인 누드 사진을 만들기 위한 AI 기반 앱

AI Clothes Remover
사진에서 옷을 제거하는 온라인 AI 도구입니다.

Undress AI Tool
무료로 이미지를 벗다

Clothoff.io
AI 옷 제거제

Video Face Swap
완전히 무료인 AI 얼굴 교환 도구를 사용하여 모든 비디오의 얼굴을 쉽게 바꾸세요!

인기 기사

뜨거운 도구

메모장++7.3.1
사용하기 쉬운 무료 코드 편집기

SublimeText3 중국어 버전
중국어 버전, 사용하기 매우 쉽습니다.

스튜디오 13.0.1 보내기
강력한 PHP 통합 개발 환경

드림위버 CS6
시각적 웹 개발 도구

SublimeText3 Mac 버전
신 수준의 코드 편집 소프트웨어(SublimeText3)
 Java 8 Stream foreach에서 나누거나 돌아 오시겠습니까?
Feb 07, 2025 pm 12:09 PM
Java 8 Stream foreach에서 나누거나 돌아 오시겠습니까?
Feb 07, 2025 pm 12:09 PM
Java 8은 스트림 API를 소개하여 데이터 컬렉션을 처리하는 강력하고 표현적인 방법을 제공합니다. 그러나 스트림을 사용할 때 일반적인 질문은 다음과 같은 것입니다. 기존 루프는 조기 중단 또는 반환을 허용하지만 스트림의 Foreach 메소드는이 방법을 직접 지원하지 않습니다. 이 기사는 이유를 설명하고 스트림 처리 시스템에서 조기 종료를 구현하기위한 대체 방법을 탐색합니다. 추가 읽기 : Java Stream API 개선 스트림 foreach를 이해하십시오 Foreach 메소드는 스트림의 각 요소에서 하나의 작업을 수행하는 터미널 작동입니다. 디자인 의도입니다
 PHP : 웹 개발의 핵심 언어
Apr 13, 2025 am 12:08 AM
PHP : 웹 개발의 핵심 언어
Apr 13, 2025 am 12:08 AM
PHP는 서버 측에서 널리 사용되는 스크립팅 언어이며 특히 웹 개발에 적합합니다. 1.PHP는 HTML을 포함하고 HTTP 요청 및 응답을 처리 할 수 있으며 다양한 데이터베이스를 지원할 수 있습니다. 2.PHP는 강력한 커뮤니티 지원 및 오픈 소스 리소스를 통해 동적 웹 컨텐츠, 프로세스 양식 데이터, 액세스 데이터베이스 등을 생성하는 데 사용됩니다. 3. PHP는 해석 된 언어이며, 실행 프로세스에는 어휘 분석, 문법 분석, 편집 및 실행이 포함됩니다. 4. PHP는 사용자 등록 시스템과 같은 고급 응용 프로그램을 위해 MySQL과 결합 할 수 있습니다. 5. PHP를 디버깅 할 때 error_reporting () 및 var_dump ()와 같은 함수를 사용할 수 있습니다. 6. 캐싱 메커니즘을 사용하여 PHP 코드를 최적화하고 데이터베이스 쿼리를 최적화하며 내장 기능을 사용하십시오. 7
 PHP vs. Python : 차이점 이해
Apr 11, 2025 am 12:15 AM
PHP vs. Python : 차이점 이해
Apr 11, 2025 am 12:15 AM
PHP와 Python은 각각 고유 한 장점이 있으며 선택은 프로젝트 요구 사항을 기반으로해야합니다. 1.PHP는 간단한 구문과 높은 실행 효율로 웹 개발에 적합합니다. 2. Python은 간결한 구문 및 풍부한 라이브러리를 갖춘 데이터 과학 및 기계 학습에 적합합니다.
 PHP 대 기타 언어 : 비교
Apr 13, 2025 am 12:19 AM
PHP 대 기타 언어 : 비교
Apr 13, 2025 am 12:19 AM
PHP는 특히 빠른 개발 및 동적 컨텐츠를 처리하는 데 웹 개발에 적합하지만 데이터 과학 및 엔터프라이즈 수준의 애플리케이션에는 적합하지 않습니다. Python과 비교할 때 PHP는 웹 개발에 더 많은 장점이 있지만 데이터 과학 분야에서는 Python만큼 좋지 않습니다. Java와 비교할 때 PHP는 엔터프라이즈 레벨 애플리케이션에서 더 나빠지지만 웹 개발에서는 더 유연합니다. JavaScript와 비교할 때 PHP는 백엔드 개발에서 더 간결하지만 프론트 엔드 개발에서는 JavaScript만큼 좋지 않습니다.
 PHP vs. Python : 핵심 기능 및 기능
Apr 13, 2025 am 12:16 AM
PHP vs. Python : 핵심 기능 및 기능
Apr 13, 2025 am 12:16 AM
PHP와 Python은 각각 고유 한 장점이 있으며 다양한 시나리오에 적합합니다. 1.PHP는 웹 개발에 적합하며 내장 웹 서버 및 풍부한 기능 라이브러리를 제공합니다. 2. Python은 간결한 구문과 강력한 표준 라이브러리가있는 데이터 과학 및 기계 학습에 적합합니다. 선택할 때 프로젝트 요구 사항에 따라 결정해야합니다.
 캡슐의 양을 찾기위한 Java 프로그램
Feb 07, 2025 am 11:37 AM
캡슐의 양을 찾기위한 Java 프로그램
Feb 07, 2025 am 11:37 AM
캡슐은 3 차원 기하학적 그림이며, 양쪽 끝에 실린더와 반구로 구성됩니다. 캡슐의 부피는 실린더의 부피와 양쪽 끝에 반구의 부피를 첨가하여 계산할 수 있습니다. 이 튜토리얼은 다른 방법을 사용하여 Java에서 주어진 캡슐의 부피를 계산하는 방법에 대해 논의합니다. 캡슐 볼륨 공식 캡슐 볼륨에 대한 공식은 다음과 같습니다. 캡슐 부피 = 원통형 볼륨 2 반구 볼륨 안에, R : 반구의 반경. H : 실린더의 높이 (반구 제외). 예 1 입력하다 반경 = 5 단위 높이 = 10 단위 산출 볼륨 = 1570.8 입방 단위 설명하다 공식을 사용하여 볼륨 계산 : 부피 = π × r2 × h (4
 PHP의 영향 : 웹 개발 및 그 이상
Apr 18, 2025 am 12:10 AM
PHP의 영향 : 웹 개발 및 그 이상
Apr 18, 2025 am 12:10 AM
phphassignificallyimpactedwebdevelopmentandextendsbeyondit
 PHP : 많은 웹 사이트의 기초
Apr 13, 2025 am 12:07 AM
PHP : 많은 웹 사이트의 기초
Apr 13, 2025 am 12:07 AM
PHP가 많은 웹 사이트에서 선호되는 기술 스택 인 이유에는 사용 편의성, 강력한 커뮤니티 지원 및 광범위한 사용이 포함됩니다. 1) 배우고 사용하기 쉽고 초보자에게 적합합니다. 2) 거대한 개발자 커뮤니티와 풍부한 자원이 있습니다. 3) WordPress, Drupal 및 기타 플랫폼에서 널리 사용됩니다. 4) 웹 서버와 밀접하게 통합하여 개발 배포를 단순화합니다.






