직각삼각형인 정사각형에 새겨진 가장 큰 루에로삼각형은 무엇입니까?
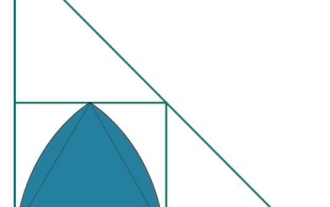
여기서 우리는 정사각형에 내접된 가장 큰 Reuleaux 삼각형의 면적을 볼 수 있으며, 이는 다시 직각 삼각형에 내접됩니다. 정사각형의 한 변의 길이는 'a'입니다. Reuleaux 삼각형의 높이는 x입니다. 삼각형의 밑변은 b, 높이는 l, 빗변은 h입니다.
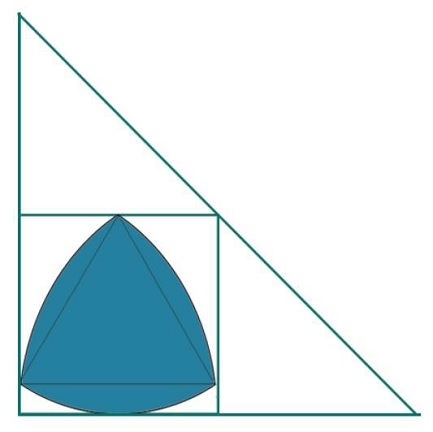
우리는 높이 l, 밑변 b인 직각삼각형에 내접하는 정사각형의 한 변의 길이가 -
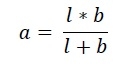
Reuleaux 삼각형의 높이는 a와 같다는 것을 알고 있습니다. 따라서 a = x입니다. 따라서 Reuleaux 삼각형의 넓이는 -
Example
#include <iostream>
#include <cmath>
using namespace std;
float areaReuleaux(float l, float b) { //l and b are height and base of right angled triangle
if (l < 0 || b < 0) //either l or b is negative it is invalid
return -1;
float a = (l*b)/(l+b);
float area = ((3.1415 - sqrt(3)) * (a) * (a))/2;
return area;
}
int main() {
float l = 5;
float b = 12;
cout << "Area of Reuleaux Triangle: " << areaReuleaux(l, b);
}Output
Area of Reuleaux Triangle: 8.77858
위 내용은 직각삼각형인 정사각형에 새겨진 가장 큰 루에로삼각형은 무엇입니까?의 상세 내용입니다. 자세한 내용은 PHP 중국어 웹사이트의 기타 관련 기사를 참조하세요!

핫 AI 도구

Undresser.AI Undress
사실적인 누드 사진을 만들기 위한 AI 기반 앱

AI Clothes Remover
사진에서 옷을 제거하는 온라인 AI 도구입니다.

Undress AI Tool
무료로 이미지를 벗다

Clothoff.io
AI 옷 제거제

AI Hentai Generator
AI Hentai를 무료로 생성하십시오.

인기 기사

뜨거운 도구

메모장++7.3.1
사용하기 쉬운 무료 코드 편집기

SublimeText3 중국어 버전
중국어 버전, 사용하기 매우 쉽습니다.

스튜디오 13.0.1 보내기
강력한 PHP 통합 개발 환경

드림위버 CS6
시각적 웹 개발 도구

SublimeText3 Mac 버전
신 수준의 코드 편집 소프트웨어(SublimeText3)

뜨거운 주제
 7518
7518
 15
15
 1378
1378
 52
52
 81
81
 11
11
 53
53
 19
19
 21
21
 68
68
 Java에서 사각형에 새겨진 원의 면적 찾기
Aug 19, 2023 pm 04:53 PM
Java에서 사각형에 새겨진 원의 면적 찾기
Aug 19, 2023 pm 04:53 PM
원은 모서리가 없는 2차원 다이어그램 주위에 있습니다. 모든 원은 원점이 있고 원 위의 모든 점은 원점으로부터 동일한 거리를 유지합니다. 원점과 원점 사이의 거리는 원의 반경으로 알려져 있습니다.
 정삼각형에 내접할 수 있는 가장 큰 정사각형은 무엇입니까?
Sep 22, 2023 pm 06:21 PM
정삼각형에 내접할 수 있는 가장 큰 정사각형은 무엇입니까?
Sep 22, 2023 pm 06:21 PM
여기서 우리는 정삼각형에 내접할 수 있는 가장 큰 정사각형의 면적을 볼 수 있습니다. 삼각형의 변의 길이는 'a'이고 정사각형의 변의 길이는 x입니다. 삼각형의 변 길이 'a'는 − 따라서 x는 −입니다. 예 #include<iostream>#include<cmath>usingnamespacestd;floatareaSquare(floata){//aissideoftriangle if(a<0)//ifaisnegative,thenthisisi
 C 프로그램에서 다음을 중국어로 번역합니다. 정삼각형에 내접한 원에 내접한 정사각형의 면적은 얼마입니까?
Aug 31, 2023 pm 12:25 PM
C 프로그램에서 다음을 중국어로 번역합니다. 정삼각형에 내접한 원에 내접한 정사각형의 면적은 얼마입니까?
Aug 31, 2023 pm 12:25 PM
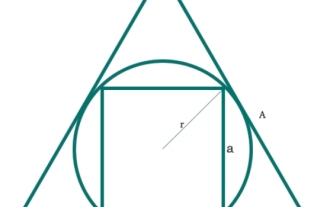
여기서 우리는 정사각형의 면적이 원에 내접되어 있고 원이 정삼각형에 내접되어 있음을 볼 수 있습니다. 정사각형의 변은 "a"입니다. 원의 반지름은 "r"이고 육각형의 변은 "A"입니다. 차트는 아래와 같습니다. 따라서 정사각형의 대각선은 - 정사각형의 면적은 - 예 #include<iostream>#include<cmath>usingnamespacestd;floatarea(floatA){//Aisthesideofthetriangle if(A<0)//ifthevalueisne
 직각삼각형인 정사각형에 새겨진 가장 큰 루에로삼각형은 무엇입니까?
Sep 04, 2023 pm 10:29 PM
직각삼각형인 정사각형에 새겨진 가장 큰 루에로삼각형은 무엇입니까?
Sep 04, 2023 pm 10:29 PM
여기서 우리는 정사각형에 내접된 가장 큰 Reuleaux 삼각형의 면적을 볼 수 있으며, 이는 다시 직각 삼각형에 내접됩니다. 정사각형의 한 변의 길이는 'a'입니다. Reuleaux 삼각형의 높이는 x입니다. 삼각형의 밑변은 b, 높이는 l, 빗변은 h입니다. 우리는 높이가 l이고 밑변이 b인 직각삼각형에 내접하는 정사각형이 한 변의 길이를 갖는다는 것을 알고 있습니다. 릴로삼각형의 높이는 a와 같습니다. 따라서 a=x입니다. 따라서 Reuleaux 삼각형의 면적은 - 예#include<iostream>#include<cmath>usingnamespacestd;fl
 육각형에 새겨진 원 안의 정사각형의 면적을 계산하는 C 프로그램
Aug 28, 2023 pm 08:41 PM
육각형에 새겨진 원 안의 정사각형의 면적을 계산하는 C 프로그램
Aug 28, 2023 pm 08:41 PM
정육각형이 내접한 원에 내접한 정사각형이 있을 때 정사각형의 넓이를 구해야 하고, 이를 위해서는 정사각형의 한 변의 길이와 정육각형의 한 변의 길이 사이의 관계를 찾아야 합니다. . 정육각형의 내접원 반지름의 수학식은 r=A√3/2입니다. 정사각형의 대각선은 원의 지름과 같으므로 반지름과 변의 길이 사이의 관계는 다음과 같습니다. 정육각형에 따른 a=√r 의 변의 길이, a=√3A/√2, 즉 정사각형의 면적,area=a2=(√3A/√2)2 예시 #include<stdio. h>#포함
 C에서는 정사각형 내부의 가장 큰 Reuleaux 삼각형입니다.
Sep 07, 2023 pm 12:29 PM
C에서는 정사각형 내부의 가장 큰 Reuleaux 삼각형입니다.
Sep 07, 2023 pm 12:29 PM
룰 삼각형은 세 개의 디스크가 교차하여 형성된 모양으로, 각 디스크의 중심은 다른 두 디스크의 경계에 있습니다. 그 경계는 일정한 너비의 곡선으로, 원 자체를 제외하면 가장 단순하고 잘 알려진 곡선이다. 폭이 일정하다는 것은 두 개의 평행한 지지선 사이의 간격이 방향에 관계없이 동일하다는 것을 의미합니다. 직경이 모두 동일하기 때문입니다. Lule 삼각형의 경계는 정삼각형을 기반으로 하는 일정한 너비의 곡선입니다. 모서리의 모든 점은 반대쪽 꼭지점에서 등거리에 있습니다. Lule 삼각형을 만드는 공식은 곡선이 정삼각형을 기반으로 하고 삼각형의 변의 길이가 h인 경우 Lule 삼각형의 면적은 A=(π*h2)/2–2*(입니다. 아리아오
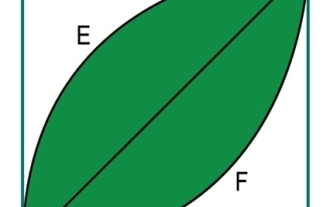 정사각형 안에 나뭇잎의 면적은 얼마입니까?
Aug 26, 2023 pm 05:13 PM
정사각형 안에 나뭇잎의 면적은 얼마입니까?
Aug 26, 2023 pm 05:13 PM
여기에서는 정사각형 ABCD 내에 있는 나뭇잎 모양의 물체의 면적을 계산하는 방법을 살펴보겠습니다. 정사각형의 각 변의 길이는 'a'입니다. 잎에는 두 개의 동일한 부분이 있습니다. 각 부분의 면적은 p이고, 이제 - 전체 잎의 면적은 2p입니다. 예 #include<iostream>usingnamespacestd;floatleafArea(floata){ return(a*a*(3.1415/2-1));}intmain(){ floatsquare
 C 프로그램에서 주어진 크기의 최대 제곱합 부분행렬을 인쇄합니다.
Sep 07, 2023 pm 01:53 PM
C 프로그램에서 주어진 크기의 최대 제곱합 부분행렬을 인쇄합니다.
Sep 07, 2023 pm 01:53 PM
NxN 행렬이 주어지면 행렬 MxM의 모든 요소의 합이 최대가 되는 M=1인 MxM 부분 행렬을 찾습니다. 행렬 NxN에 대한 입력에는 0, 양의 정수 및 음의 정수 값이 포함될 수 있습니다. 입력 예: {{1,1,1,1,1}, {2,2,2,2,2}, {3,3,3,3,3}, {4,4,4,4,4 }, {5,5,5,5,5}}출력:&




