AA樹在C/C++中是什麼?
在電腦科學中,AA樹被定義為一種用於高效地儲存和檢索有序資料的平衡樹實作。 AA樹被視為紅黑樹的變體,紅黑樹是一種支援高效添加和刪除條目的二元搜尋樹。與紅黑樹不同,AA樹上的紅色節點只能作為右子節點添加,不能作為左子節點添加。這個操作的結果是模擬2-3樹而不是2-3-4樹,從而簡化了維護操作。紅黑樹的維護演算法需要假設或考慮七種不同的形狀來正確平衡樹。

與紅黑樹相反,AA樹只需要假設或考慮兩個形狀,因為只有右連結可以是紅色。
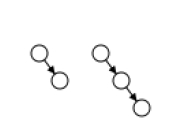
平衡旋轉
#紅黑樹每個節點需要一個平衡元資料位元(顏色) ,而AA樹每個節點需要O(log(log(N)))個元資料位,以整數「level」的形式。以下不變式適用於AA樹:
每個葉節點的層級被視為1。
每個左子節點的層級比其父節點小1。
每個右子節點的層級等於或比其父節點小1。
每個右孫子節點的等級嚴格小於其祖父節點。
等級大於1的每個節點都有兩個子節點。
重新平衡AA樹比重新平衡紅黑樹要簡單得多。
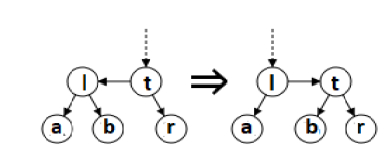
在AA樹中,只需要兩個不同的操作來恢復平衡:「skew」和「split」。 Skew被視為右旋,用右水平連結取代一個由左水平連結組成的子樹。在Split的情況下,是左旋和等級增加,用兩個或更多連續的右水平連結替換一個包含兩個較少連續的右水平連結的子樹。下面解釋了“skew”和“split”這兩個操作。
函數skew的定義如下:
input: An AA tree that needs to be rebalanced is represented by a node, t. output: The rebalanced AA tree is represented by another node. if nil(t) then return nil else if nil(left(t)) then return t else if level(left(t)) == level(t) then Exchange the pointers of horizontal left links. l = left(t) left(t) := right(l) right(l) := t return l else return t end if end function
#function split is
的翻譯為:
函數split是
input: An AA tree that needs to be rebalanced is represented by a node, t.
output: The rebalanced AA tree is represented by another node.
if nil(t) then
return nil
else if nil(right(t)) or nil(right(right(t))) then
return t
else if level(t) == level(right(right(t))) then
We have two horizontal right links. The middle node is taken, elevate it, and return it.
r = right(t)
right(t) := left(r)
left(r) := t
level(r) := level(r) + 1
return r
else
return t
end if
end function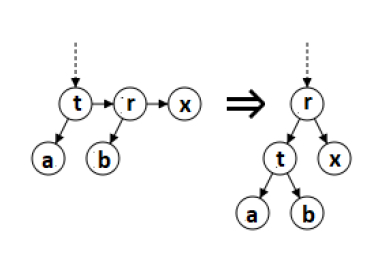
分割-
以上是AA樹在C/C++中是什麼?的詳細內容。更多資訊請關注PHP中文網其他相關文章!

熱AI工具

Undresser.AI Undress
人工智慧驅動的應用程序,用於創建逼真的裸體照片

AI Clothes Remover
用於從照片中去除衣服的線上人工智慧工具。

Undress AI Tool
免費脫衣圖片

Clothoff.io
AI脫衣器

AI Hentai Generator
免費產生 AI 無盡。

熱門文章

熱工具

記事本++7.3.1
好用且免費的程式碼編輯器

SublimeText3漢化版
中文版,非常好用

禪工作室 13.0.1
強大的PHP整合開發環境

Dreamweaver CS6
視覺化網頁開發工具

SublimeText3 Mac版
神級程式碼編輯軟體(SublimeText3)

熱門話題
 C語言數據結構:樹和圖的數據表示與操作
Apr 04, 2025 am 11:18 AM
C語言數據結構:樹和圖的數據表示與操作
Apr 04, 2025 am 11:18 AM
C語言數據結構:樹和圖的數據表示與操作樹是一個層次結構的數據結構由節點組成,每個節點包含一個數據元素和指向其子節點的指針二叉樹是一種特殊類型的樹,其中每個節點最多有兩個子節點數據表示structTreeNode{intdata;structTreeNode*left;structTreeNode*right;};操作創建樹遍歷樹(先序、中序、後序)搜索樹插入節點刪除節點圖是一個集合的數據結構,其中的元素是頂點,它們通過邊連接在一起邊可以是帶權或無權的數據表示鄰
 C語言文件操作難題的幕後真相
Apr 04, 2025 am 11:24 AM
C語言文件操作難題的幕後真相
Apr 04, 2025 am 11:24 AM
文件操作難題的真相:文件打開失敗:權限不足、路徑錯誤、文件被佔用。數據寫入失敗:緩衝區已滿、文件不可寫、磁盤空間不足。其他常見問題:文件遍歷緩慢、文本文件編碼不正確、二進製文件讀取錯誤。
 在C中如何有效地使用RVALUE參考?
Mar 18, 2025 pm 03:29 PM
在C中如何有效地使用RVALUE參考?
Mar 18, 2025 pm 03:29 PM
文章討論了在C中有效使用RVALUE參考,以進行移動語義,完美的轉發和資源管理,重點介紹最佳實踐和性能改進。(159個字符)
 如何在C 20中使用範圍進行更有表現的數據操縱?
Mar 17, 2025 pm 12:58 PM
如何在C 20中使用範圍進行更有表現的數據操縱?
Mar 17, 2025 pm 12:58 PM
C 20範圍通過表現力,合成性和效率增強數據操作。它們簡化了複雜的轉換並集成到現有代碼庫中,以提高性能和可維護性。
 c語言函數的基本要求有哪些
Apr 03, 2025 pm 10:06 PM
c語言函數的基本要求有哪些
Apr 03, 2025 pm 10:06 PM
C語言函數是代碼模塊化和程序搭建的基礎。它們由聲明(函數頭)和定義(函數體)組成。 C語言默認使用值傳遞參數,但也可使用地址傳遞修改外部變量。函數可以有返回值或無返回值,返回值類型必須與聲明一致。函數命名應清晰易懂,使用駝峰或下劃線命名法。遵循單一職責原則,保持函數簡潔性,以提高可維護性和可讀性。
 如何使用C中的移動語義來提高性能?
Mar 18, 2025 pm 03:27 PM
如何使用C中的移動語義來提高性能?
Mar 18, 2025 pm 03:27 PM
本文討論了使用C中的移動語義來通過避免不必要的複制來提高性能。它涵蓋了使用std :: Move的實施移動構造函數和任務運算符,並確定了關鍵方案和陷阱以有效
 動態調度如何在C中起作用,如何影響性能?
Mar 17, 2025 pm 01:08 PM
動態調度如何在C中起作用,如何影響性能?
Mar 17, 2025 pm 01:08 PM
本文討論了C中的動態調度,其性能成本和優化策略。它突出了動態調度會影響性能並將其與靜態調度進行比較的場景,強調性能和之間的權衡







