Rabin-Karp演算法的C程式用於模式搜尋

C 中的模式匹配- 我們必須查找一個字串是否存在於另一個字串中,例如,字串「algorithm」存在於字串“naive algorithm”中。如果是找到,然後顯示它的位置(即它的位置)。我們傾向於創建一個接收 2 個字元數組的函數,如果匹配則返回位置 否則返回-1。
Input: txt = "HERE IS A NICE CAP" pattern = "NICE" Output: Pattern found at index 10 Input: txt = "XYZXACAADXYZXYZX" pattern = "XYZX" Output: Pattern found at index 0 Pattern found at index 9 Pattern found at index 12
Rabin-Karp 是另一種模式搜尋演算法。就是字串匹配 Rabin 和 Karp 提出的演算法,用於更有效地尋找模式 方式。與樸素演算法一樣,它也透過移動視窗來檢查模式 它會一一查找哈希值,但無需檢查所有情況下的所有字元。當雜湊值匹配時,才繼續檢查每個字元。這樣,每個文字子序列只有一次比較,使其成為更有效的模式搜尋演算法。
預處理時間- O(m)
Rabin-Karp演算法的時間複雜度為O(m n),但對於最壞的情況, 它是O(mn)。
演算法
rabinkarp_algo(text,pattern,prime)
輸入 rabinkarp_algo(text,pattern,prime) #輸入 strong>− 正文和模式。尋找另一個雜湊位置的質數 輸出− 找到模式的位置 即時示範 以上是Rabin-Karp演算法的C程式用於模式搜尋的詳細內容。更多資訊請關注PHP中文網其他相關文章!Start
pat_len := pattern Length
str_len := string Length
patHash := 0 and strHash := 0, h := 1
maxChar := total number of characters in character set
for index i of all character in the pattern, do
h := (h*maxChar) mod prime
for all character index i of pattern, do
patHash := (maxChar*patHash + pattern[i]) mod prime
strHash := (maxChar*strHash + text[i]) mod prime
for i := 0 to (str_len - pat_len), do
if patHash = strHash, then
for charIndex := 0 to pat_len -1, do
if text[i+charIndex] ≠ pattern[charIndex], then
break
if charIndex = pat_len, then
print the location i as pattern found at i position.
if i < (str_len - pat_len), then
strHash := (maxChar*(strHash – text[i]*h)+text[i+patLen]) mod prime, then
if strHash < 0, then
strHash := strHash + prime
End範例
#include<stdio.h>
#include<string.h>
int main (){
char txt[80], pat[80];
int q;
printf ("Enter the container string </p><p>");
scanf ("%s", &txt);
printf ("Enter the pattern to be searched </p><p>");
scanf ("%s", &pat);
int d = 256;
printf ("Enter a prime number </p><p>");
scanf ("%d", &q);
int M = strlen (pat);
int N = strlen (txt);
int i, j;
int p = 0;
int t = 0;
int h = 1;
for (i = 0; i < M - 1; i++)
h = (h * d) % q;
for (i = 0; i < M; i++){
p = (d * p + pat[i]) % q;
t = (d * t + txt[i]) % q;
}
for (i = 0; i <= N - M; i++){
if (p == t){
for (j = 0; j < M; j++){
if (txt[i + j] != pat[j])
break;
}
if (j == M)
printf ("Pattern found at index %d </p><p>", i);
}
if (i < N - M){
t = (d * (t - txt[i] * h) + txt[i + M]) % q;
if (t < 0)
t = (t + q);
}
}
return 0;
}輸出
Enter the container string
tutorialspointisthebestprogrammingwebsite
Enter the pattern to be searched
p
Enter a prime number
3
Pattern found at index 8
Pattern found at index 21

熱AI工具

Undresser.AI Undress
人工智慧驅動的應用程序,用於創建逼真的裸體照片

AI Clothes Remover
用於從照片中去除衣服的線上人工智慧工具。

Undress AI Tool
免費脫衣圖片

Clothoff.io
AI脫衣器

AI Hentai Generator
免費產生 AI 無盡。

熱門文章

熱工具

記事本++7.3.1
好用且免費的程式碼編輯器

SublimeText3漢化版
中文版,非常好用

禪工作室 13.0.1
強大的PHP整合開發環境

Dreamweaver CS6
視覺化網頁開發工具

SublimeText3 Mac版
神級程式碼編輯軟體(SublimeText3)

熱門話題
 將以下內容翻譯為中文:C程式將羅馬數字轉換為十進位數字
Sep 05, 2023 pm 09:53 PM
將以下內容翻譯為中文:C程式將羅馬數字轉換為十進位數字
Sep 05, 2023 pm 09:53 PM
給出以下是一個將羅馬數字轉換為十進制數字的C語言演算法:演算法步驟1-開始步驟2-在運行時讀取羅馬數字步驟3-長度:=strlen(roman)步驟4-對於i=0到長度-1 步驟4.1-switch(roman[i]) 步驟4.1.1-case'm': &nbs
 C++程式比較兩個字串的字典序
Sep 04, 2023 pm 05:13 PM
C++程式比較兩個字串的字典序
Sep 04, 2023 pm 05:13 PM
字典序字串比較是指字串依照字典順序進行比較。例如,如果有兩個字串'apple'和'appeal',第一個字串將排在後面,因為前三個字元'app'是相同的。然後對於第一個字串,字元是'l',而在第二個字串中,第四個字元是'e'。由於'e'比'l'短,所以如果我們按照字典順序排列,它將排在前面。在安排之前,字串會按字典順序進行比較。在本文中,我們將看到使用C++進行按字典順序比較兩個字串的不同技術。在C++字串中使用compare()函數C++string物件有一個compare()
 C++程式以給定值為參數,找出雙曲正弦反函數的值
Sep 17, 2023 am 10:49 AM
C++程式以給定值為參數,找出雙曲正弦反函數的值
Sep 17, 2023 am 10:49 AM
雙曲函數是使用雙曲線而不是圓定義的,與普通三角函數相當。它從提供的弧度角傳回雙曲正弦函數中的比率參數。但要做相反的事,或者換句話說。如果我們想要根據雙曲正弦值計算角度,我們需要像雙曲反正弦運算一樣的反雙曲三角運算。本課程將示範如何使用C++中的雙曲反正弦(asinh)函數,並使用雙曲正弦值(以弧度為單位)計算角度。雙曲反正弦運算遵循下列公式-$$\mathrm{sinh^{-1}x\:=\:In(x\:+\:\sqrt{x^2\:+\:1})},其中\:In\:是\:自然對數\:(log_e\:k)
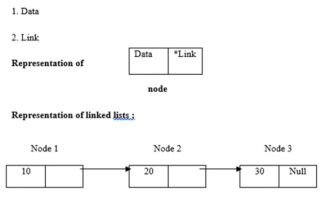 C程式以找到鍊錶的長度
Sep 07, 2023 pm 07:33 PM
C程式以找到鍊錶的長度
Sep 07, 2023 pm 07:33 PM
連結列表使用動態記憶體分配,即它們相應地增長和收縮。它們被定義為節點的集合。這裡,節點有兩個部分,即資料和鏈路。資料、連結和鍊錶的表示如下-鍊錶的類型鍊錶有四種類型,如下:-單鍊錶/單鍊錶雙/雙向鍊錶循環單鍊錶循環雙鍊錶我們使用遞歸方法求鍊錶長度的邏輯是-intlength(node *temp){ if(temp==NULL) returnl; else{&n
 C程式使用rename()函數更改檔名
Sep 21, 2023 pm 10:01 PM
C程式使用rename()函數更改檔名
Sep 21, 2023 pm 10:01 PM
rename函數將檔案或目錄從舊名稱變更為新名稱。此操作類似於移動操作。因此,我們也可以使用此rename函數來移動檔案。此函數存在於stdio.h庫頭檔中。 rename函數的語法如下:intrename(constchar*oldname,constchar*newname);rename()函數的函數它接受兩個參數。一個是oldname,一個是newname。這兩個參數都是指向常數字元的指針,用於定義檔案的舊名稱和新名稱。如果檔案重新命名成功,則傳回零;否則,傳回非零整數。在重新命名操作期間
 C++程式列印字典
Sep 11, 2023 am 10:33 AM
C++程式列印字典
Sep 11, 2023 am 10:33 AM
映射是C++中的一種特殊類型的容器,其中每個元素都是一對兩個值,即鍵值和映射值。鍵值用於索引每個項目,映射值是與鍵關聯的值。無論映射值是否唯一,鍵始終是唯一的。要在C++中列印映射元素,我們必須使用迭代器。一組項目中的一個元素由迭代器物件指示。迭代器主要與陣列和其他類型的容器(例如向量)一起使用,並且它們具有一組特定的操作,可用於識別特定範圍內的特定元素。可以增加或減少迭代器來引用範圍或容器中存在的不同元素。迭代器指向範圍內特定元素的記憶體位置。使用迭代器在C++中列印地圖首先,我們先來看看如何定義
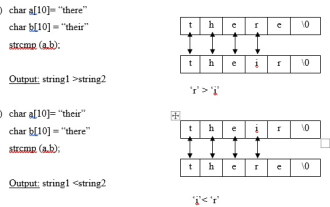 寫一個C程序,使用strncmp函式庫函數來比較兩個字串
Sep 09, 2023 pm 01:17 PM
寫一個C程序,使用strncmp函式庫函數來比較兩個字串
Sep 09, 2023 pm 01:17 PM
Strncmp是一個預先定義的函式庫函數,存在於string.h檔案中,它用於比較兩個字串並顯示哪個字串較大。 strcmp函數(字串比較)此函數比較兩個字串。它會傳回兩個字串中第一個不匹配字元的ASCII差異。語法intstrcmp(string1,string2);如果差異等於零,則string1=string2。如果差異為正,則string1>string2。如果差異為負,則string1<string2。範例strncmp函數此函數用於比較兩個字串的前n個字元。語法strn
 C++程式來檢查一個字元是否為字母或非字母
Sep 14, 2023 pm 03:37 PM
C++程式來檢查一個字元是否為字母或非字母
Sep 14, 2023 pm 03:37 PM
在解決一些邏輯程式設計問題時,使用字串或字元有時非常有用。字串是字元的集合,字元是1位元組資料類型,用於保存ASCII值中的符號。符號可以是英文字母、數字或特殊字元。在本文中,我們將學習如何使用C++檢查一個字元是否是英文字母或字母表中的字母。檢查isalpha()函數要檢查數字是否為字母,我們可以使用ctype.h頭檔中的isalpha()函數。這將一個字元作為輸入,如果是字母表,則傳回true,否則傳回false。讓我們看看下面的C++實作來了解這個函數的用法。例子的中文翻譯為:示






